Institute of Engineering and Economics, Henan Institute of Economics and Trade, Zhengzhou, Henan, 450018, China
Received:
June 28, 2022
Accepted:
August 5, 2022
Publication Date:
October 14, 2022
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
Download Citation: ||https://doi.org/10.6180/jase.202307_26(7).0009
ABSTRACT
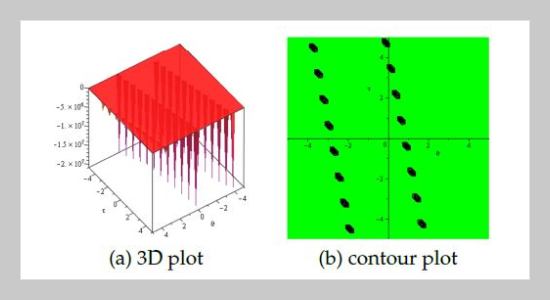
The generalized algebra technique is used to search for exact wave solutions of the ZK-BBM equation in this article. This equation is a notable model for describing the acoustic waves in harmonic crystals, shallow water waves etc. By applying the wave transformation, we obtained an ordinary differential equation. We have successfully obtained many exact wave solutions with arbitrary parameters by the method that exact wave solutions are expressed in terms of generalized hyperbolic function solution, generalized trigonometric function solution, exponential function solution, and rational function solution. The results show that the generalized algebra technique is a very concise and powerful mathematical tool for nonlinear evolution equations in engineering and science.
Keywords:
ZK-BBM equation; generalized algebra technique; exact wave solution
REFERENCES
- [1] R. Cimpoiasu, (2020) “Multiple invariant solutions of the 3 D potential Yu–Toda–Sasa–Fukuyama equation via symmetry technique" International Journal of Modern Physics B 34(20): 2050188. DOI: 10.1142/S021797922050188X.
- [2] R. Cimpoiasu, (2018) “Travelling Wave Solutions For The Long-Short Wave Resonance Model Through An Improved" Romanian Journal of Physics 63: 111.
- [3] R. Cimpoiasu and A. S. Pauna, (2018) “Complementary wave solutions for the long-short wave resonance model via the extended trial equation method and the generalized Kudryashov method" Open Physics 16(1): 419–426. DOI: 10.1515/phys-2018-0057.
- [4] A. Houwe, S. Abbagari, Y. Salathiel, M. Inc, S. Y. Doka, K. T. Crepin, and D. Baleanu, (2020) “Complex traveling-wave and solitons solutions to the Klein-Gordon-Zakharov equations" Results in Physics 17: 103127. DOI: 10.1016/j.rinp.2020.103127.
- [5] A. Houwe, M. ˙Inç, S. Doka, M. Akinlar, and D. Baleanu, (2020) “Chirped solitons in negative index materials generated by Kerr nonlinearity" Results in Physics 17: 103097. DOI: 10.1016/j.rinp.2020.103097.
- [6] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear engineering 4(1): 15–22. DOI: 10.1515/nleng-2014-0018.
- [7] H. Aminikhah, A. H. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da sociedade paranaense de matemática 34(2): 213–229. DOI: 10.5269/bspm.v34i2.25501.
- [8] B. Ghanbari, K. S. Nisar, and M. Aldhaifallah, (2020) “Abundant solitary wave solutions to an extended nonlinear Schrodinger’s equation with conformable derivative using an efficient integration method" Advances in Difference Equations 2020(1): 1–25. DOI: 10.1186/s13662-020-02787-7.
- [9] K. Hosseini, M. Matinfar, and M. Mirzazadeh, (2021) “Soliton solutions of high-order nonlinear Schrodinger equations with different laws of nonlinearities" Regular and Chaotic Dynamics 26(1): 105–112. DOI: 10.1134/S1560354721010068.
- [10] H. M. Baskonus, H. Bulut, and T. A. Sulaiman, (2019) “New complex hyperbolic structures to the lonngren-wave equation by using sine-gordon expansion method" Applied Mathematics and Nonlinear Sciences 4(1): 129–138.
- [11] A. Kurt, M. ¸Senol, O. Tasbozan, and M. Chand, (2019) “Two reliable methods for the solution of fractional coupled Burgers’ equation arising as a model of Polydispersive sedimentation" Applied Mathematics and Nonlinear Sciences 4(2): 523–534.
- [12] E. M. Zayed and A.-G. Al-Nowehy, (2020) “New generalized ϕ6-model expansion method and its applications to the (3+ 1) dimensional resonant nonlinear Schrödinger equation with parabolic law nonlinearity" Optik 214: 164702.
- [13] E. M. Zayed, T. A. Nofal, A. Al-Nowehy, and M. E. Alngar, (2021) “Optical solitons and other solutions to the (2+ 1)-dimensional coupled system of NLSE by two integration approaches" Optik 232: 166510.
- [14] M. Ramzan, Y.-M. Chu, H. ur Rehman, M. S. Saleem, and C. Park, (2021) “Soliton Solutions for Anti-Cubic Nonlinearity Using Three Analytical Approaches" Journal of Applied Analysis & Computation 11(4): 2177–2192. DOI: 10.11948/20200380.
- [15] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable timefractional derivative" Calcolo 53(3): 475–485. DOI: 10.1007/s10092-015-0158-8.
- [16] V. Kumar and A. Patel, (2020) “Construction of the soliton solutions and modulation instability analysis for the Mel’nikov system" Chaos, Solitons & Fractals 140: 110159. DOI: 10.1016/j.chaos.2020.110159.
- [17] V. Kumar and A. Patel, (2019) “Soliton solutions and modulation instability analysis of the coupled Zakharov-Kuznetsov equation" The European Physical Journal Plus 134(4): 170. DOI: 10.1140/epjp/i2019-12521-6.
- [18] V. Kumar and A. Patel, (2021) “Dispersion and phase managed optical soliton solutions of a nonautonomous (3+1)-dimensional coupled nonlinear Schrodinger equation" Optik 242: 166648. DOI: 10.1016/j.ijleo.2021.166648.
- [19] S. Kumar, M. Niwas, and N. Mann, (2021) “Abundant analytical closed-form solutions and various solitonic wave forms to the ZK-BBM and GZK-BBM equations in fluids and plasma physics" Partial Differential Equations in Applied Mathematics 4: 100200. DOI: 10.1016/j.padiff.2021.100200.
- [20] S. Kumar, L. Kaur, and M. Niwas, (2021) “Some exact invariant solutions and dynamical structures of multiple solitons for the (2+ 1)-dimensional Bogoyavlensky-Konopelchenko equation with variable coefficients using Lie symmetry analysis" Chinese Journal of Physics 71: 518–538. DOI: 10.1016/j.cjph.2021.03.021.
- [21] S. Kumar, M. Niwas, and S. K. Dhiman, (2021) “Abundant analytical soliton solutions and different wave profiles to the Kudryashov-Sinelshchikov equation in mathematical physics" Journal of Ocean Engineering and Science: DOI: 10.1016/j.joes.2021.10.009.
- [22] S. Kumar, M. Niwas, M. Osman, and M. Abdou, (2021) “Abundant different types of exact soliton solution to the (4+ 1)-dimensional Fokas and (2+ 1)-dimensional breaking soliton equations" Communications in Theoretical Physics 73(10): 105007. DOI: 10.1088/1572-9494/ac11ee.
- [23] S. Kumar, M. Niwas, and A.-M. Wazwaz, (2020) “Lie symmetry analysis, exact analytical solutions and dynamics of solitons for (2+ 1)-dimensional NNV equations" Physica Scripta 95(9): 095204. DOI: 10.1088/1402-4896/aba5ae.
- [24] S. El-Ganaini, S. Kumar, and M. Niwas, (2022) “Construction of multiple new analytical soliton solutions and various dynamical behaviors to the nonlinear convectiondiffusion-reaction equation with power-law nonlinearity and density-dependent diffusion via Lie symmetry approach together with a couple of integration approaches" Journal of Ocean Engineering and Science: DOI: 10.1016/j.joes.2022.01.006.
- [25] B. Gao and Y. Wang, (2021) “Traveling wave solutions for the (2+ 1)-dimensional generalized Zakharov–Kuznetsov equation with variable coefficients" Optical and Quantum Electronics 53(1): 1–15.
- [26] S. Kumar, M. Niwas, and I. Hamid, (2021) “Lie symmetry analysis for obtaining exact soliton solutions of generalized Camassa–Holm–Kadomtsev–Petviashvili equation" International Journal of Modern Physics B 35(02): 2150028. DOI: 10.1142/S0217979221500284.
- [27] M. S. Osman and A.-M. Wazwaz, (2018) “An efficient algorithm to construct multi-soliton rational solutions of the (2+ 1)-dimensional KdV equation with variable coefficients" Applied mathematics and Computation 321: 282–289. DOI: 10.1016/j.amc.2017.10.042.
- [28] B. Ghanbari, M. Osman, and D. Baleanu, (2019) “Generalized exponential rational function method for extended Zakharov–Kuzetsov equation with conformable derivative" Modern Physics Letters A 34(20): 1950155. DOI: 10.1142/S0217732319501554.
- [29] M. Osman and B. Ghanbari, (2018) “New optical solitary wave solutions of Fokas-Lenells equation in presence of perturbation terms by a novel approach" Optik 175: 328–333. DOI: 10.1016/j.ijleo.2018.08.007.
- [30] K. K. Ali, M. S. Osman, H. M. Baskonus, N. S. Elazabb, and E. ˙Ilhan, (2020) “Analytical and numerical study of the HIV-1 infection of CD4+ T-cells conformable fractional mathematical model that causes acquired immunodeficiency syndrome with the effect of antiviral drug therapy" Mathematical Methods in the Applied Sciences: DOI: 10.1002/mma.7022.
- [31] K. K. Ali, A.-M. Wazwaz, and M. Osman, (2020) “Optical soliton solutions to the generalized nonautonomous nonlinear Schrödinger equations in optical fibers via the sine-Gordon expansion method" Optik 208: 164132. DOI:10.1016/j.ijleo.2019.164132.
- [32] J.-G. Liu,W.-H. Zhu, M. Osman, andW.-X. Ma, (2020) “An explicit plethora of different classes of interactive lump solutions for an extension form of 3D-Jimbo–Miwa model" The European Physical Journal Plus 135(5): 1–9. DOI: 10.1140/epjp/s13360-020-00405-9.
- [33] B. Inan, M. S. Osman, T. Ak, and D. Baleanu, (2020) “Analytical and numerical solutions of mathematical biology models: The Newell-Whitehead-Segel and Allen-Cahn equations" Mathematical methods in the applied sciences 43(5): 2588–2600. DOI: 10.1002/mma.6067.
- [34] K. S. Nisar, A. Ciancio, K. K. Ali, M. Osman, C. Cattani, D. Baleanu, A. Zafar, M. Raheel, and M. Azeem, (2022) “On beta-time fractional biological population model with abundant solitary wave structures" Alexandria Engineering Journal 61(3): 1996–2008. DOI: 10.1016/j.aej.2021.06.106.
- [35] M. A. Akbar, M. A. Kayum, and M. Osman, (2021) “Bright, periodic, compacton and bell-shape soliton solutions of the extended QZK and (3+ 1)-dimensional ZK equations" Communications in Theoretical Physics 73(10): 105003. DOI: 10.1088/1572-9494/ac1a6c.
- [36] N. H. Aljahdaly, R. G. ALoufi, and A. R. Seadawy, (2021) “Stability analysis and soliton solutions for the longitudinal wave equation in magneto electro-elastic circular rod" Results in Physics 26: 104329. DOI: 10.1016/j.rinp.2021.104329.
- [37] H. K. Barman, M. A. Akbar, M. Osman, K. S. Nisar, M. Zakarya, A.-H. Abdel-Aty, and H. Eleuch, (2021) “Solutions to the Konopelchenko-Dubrovsky equation and the Landau-Ginzburg-Higgs equation via the generalized Kudryashov technique" Results in Physics 24: 104092. DOI: 10.1016/j.rinp.2021.104092.
- [38] J.-G. Liu, M. S. Osman, W.-H. Zhu, L. Zhou, and D. Baleanu, (2020) “The general bilinear techniques for studying the propagation of mixed-type periodic and lumptype solutions in a homogenous-dispersive medium" AIP Advances 10(10): 105325. DOI: 10.1063/5.0019219.
- [39] H. Almusawa, K. K. Ali, A.-M.Wazwaz, M. Mehanna, D. Baleanu, and M. Osman, (2021) “Protracted study on a real physical phenomenon generated by media inhomogeneities" Results in Physics 31: 104933. DOI: 10.1016/j.rinp.2021.104933.
- [40] L. Akinyemi, (2021) “Two improved techniques for the perturbed nonlinear Biswas–Milovic equation and its optical solitons" Optik 243: 167477. DOI: 10.1016/j.ijleo.2021.167477.
- [41] M. Senol, L. Akinyemi, A. Ata, and O. S. Iyiola, (2021) “Approximate and generalized solutions of conformable type Coudrey–Dodd–Gibbon–Sawada–Kotera equation" International Journal of Modern Physics B 35(02): 2150021. DOI: 10.1142/S0217979221500211.
- [42] L. Akinyemi, M. ¸Senol, M. Mirzazadeh, and M. Eslami, (2021) “Optical solitons for weakly nonlocal Schrödinger equation with parabolic law nonlinearity and external potential" Optik 230: 166281. DOI: 10.1016/j.ijleo.2021.166281.
- [43] M. Hashemi, (2017) “A novel simple algorithm for solving the magneto-hemodynamic flow in a semi-porous channel" European Journal of Mechanics-B/Fluids 65: 359–367. DOI: 10.1016/j.euromechflu.2017.05.008.
- [44] R. Najafi, F. Bahrami, and M. Hashemi, (2017) “Classical and nonclassical Lie symmetry analysis to a class of nonlinear time-fractional differential equations" Nonlinear Dynamics 87(3): 1785–1796. DOI: 10.1007/s11071-016-3152-z.
- [45] M. T. Darvishi, M. Najafi, and A.-M. Wazwaz, (2021) “Some optical soliton solutions of space-time conformable fractional Schrödinger-type models" Physica Scripta 96(6): 065213. DOI: 10.1088/1402-4896/abf269.
- [46] M. Khater and B. Ghanbari, (2021) “On the solitary wave solutions and physical characterization of gas diffusion in a homogeneous medium via some efficient techniques" The European Physical Journal Plus 136(4): 1–28. DOI: 10.1140/epjp/s13360-021-01457-1.
- [47] R. M. El-Shiekh and M. Gaballah, (2020) “Bright and dark optical solitons for the generalized variable coefficients nonlinear Schrödinger equation" International Journal of Nonlinear Sciences and Numerical Simulation 21(7-8): 675–681. DOI: 10.1515/ijnsns-2019-0054.
- [48] C. Chen, Y. Jiang, Z.Wang, and J.Wu, (2020) “Dynamical behavior and exact solutions for time-fractional nonlinear Schrödinger equation with parabolic law nonlinearity" Optik 222: 165331. DOI: 10.1016/j.ijleo.2020.165331.
- [49] M. S. Shehata, H. Rezazadeh, E. H. Zahran, E. Tala- Tebue, and A. Bekir, (2019) “New optical soliton solutions of the perturbed Fokas-Lenells equation" Communications in Theoretical Physics 71(11): 1275. DOI: 10.1088/0253-6102/71/11/1275.
- [50] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17):1950196. DOI: 10.1142/S0217984919501963.
- [51] H. Rezazadeh, (2018) “New solitons solutions of the complex Ginzburg-Landau equation with Kerr law nonlinearity" Optik 167: 218–227. DOI: 10.1016/j.ijleo.2018.04.026.
- [52] A.-M.Wazwaz, (2005) “Compact and noncompact physical structures for the ZK–BBM equation" Applied Mathematics and Computation 169(1): 713–725. DOI: 10.1016/j.amc.2004.09.062.
- [53] R. Kumar, M. Kumar, and A. Kumar, (2013) “Some soliton solutions of non linear partial differential equations by Tan-Cot method" IOSR Journal of Mathematics (IOSR-JM) 6(6): 23–28.
- [54] M. Kayum, R. Roy, M. A. Akbar, M. Osman, et al., (2021) “Study of W-shaped, V-shaped, and other type of surfaces of the ZK-BBM and GZD-BBM equations" Optical and Quantum Electronics 53(7): 1–20. DOI: 10.1007/s11082-021-03031-6.
- [55] M. Shakeel and S. T. Mohyud-Din, (2015) “New (G′ /G)-expansion method and its application to the Zakharov-Kuznetsov–Benjamin-Bona-Mahony (ZK–BBM) equation" Journal of the Association of Arab Universities for Basic and Applied Sciences 18: 66–81. DOI: 10.1016/j.jaubas.2014.02.007.
- [56] O. Guner, A. Bekir, L. Moraru, and A. Biswas. “Bright and dark soliton solutions of the generalized Zakharov–Kuznetsov–Benjamin–Bona–Mahony nonlinear evolution equation”. In: Proc. Rom. Acad. Ser. A. 16. 3. 2015, 422–429.
- [57] A. Patel and V. Kumar, (2018) “Dark and kink soliton solutions of the generalized ZK–BBM equation by iterative scheme" Chinese journal of physics 56(3): 819–829. DOI: 10.1016/j.cjph.2018.03.012.