Biao Xu1, Jiangli Wang2 , Fang Yuanlu3
1Shijiazhuang University of Applied Technology, Shijiazhuang 050081, China 2Qinhuangdao Open University, Qinhuangdao 066000, China 3Tianjin Vocational College of Bioengineering, Basic teaching department, Tianjin Kaifaquxiqu 300462, China
Received:
September 21, 2022
Accepted:
February 21, 2023
Publication Date:
June 14, 2023
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
Download Citation: ||https://doi.org/10.6180/jase.202401_27(1).0011
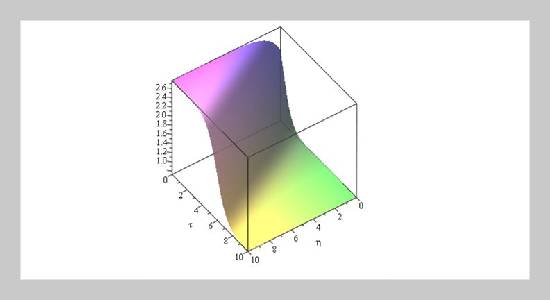
In fact, due to the existence of this category of equations, our understanding of many phenomena around us becomes more complete. In this paper, we study an integrable partial differential equation called the Kadomtsev–Petviashvili equation with a local conformable derivative. This equation is used to describe nonlinear motion. In order to solve the equation, it is first necessary to convert the form of the equation from a
partial derivative to an equation with ordinary derivatives using a suitable variable change. The resulting form will then be the basis of our work to determine the main solutions. All the solutions reported in the paper for the present equation are quite different from the previous findings in other papers. All necessary calculations are provided using symbolic computing software in Maple.
Keywords:
Conformable potential Kadomtsev–Petviashvili equation; new extended direct algebraic method; exact wave solutions
- [1] G.-Q. Xu and Z.-B. Li, (2004) “Symbolic computation of the Painlev ´ e test for nonlinear partial differential equations
using Maple" Computer Physics Communications 161(1-2): 65–75. DOI: 10.1016/j.cpc.2004.04.005. - [2] V. F. Zaitsev and A. D. Polyanin. Handbook of exact solutions for ordinary differential equations. Chapman and Hall/CRC, 2002.
- [3] C. Grossmann, H.-G. Roos, and M. Stynes. Numerical treatment of partial differential equations. 154. Springer, 2007.
- [4] C. Soize and R. Ghanem, (2021) “Probabilistic learning on manifolds constrained by nonlinear partial differential equations for small datasets" Computer Methods in Applied Mechanics and Engineering 380: DOI: 10.1016/j.cma.2021.113777.
- [5] J. Pu, W. Peng, and Y. Chen, (2021) “The datadriven localized wave solutions of the derivative nonlinear Schr¨odinger equation by using improved PINN approach" Wave Motion 107: DOI: 10.1016/j.wavemoti.2021.102823.
- [6] L. Akinyemi, M. ¸Senol, and O. S. Iyiola, (2021) “Exact solutions of the generalized multidimensional mathematical physics models via sub-equation method" Mathematics and Computers in Simulation 182: 211–233. DOI:10.1016/j.matcom.2020.10.017.
- [7] L. Akinyemi, (2021) “Two improved techniques for the perturbed nonlinear Biswas–Milovic equation and its optical solitons" Optik 243: DOI:10.1016/j.ijleo.2021.167477.
- [8] X.-Y. Gao, Y.-J. Guo, andW.-R. Shan, (2021) “Beholding the shallow water waves near an ocean beach or in a lake via a Boussinesq-Burgerssystem" Chaos, Solitons and Fractals 147: DOI: 10.1016/j.chaos.2021.110875.
- [9] A. Yoku¸s, (2021) “Construction of different types of traveling wave solutions of the relativistic wave equation associated with the Schr¨odinger equation" Mathematical Modelling and Numerical Simulation with Applications 1(1): 24–31.
- [10] J.-H. He and Y. O. El-Dib, (2021) “The reducing rank method to solve third-order Duffing equation with the homotopy perturbation" Numerical Methods for Partial Differential Equations 37(2): 1800–1808. DOI: 10.1002/num.22609.
- [11] J. Singh, A. Ahmadian, S. Rathore, D. Kumar, D. Baleanu, M. Salimi, and S. Salahshour, (2021) “An efficient computational approach for local fractional Poisson equation in fractal media" Numerical Methods for Partial Differential Equations 37(2): 1439–1448. DOI: 10.1002/num.22589.
- [12] H. Tajadodi, Z. A. Khan, A. u. Rehman Irshad, J. Gómez-Aguilar, A. Khan, and H. Khan, (2021) “Exact solutions of conformable fractional differential equations" Results in Physics 22: DOI: 10.1016/j.rinp.2021.103916.
- [13] M. M. Khater, T. A. Nofal, H. Abu-Zinadah, M. S. Lotayif, and D. Lu, (2021) “Novel computational and accurate numerical solutions of the modified Benjamin–Bona–Mahony (BBM) equation arising in the optical illusions field" Alexandria Engineering Journal 60(1): 1797–1806. DOI: 10.1016/j.aej.2020.11.028.
- [14] M. M. A. Khater, R. A. M. Attia, S. K. Elagan, and F. S. Bayones, (2021) “ANALYTICAL AND SEMI ANALYTICAL SOLUTIONS OF THE INTERNAL WAVES OF DEEP-STRATIFIED FLUIDS" Thermal Science 25(SpecialIssue 2): S227–S232. DOI: 10.2298/TSCI21S2227K.
- [15] M. M. A. Khater, S. Anwar, K. U. Tariq, and M. S. Mohamed, (2021) “Some optical soliton solutions to the perturbed nonlinear Schrödinger equation by modified Khater method" AIP Advances 11(2): DOI: 10.1063/5.0038671.
- [16] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear engineering 4(1): 15–22.
- [17] M. M. Khater, (2021) “Numerical simulations of Zakharov’s (ZK) non-dimensional equation arising in Langmuir and ion-acoustic waves" Modern Physics Letters B 35(31): 2150480.
- [18] M. M. Khater, (2021) “Diverse bistable dark novel explicit wave solutions of cubic–quintic nonlinear Helmholtz model" Modern Physics Letters B 35(26): 2150441.
- [19] M. M. Khater, (2021) “Abundant breather and semianalytical investigation: On high-frequency waves’ dynamics
in the relaxation medium" Modern Physics Letters B 35(22): 2150372. - [20] M. M. A. Khater, (2021) “Diverse solitary and Jacobian solutions in a continually laminated fluid with respect to shear flows through the Ostrovsky equation" Modern Physics Letters B 35(13): DOI: 10.1142 /S0217984921502201.
- [21] M. M. Khater, S. Elagan, M. El-Shorbagy, S. Alfalqi, J. Alzaidi, and N. A. Alshehri, (2021) “Folded novel accurate analytical and semi-analytical solutions of a generalized Calogero-Bogoyavlenskii-Schiff equation" Communications in Theoretical Physics 73(9): DOI: 10.1088/1572-9494/ac049f.
- [22] M. M. A. Khater and D. Lu, (2021) “Analytical versus numerical solutions of the nonlinear fractional time-space telegraph equation" Modern Physics Letters B 35(19): DOI: 10.1142/S0217984921503243.
- [23] M. M. Khater, M. S. Mohamed, and R. A. Attia, (2021) “On semi analytical and numerical simulations for a mathematical biological model; the time-fractional nonlinear Kolmogorov– Petrovskii–Piskunov (KPP) equation" Chaos, Solitons and Fractals 144: DOI: 10.1016/j.chaos.2021.110676.
- [24] M. M. A. Khater and B. Ghanbari, (2021) “On the solitary wave solutions and physical characterization of gas diffusion in a homogeneous medium via some efficient techniques" European Physical Journal Plus 136(4): DOI: 10.1140/epjp/s13360-021-01457-1.
- [25] M. M. Khater, K. S. Nisar, and M. S. Mohamed, (2021) “Numerical investigation for the fractional nonlinear spacetime telegraph equation via the trigonometric Quintic B-spline scheme" Mathematical Methods in the Applied Sciences 44(6): 4598–4606.
- [26] M. M. Khater, A. Mousa, M. El-Shorbagy, and R. A. Attia, (2021) “Analytical and semi-analytical solutions for Phi-four equation through three recent schemes" Results in Physics 22: DOI: 10.1016/j.rinp.2021.103954.
- [27] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da Sociedade Paranaense de Matematica 34(2): 213–229. DOI: 10.5269/bspm.v34i2.25501.
- [28] M. M. Khater, A. E.-S. Ahmed, S. Alfalqi, J. Alzaidi, S. Elbendary, and A. M. Alabdali, (2021) “Computational and approximate solutions of complex nonlinear Fokas–Lenells equation arising in optical fiber" Results in Physics 25: DOI: 10.1016/j.rinp.2021.104322.
- [29] M. M. Khater, A. E.-S. Ahmed, and M. El-Shorbagy, (2021) “Abundant stable computational solutions of Atangana–Baleanu fractional nonlinear HIV-1 infection of CD4+ T-cells of immunodeficiency syndrome" Results in Physics 22: DOI: 10.1016/j.rinp.2021.103890.
- [30] J. Zhang, D. Lu, S. A. Salama, and M. M. A. Khater,(2022) “Accurate demonstrating of the interactions of two long waves with different dispersion relations: Generalized Hirota-Satsuma couple KdV equation" AIP Advances 12(2): DOI: 10.1063/5.0084588.
- [31] L. Akinyemi, M. Mirzazadeh, and K. Hosseini, (2022) “Solitons and other solutions of perturbed nonlinear Biswas–Milovic equation with Kudryashov’s law of refractive index" Nonlinear Analysis: Modelling and Control 27(3): 479–495. DOI: 10.15388/namc.2022.27.26374.
- [32] S. Abbagari, A. Houwe, L. Akinyemi, Y. Saliou, and T. B. Bouetou, (2022) “Modulation instability gain and discrete soliton interaction in gyrotropic molecular chain" Chaos, Solitons and Fractals 160: DOI: 10.1016/j.chaos.2022.112255.
- [33] H. Ahmad, M. N. Alam, and M. Omri, (2021) “New computational results for a prototype of an excitable system" Results in Physics 28: DOI: 10.1016/j.rinp.2021.104666.
- [34] G. Akram, M. Sadaf, and I. Zainab, (2022) “Observations of fractional effects of β-derivative and M-truncated derivative for space time fractional Phi-4 equation via two analytical techniques" Chaos, Solitons and Fractals 154: DOI: 10.1016/j.chaos.2021.111645.
- [35] G. Akram, M. Sadaf, and M. A. U. Khan, (2023) “Soliton solutions of the resonant nonlinear Schr ¨odinger equation using modified auxiliary equation method with three different nonlinearities" Mathematics and Computers in Simulation 206: 1–20. DOI: 10.1016/j.matcom.2022.10.032.
- [36] H. Ahmad, T. A. Khan, P. S. Stanimirovic, W. Shatanawi, and T. Botmart, (2022) “New approach on conventional solutions to nonlinear partial differential equations describing physical phenomena" Results in Physics 41: DOI: 10.1016/j.rinp.2022.105936.
- [37] Hamood-Ur-Rehman, M. I. Asjad, M. Inc, and I. Iqbal, (2022) “Exact solutions for new coupled Konno–Oono equation via Sardar subequation method" Optical and Quantum Electronics 54(12): DOI: 10.1007/s11082-022-04208-3.
- [38] M. S. M. Shehata, H. Rezazadeh, E. H. M. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New Optical Soliton Solutions of the Perturbed Fokas-Lenells Equation" Communications in Theoretical Physics 71(11): 1275–1280. DOI: 10.1088/0253-6102/71/11/1275.
- [39] W. A. Faridi, M. I. Asjad, and S. M. Eldin, (2022) “Exact Fractional Solution by Nucci’s Reduction Approach and New Analytical Propagating Optical Soliton Structures in Fiber-Optics" Fractal and Fractional 6(11): DOI: 10.3390/fractalfract6110654.
- [40] M. Jaradat, A. Batool, A. R. Butt, and N. Raza, (2022) “New solitary wave and computational solitons for Kundu–Eckhaus equation" Results in Physics 43: DOI: 10.1016/j.rinp.2022.106084.
- [41] T. A. Alrebdi, N. Raza, S. Arshed, and A.-H. Abdel-Aty, (2022) “New solitary wave patterns of Fokas-System arising in monomode fiber communication systems" Optical and Quantum Electronics 54(11): DOI: 10.1007/s11082-022-04062-3.
- [42] J. Dikwa, A. Houwe, S. Abbagari, L. Akinyemi, and M. Inc, (2022) “Modulated waves patterns in the photovoltaic photorefractive crystal" Optical and Quantum Electronics 54(12): DOI: 10.1007/s11082-022-04224-3.
- [43] A. Houwe, Y. Saliou, P. Djorwe, S. Abbagari, L. Akinyemi, and S. Y. Doka, (2022) “Modulation instability gain and modulated wave shape incited by the acoustic longitudinal vibrations in molecular chain model" Physica Scripta 97(8): DOI: 10.1088/1402-4896/ac7a6b.
- [44] H. S. Ali, M. Habib, M. M. Miah, M. M. Miah, and M. A. Akbar, (2023) “Diverse solitary wave solutions of fractional order Hirota-Satsuma coupled KdV system using two expansion methods" Alexandria Engineering Journal 66: 1001–1014. DOI: 10.1016/j.aej.2022.12.021.
- [45] M. A. Akbar, F. A. Abdullah, and M. M. Haque, (2023) “Analytical soliton solutions of the perturbed fractional nonlinear Schr¨odinger equation with space–time beta derivative by some techniques" Results in Physics 44: DOI: 10.1016/j.rinp.2022.106170.
- [46] S. Kumar, B. Mohan, and R. Kumar, (2022) “Lump, soliton, and interaction solutions to a generalized twomode higher-order nonlinear evolution equation in plasma physics" Nonlinear Dynamics 110(1): 693–704. DOI: 10.1007/s11071-022-07647-5.
- [47] S. K. Mohanty, S. Kumar, A. N. Dev, M. K. Deka, D. V. Churikov, and O. V. Kravchenko, (2022) “An efficient technique of [Formula presented]–expansion method for modified KdV and Burgers equations with variable coefficients" Results in Physics 37: DOI: 10.1016/j.rinp.2022.105504.
- [48] A. R. Seadawy, S. T. R. Rizvi, S. Ahmed, and T. Batool, (2023) “Propagation of W-shaped and M-shaped solitons with multi-peak interaction for ultrashort light pulse in fibers" Optical and Quantum Electronics 55(3): DOI: 10.1007/s11082-022-04478-x.
- [49] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17): DOI: 10.1142/S0217984919501963.
- [50] S. T. R. Rizvi, A. R. Seadawy, S. K. Naqvi, and S. O. Abbas, (2023) “Study of mixed derivative nonlinear Schr¨odinger equation for rogue and lump waves, breathers and their interaction solutions with Kerr law" Optical and Quantum Electronics 55(2): DOI: 10.1007/s11082-022-04415-y.
- [51] Z. Zhao, L. He, and A.-M.Wazwaz, (2023) “Dynamics of lump chains for the BKP equation describing propagation of nonlinear waves" Chinese Physics B:
- [52] Z. Zhao, J. Yue, and L. He, (2022) “New type of multiple lump and rogue wave solutions of the (2+1)-dimensional Bogoyavlenskii–Kadomtsev–Petviashvili equation" Applied Mathematics Letters 133: DOI: 10.1016/j.aml.2022.108294.
- [53] Z. Zhao, (2019) “Conservation laws and nonlocally related systems of the Hunter–Saxton equation for liquid crystal" Analysis and Mathematical Physics 9(4):2311–2327. DOI: 10.1007/s13324-019-00337-3.
- [54] Z. Zhao and L. He, (2021) “Lie symmetry, nonlocal symmetry analysis, and interaction of solutions of a (2+1)-dimensional KdV–mKdV equation" Theoretical and Mathematical Physics 206(2): 142–162.
- [55] Z. Zhao and L. He, (2021) “Resonance Y-type soliton and hybrid solutions of a (2+1)-dimensional asymmetrical Nizhnik–Novikov–Veselov equation" Applied Mathematics Letters 122: DOI: 10.1016/j.aml.2021.107497.
- [56] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable timefractional
derivative" Calcolo 53(3): 475–485. DOI: 10.1007/s10092-015-0158-8. - [57] M. Hashemi and Z. Balmeh, (2018) “On invariant analysis and conservation laws of the time fractional variant Boussinesq and coupled Boussinesq-Burger’s equations" European Physical Journal Plus 133(10): DOI: 10.1140/epjp/i2018-12289-1.
- [58] F.-L. Xia, F. Jarad, M. S. Hashemi, and M. B. Riaz, (2022) “A reduction technique to solve the generalized nonlinear dispersive mK(m,n) equation with new local derivative" Results in Physics 38: DOI: 10.1016/j.rinp.2022.105512.
- [59] M. Hashemi, (2021) “A novel approach to find exact solutions of fractional evolution equations with non-singular kernel derivative" Chaos, Solitons and Fractals 152:DOI: 10.1016/j.chaos.2021.111367.
- [60] R. Johnson and S. Thompson, (1978) “A solution of the inverse scattering problem for the Kadomtsev-Petviashvili equation by the method of separation of variables" Physics Letters A 66(4): 279–281. DOI: 10.1016/0375-9601(78)90236-0.
- [61] W.-X. Ma, (2015) “Lump solutions to the Kadomtsev-Petviashvili equation" Physics Letters, Section A: General, Atomic and Solid State Physics 379(36): 1975–1978. DOI: 10.1016/j.physleta.2015.06.061.
- [62] M. El-Sabbagh and A. Ali, (2005) “New exact solutions for (3+1)-dimensional Kadomtsev-Petviashvili equation and generalized (2+1)-dimensional Boussinesq equation" International Journal of Nonlinear Sciences and Numerical Simulation 6(2): 151–162. DOI: 10.1515/IJNSNS.2005.6.2.151.
- [63] X. Yong, W.-X. Ma, Y. Huang, and Y. Liu, (2018) “Lump solutions to the Kadomtsev–Petviashvili I equation with a self-consistent source" Computers and Mathematics with Applications 75(9): 3414–3419. DOI: 10.1016/j.camwa.2018.02.007.
- [64] W.-X. Ma, X. Yong, and X. Lü, (2021) “Soliton solutions to the B-type Kadomtsev–Petviashvili equation under general dispersion relations"Wave Motion 103: DOI: 10.1016/j.wavemoti.2021.102719.
- [65] J.-W. Xia, Y.-W. Zhao, and X. Lü, (2020) “Predictability, fast calculation and simulation for the interaction solutions to the cylindrical Kadomtsev-Petviashvili equation" Communications in Nonlinear Science and Numerical Simulation 90: DOI: 10.1016/j.cnsns.2020.105260.
- [66] C. Wang and H. Fang, (2020) “General high-order localized waves to the Bogoyavlenskii–Kadomtsev–Petviashvili equation" Nonlinear Dynamics 100(1): 583–599. DOI: 10.1007/s11071-020-05499-5.
- [67] H. F. Ismael, W.-X. Ma, and H. Bulut, (2021) “Dynamics of soliton and mixed lump-soliton waves to a generalized Bogoyavlensky-Konopelchenko equation" Physica Scripta 96(3): DOI: 10.1088/1402-4896/abdc55.
- [68] L. Cheng, Y. Zhang, W.-X. Ma, and J.-Y. Ge, (2021) “Wronskian and lump wave solutions to an extended second KP equation" Mathematics and Computers in Simulation 187: 720–731. DOI: 10.1016/j.matcom.2021.03.024.
- [69] Y.-L. Wang, Y.-T. Gao, S.-L. Jia, G.-F. Deng, and W.-Q. Hu, (2017) “Solitons for a (2 + 1)-dimensional variablecoefficient Bogoyavlensky-Konopelchenko equation in a fluid" Modern Physics Letters B 31(25): DOI: 10.1142/S0217984917502165.
- [70] F. Calogero and A. Degasperis, (1976) “Nonlinear evolution equations solvable by the inverse spectral transform.-I" Il Nuovo Cimento B Series 11 32(2): 201–242. DOI: 10.1007/BF02727634.
- [71] A. Atangana, D. Baleanu, and A. Alsaedi, (2015) “New properties of conformable derivative" Open Mathematics 13(1): 889–898. DOI: 10.1515/math-2015-0081.
- [72] H. Rezazadeh, (2018) “New solitons solutions of the complex Ginzburg-Landau equation with Kerr law nonlinearity" Optik 167: 218–227. DOI: 10.1016/j.ijleo.2018.04.026.