Wan Nura’in Nabilah Noranuar1, Ahmad Qushairi Mohamad 1, Sharidan Shafie1, and Ilas Khan2
1Department of Mathematical Sciences, Faculty of Science, Universiti Teknologi Malaysia, 81310 Johor Bahru, Johor, Malaysia
2Basic Engineering Sciences Department, College of Engineering Majmaah University, Majmaah 11952, Saudi Arabia
Received:
December 6, 2020
Accepted:
February 19, 2021
Publication Date:
October 11, 2021
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
Download Citation: ||https://doi.org/10.6180/jase.202206_25(3).0005
ABSTRACT
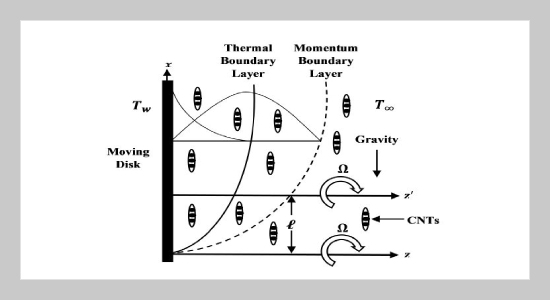
Nanofluid is one of the significant developments for having an efficient heat transport process. Its implementation in a non-coaxial rotating system has benefited from designing a mixer machine with two stirrer blades, cooling fan, and jet engines. This study analytically investigates the free convection of unsteady non-coaxial rotating nanofluid flow through a moving disk. The suspension of single-wall or multi-wall carbon nanotubes in water is known as the nanofluid in this study. The fluid motion is affected by the effects of rotation and buoyancy forces. Using suitable dimensionless variables, the dimensional coupled partial differential of momentum and energy equations along with their initial and moving boundary conditions are converted into the dimensionless form. The expressions for temperature and velocity profiles are obtained by solving governing equations using Laplace transform method. The validity of obtained solution is confirmed by having a good agreement when comparing present results with the published result. The results show that the insertion of CNTs particles into the rotating water causes the temperature and velocity profiles to increase. The amount of heat transferred by SWCNTs is greater than MWCNTs. Increasing CNTs particles has descended both primary and secondary skin friction but increase Nusselt number. Further analysis with the help of pictorial discussion for the fluid flows and heat transfer under the influences of nanoparticle volume fraction, Grashof number, the amplitude of disk, and time is carried out.
Keywords:
Non-coaxial rotation, Nanofluid, Carbon nanotubes, Laplace transform method, Moving disk
REFERENCES
- [1] S. U. Choi. “Enhancing thermal conductivity of fluids with nanoparticles”. In: American Society of Mechanical Engineers, Fluids Engineering Division (Publication) FED. 231. 1995, 99–105.
- [2] P. Sivashanmugam. “Application of Nanofluids in Heat Transfer”. In: An Overview of Heat Transfer Phenomena. 2012. DOI: 10.5772/52496.
- [3] , (2019) “Free convection flow of nanofluid over infinite vertical plate with damped thermal flux" Chinese Journal of Physics 59: 175–188. DOI: 10.1016/j.cjph.2019.02.029.
- [4] T. Anwar, P. Kumam, Z. Shah, W. Watthayu, and P. Thounthong, (2020) “Unsteady radiative natural convective MHD nanofluid flow past a porous moving vertical plate with heat source/sink" Molecules 25(4): DOI:10.3390/molecules25040854.
- [5] W. A. Azhar, D. Vieru, and C. Fetecau, (2017) “Free convection flow of some fractional nanofluids over a moving vertical plate with uniform heat flux and heat source" Physics of Fluids 29(8): DOI: 10.1063/1.4996034.
- [6] S. Nasir, Z. Shah, S. Islam,W. Khan, and S. N. Khan, (2019) “Radiative flow of magneto hydrodynamics singlewalled carbon nanotube over a convectively heated stretchable rotating disk with velocity slip effect" Advances in Mechanical Engineering 11(3): DOI: 10.1177/1687814019827713.
- [7] A. Khalid, L. Y. Jiann, I. Khan, and S. Shafie. “Exact solutions for unsteady free convection flow of carbon nanotubes over an oscillating vertical plate”. In: AIP Conference Proceedings. 1830. 2017. DOI: 10.1063/1.4980917.
- [8] A. Khalid, I. Khan, A. Khan, S. Shafie, and I. Tlili, (2018) “Case study of MHD blood flow in a porous medium with CNTS and thermal analysis" Case Studies in Thermal Engineering 12: 374–380. DOI: 10.1016/j.csite.2018.04.004.
- [9] N. Acharya, R. Bag, and P. K. Kundu, (2020) “On the mixed convective carbon nanotube flow over a convectively heated curved surface" Heat Transfer 49(4): 1713–1735. DOI: 10.1002/htj.21687.
- [10] B. Mahanthesh, B. J. Gireesha, N. S. Shashikumar, and S. A. Shehzad, (2017) “Marangoni convectiveMHD flow of SWCNT and MWCNT nanoliquids due to a disk with solar radiation and irregular heat source" Physica E: Low-Dimensional Systems and Nanostructures 94: 25–30. DOI: 10.1016/j.physe.2017.07.011.
- [11] N. S. Shashikumar, B. J. Gireesha, B. Mahanthesh, and B. C. Prasannakumara, (2018) “Brinkman-Forchheimer flow of SWCNT and MWCNT magnetonanoliquids in a microchannel with multiple slips and Joule heating aspects" Multidiscipline Modeling in Materials and Structures 14(4): 769–786. DOI: 10.1108/MMMS-01-2018-0005.
- [12] S. A. Shehzad, B. Mahanthesh, B. J. Gireesha, N. S. Shashikumar, and M. Madhu, (2019) “Brinkman-Forchheimer slip flow subject to exponential space and thermal-dependent heat source in a microchannel utilizing SWCNT and MWCNT nanoliquids" Heat Transfer- Asian Research 48(5): 1688–1708. DOI: 10.1002/htj.21452.
- [13] A. A. Al-Rashed, K. Kalidasan, L. Kolsi, A. Aydi, E. H. Malekshah, A. K. Hussein, and P. Rajesh Kanna, (2018) “Three-dimensional investigation of the effects of external magnetic field inclination on laminar natural convection heat transfer in CNT–water nanofluid filled cavity" Journal of Molecular Liquids 252: 454–468. DOI: 10.1016/j.molliq.2018.01.006.
- [14] Z. H. Khan,W. A. Khan, R. U. Haq, M. Usman, and M. Hamid, (2020) “Effects of volume fraction on water-based carbon nanotubes flow in a right-angle trapezoidal cavity: FEM based analysis" International Communications in Heat and Mass Transfer 116: DOI: 10.1016/j.icheatmasstransfer.2020.104640.
- [15] A. Ebaid and M. A. Al Sharif, (2015) “Application of laplace transform for the exact effect of a magnetic field on heat transfer of carbon nanotubes-suspended nanofluids" Zeitschrift fur Naturforschung - Section A Journal of Physical Sciences 70(6): 471–475. DOI: 10.1515/zna-2015-0125.
- [16] M. Saqib, I. Khan, and S. Shafie, (2018) “Natural convection channel flow of CMC-based CNTs nanofluid" European Physical Journal Plus 133(12): DOI: 10.1140/epjp/i2018-12340-3.
- [17] N. Acharya, (2021) “Spectral Simulation to Investigate the Effects of Active Passive Controls of Nanoparticles on the Radiative Nanofluidic Transport Over a Spinning Disk" Journal of Thermal Science and Engineering Applications 13(3): DOI: 10.1115/1.4048677.
- [18] N. Acharya, (2020) “Framing the Impacts of Highly Oscillating Magnetic Field on the Ferrofluid Flow Over a Spinning Disk Considering Nanoparticle Diameter and Solid–Liquid Interfacial Layer" Journal of Heat Transfer 142(10): DOI: 10.1115/1.4047503.
- [19] P. R. J. Gowda, N. R. Kumar, A. Aldalbahi, A. Issakhov, B. C. Prasannakumara, M. Rahimi-Gorji, and M. Rahaman, (2021) “Thermophoretic particle deposition in time-dependent flow of hybrid nanofluid over rotating and vertically upward/ downward moving disk" Surfaces and Interfaces 22: 100864.
- [20] P. G. R. Jayadevamurthy, N. kumar Rangaswamy, B. C. Prasannakumara, and K. S. Nisar, (2020) “Emphasis on unsteady dynamics of bioconvective hybrid nanofluid flow over an upward–downward moving rotating disk" Numerical Methods for Partial Differential Equations: DOI: 10.1002/num.22680.
- [21] N. Acharya, S. Maity, and P. K. Kundu, (2019) “Framing the hydrothermal features of magnetized TiO2–CoFe2O4 water-based steady hybrid nanofluid flow over a radiative revolving disk" Multidiscipline Modeling in Materials and Structures 16(4): 765–790. DOI: 10.1108/MMMS-08-2019-0151.
- [22] N. Acharya, K. Das, and P. K. Kundu, (2018) “Rotating flow of carbon nanotube over a stretching surface in the presence of magnetic field: A comparative study" Applied Nanoscience (Switzerland) 8(3): 369–378. DOI: 10.1007/s13204-018-0794-9.
- [23] F. Rehman, M. I. Khan, M. Sadiq, and A. Malook, (2017) “MHD flow of carbon in micropolar nanofluid with convective heat transfer in the rotating frame" Journal of Molecular Liquids 231: 353–363. DOI: 10.1016/j.molliq.2017.02.022.
- [24] B. Mahanthesh, B. J. Gireesha, I. L. Animasaun, T. Muhammad, and N. S. Shashikumar, (2019) “MHD flow of SWCNT and MWCNT nanoliquids past a rotating stretchable disk with thermal and exponential space dependent heat source" Physica Scripta 94(8): DOI: 10.1088/1402-4896/ab18ba.
- [25] M. J. Kotresh, G. K. Ramesh, V. K. R. Shashikala, and B. C. Prasannakumara, (2020) “Assessment of Arrhenius activation energy in stretched flow of nanofluid over a rotating disc" Heat Transfer: DOI: 10.1002/htj.22006.
[26] A. Q. Mohamad, I. Khan, Z. Ismail, and S. Shafie, (2016) “Exact solutions for unsteady free convection flow over an oscillating plate due to non-coaxial rotation" SpringerPlus 5(1): DOI: 10.1186/s40064-016-3748-2.- [27] H. V. Ersoy. Effect of magnetic field on the time-dependent flow due to a disk with non-torsional oscillation and a Newtonian fluid at infinity rotating about distinct axes. 2019. DOI: 10.1007/s12046-019-1132-y.
- [28] S. Das, B. Tarafdar, and R. N. Jana, (2018) “Hall effects on magnetohydrodynamics flow of nanofluids due to noncoaxial rotation of a porous disk and a fluid at infinity" Journal of Nanofluids 7(6): 1172–1186. DOI: 10.1166/jon.2018.1527.
- [29] T. S. Ashlin and B. Mahanthesh, (2019) “Exact solution of non-coaxial rotating and non-linear convective flow of cu–al2 o3 –h2 o hybrid nanofluids over an infinite vertical plate subjected to heat source and radiative heat" Journal of Nanofluids 8(4): 781–794. DOI: 10.1166/jon.2019.1633.
- [30] Q. Z. Xue, (2005) “Model for thermal conductivity of carbon nanotube-based composites" Physica B: Condensed Matter 368(1-4): 302–307. DOI: 10.1016/j.physb.2005.07.024.
- [31] H. Villinger, (1985) “Solving cylindrical geothermal problems using the Gaver-Stehfest inverse Laplace transform." Geophysics 50(10): 1581–1587. DOI: 10.1190/1.1441848.
- [32] H. Stehfest, (1970) “Algorithm 368: Numerical inversion of Laplace transforms [D5]" Communications of the ACM 13(1): 47–49. DOI: 10.1145/361953.361969.