Influence of Anisotropy on the Love Waves in a Self-reinforced Medium

A.  Pradhan; S.K. Samal; N.C. Mahanti

doi:10.6180/jase.2003.6.3.06

Influence of Anisotropy on the Love Waves in a Self-reinforced Medium

A. Pradhan This email address is being protected from spambots. You need JavaScript enabled to view it.¹, S. K. Samal¹ and N. C. Mahanti¹

¹Department of Applied Mathematics Birla Institute of Technology Mesra, Ranchi 835215, India

Received: December 2, 2002
Accepted: June 17, 2003
Publication Date: September 1, 2003

Download Citation: ||https://doi.org/10.6180/jase.2003.6.3.06

ABSTRACT

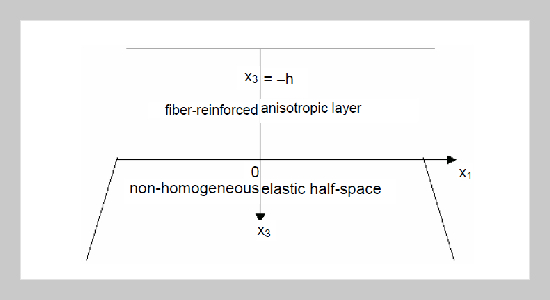
Dispersion of Love waves is studied in a self-reinforced layer lying over an elastic non-homogeneous half space. The wave velocity equation is found to be in perfect agreement with the corresponding classical result when the anisotropic elastic parameters tend to zero. Dispersion curves for the shear wave velocity ratio and the effect of anisotropy and heterogeneity have been shown graphically. It is observed that the shear wave velocity ratio of Love waves is greater for the heterogeneous half-space than for the homogeneous one. The differences are quite distinct for the smaller values of wave numbers.

Keywords: Anisotropy, Love Waves, Self-reinforced Medium, Shear Wave Velocity, Inhomogeneity Parameter

REFERENCES

[1] Richter, C. F., Elementary Seismology, Freeman, San Francisco, U.S.A., 85,646,96 (1958).
[2] Belfield, A. J., Rogers, T. G. and Spencer, A. J. M., “Stress in Elastic Plates Reinforced by Fibers Lying in Concentric Circles,” J. Mech. Phy. Solids, Vol. 31, 259 (1983).
[3] Lord Rayleigh, “Theory of Sound,” Dover, NY, U.S.A. (1945).
[4] Konczak, Z., “The Propagation of Love Waves in a Fluid-saturated Porous Anisotropic Layer,” Acta Mechanica, Vol. 79, pp. 155-168 (1989).
[5] Ewing, W. M., Jardetzky, W. S. and Press, H., Elastic Waves in Layered Media, McGrawHill, NY, U.S.A. (1957).
[6] Chakraborty, S. K. and Dey, S., “The Propagation of Love Waves in Watersaturated Soil Underlain by Hetero- geneous Elastic Medium,” Acta Mechanica, Vol. 44, pp. 169-176 (1982).
[7] Chattopadhyay, A. and Choudhury, S., “Propagation, Reflection and Trans mission of Magneto-elastic Shear Waves in a Self-reinforced Medium,” Int. J. Eng. Sci., Vol. 28, 485-495 (1990).
[8] Chattopadhyay, A. and Choudhury, S., “Magneto-elastic Shear Waves in a Selfreinforced Plate,” Int. J. Numer. Anal. Methods Geomech., Vol. 19, pp. 289-304 (1995).
[9] Sengupta, P. R. and Nath, S., “Surface Waves in Fiber-reinforced Anisotropic Elastic Medium,” Sādhanā (The Indian Academy of Sciences, Bangalore, India), Vol. 26, pp. 363- 370 (2001).
[10] Bullen, K. E., An Introduction to the Theory of Seismology, Cambridge University Press, London, U.K. (1965).
[11] Spencer, A. J. M., Deformation of Fiberreinforced Material, Oxford University Press, London, U.K. (1972).