1School of Electrical Engineering and Automation, Henan Institute of Technology, Xinxiang, Henan 453003, China
2School of Electrical Engineering and Automation, Hefei University of Technology, Hefei, Anhui 230009, China
Received:
March 2, 2023
Accepted:
July 28, 2023
Publication Date:
September 10, 2023
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
Download Citation: ||https://doi.org/10.6180/jase.202404_27(4).0003
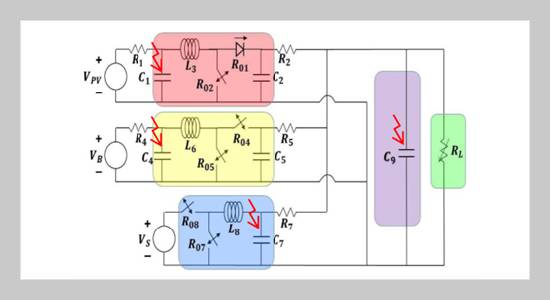
In the present work, using the State-Dependent Riccati Equation approach, the optimal optimizer-controller is designed for a small DC network isolated from the network. The objectives are to control the output voltage of the solar cell, as well as output voltages of the battery, the capacitor bank and the DC busbar, and to detect possible faults in a timely manner. In the State-Dependent Riccati Equation observer–controller design process, a non-linear model is used for modeling the dynamic behavior of the microgrid in different operating conditions. The efficiency of the studied microgrid has been assessed in the presence of uncertainty in system parameters and measurement noise. The results of the simulations show the ability of the suggested approach to detect faults in a timely manner, not to recognize the disturbance as a fault, as well as the effective and resistant performance of the progressive controller even in the disturbance presence. Quantitatively, the proposed fault detection method was able to generate non-zero residual current in the presence of faults, allowing for fault detection by defining an appropriate threshold. The threshold used in the study was 50. Additionally, the fault detection system was able to avoid misdiagnosis of disturbances as faults.
Keywords:
Micro grid, State-Dependent Riccati Equation, Fault Detection, DC network
- [1] S. Huang and O. Abedinia, (2021) “Investigation in economic analysis of microgrids based on renewable energy uncertainty and demand response in the electricity market" Energy 225: 120247. DOI: https://doi.org/10.1016/j.energy.2021.120247.
- [2] C. Wang, Z. Zhang, O. Abedinia, and S. G. Farkoush, (2021) “Modeling and analysis of a microgrid considering the uncertainty in renewable energy resources, energy storage systems and demand management in electrical retail market" Journal of Energy Storage 33: 102111. DOI: https://doi.org/10.1016/j.est.2020.102111.
- [3] J.-D. Park and J. Candelaria, (2013) “Fault Detection and Isolation in Low-Voltage DC-Bus Microgrid System" IEEE Transactions on Power Delivery 28(2): 779–787. DOI: 10.1109/TPWRD.2013.2243478.
- [4] J.-D. Park, J. Candelaria, L. Ma, and K. Dunn, (2013) “DC Ring-Bus Microgrid Fault Protection and Identification of Fault Location" IEEE Transactions on Power Delivery 28(4): 2574–2584. DOI: 10.1109/TPWRD.2013.2267750.
- [5] G. P. Adam, F. Alsokhiry, and A. Alabdulwahab, (2022) “DC grid controller for optimized operation of voltage source converter based multi-terminal HVDC networks" Electric Power Systems Research 202: 107595. DOI: https://doi.org/10.1016/j.epsr.2021.107595.
- [6] A. Ahl, M. Yarime, M. Goto, S. S. Chopra, N. M. Kumar, K. Tanaka, and D. Sagawa, (2020) “Exploring blockchain for the energy transition: Opportunities and challenges based on a case study in Japan" Renewable and Sustainable Energy Reviews 117: 109488. DOI: https://doi.org/10.1016/j.rser.2019.109488.
- [7] M. A. Zamani, T. S. Sidhu, and A. Yazdani, (2011) “A Protection Strategy and Microprocessor-Based Relay for Low-Voltage Microgrids" IEEE Transactions on Power Delivery 26(3): 1873–1883. DOI: 10.1109/TPWRD.2011.2120628.
- [8] S. Matos, M. Vargas, L. Fracalossi, L. Encarnação, and O. Batista, (2021) “Protection philosophy for distribution grids with high penetration of distributed generation" Electric Power Systems Research 196: 107203. DOI: https://doi.org/10.1016/j.epsr.2021.107203.
- [9] A. Hwas and R. Katebi. “Nonlinear observer-based fault detection and isolation for wind turbines”. In: 22nd Mediterranean Conference on Control and Automation. 2014, 870–875. DOI: 10.1109/MED.2014.6961483.
- [10] M. Esreraig and J. Mitra. “An observer-based protection system for microgrids”. In: 2011 IEEE Power and Energy Society General Meeting. 2011, 1–7. DOI: 10.1109/PES.2011.6039818.
- [11] M. Esreraig and J. Mitra, (2015) “Microgrid protection using system observer and minimum measurement set" International Transactions on Electrical Energy Systems 25(4): 607–622. DOI: https://doi.org/10.1002/etep.1849. eprint: https://onlinelibrary.wiley.com/doi/pdf/10.1002/etep.1849.
- [12] T. Çimen, (2010) “Systematic and effective design of nonlinear feedback controllers via the state-dependent Riccati equation (SDRE) method" Annual Reviews in Control 34(1): 32–51. DOI: https://doi.org/10.1016/j.arcontrol.2010.03.001.
- [13] T. Çimen, (2012) “Survey of State-Dependent Riccati Equation in Nonlinear Optimal Feedback Control Synthesis" Journal of Guidance, Control, and Dynamics 35(4): 1025–1047. DOI: 10.2514/1.55821.
- [14] T. T. Darabseh, (2022) “Nonlinear State Dependent Riccati Equation Controller for 3-DOF Airfoil with Cubic Structural Nonlinearity" International Review of Aerospace Engineering 15(2): 97–111. DOI: 10.15866/irease.v15i2.21426.
- [15] Y. Batmani and H. Khaloozadeh, (2016) “On the design of suboptimal sliding manifold for a class of nonlinear uncertain time-delay systems" International Journal of Systems Science 47(11): 2543–2552. DOI: 10.1080/00207721.2014.999263.
- [16] Y. Batmani and H. Khaloozadeh, (2014) “On the design of observer for nonlinear time-delay systems" Asian Journal of Control 16(4): 1191–1201. DOI: 10.1002/asjc.795.
- [17] Y. Batmani and H. Khaloozadeh, “Optimal chemotherapy in cancer treatment: state dependent Riccati equation control and extended Kalman filter" Optimal Control Applications and Methods 34(5): 562–577. DOI: https://doi.org/10.1002/oca.2039. eprint: https://onlinelibrary.wiley.com/doi/pdf/10.1002/oca.2039.
- [18] T. D. Do, H. H. Choi, and J.-W. Jung, (2012) “SDREBased Near Optimal Control System Design for PM Synchronous Motor" IEEE Transactions on Industrial Electronics 59(11): 4063–4074. DOI: 10.1109/TIE.2011.2174540.
- [19] Y. Batmani, M. Davoodi, and N. Meskin, (2017) “Nonlinear Suboptimal Tracking Controller Design Using State-Dependent Riccati Equation Technique" IEEE Transactions on Control Systems Technology 25(5): 1833–1839. DOI: 10.1109/TCST.2016.2617285.