Journal of Applied Science and Engineering

Published by Tamkang University Press

1.30

Impact Factor

2.10

CiteScore

Mathematics / Statistics

Jing Ran This email address is being protected from spambots. You need JavaScript enabled to view it.

Mathematics teaching department, Sichuan University Jinjiang College, Meishan 620000, Sichuan, China

 

Received: June 11, 2022
Accepted: November 28, 2022
Publication Date: February 9, 2023

 Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.


Download Citation: ||https://doi.org/10.6180/jase.202310_26(10).0007  


ABSTRACT

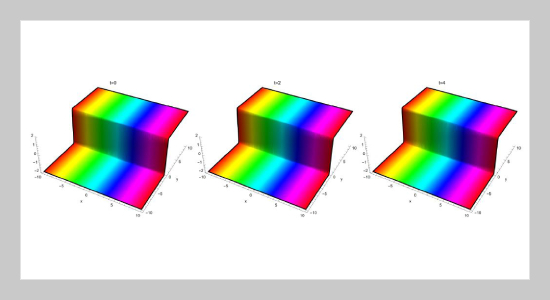
In recent decades, many applications in the literature for partial differential equations have been proposed. In this paper, we aim to determine novel wave solutions for the Calogero-Bogoyavlenskii-Schiff equation that have not been found in previous works. This equation has many applications in explaining the wave profiles in soliton theory. To reach the main results of this article, we have employed an efficient technique, namely the generalized exponential rational function method. Using the method, abundant analytical solutions are proposed that have not been obtained for the model in the existing literature. For a better description of the dynamic properties of the obtained solutions, several three-dimensional diagrams have been plotted. The results confirm that the employed technique is very simple, effective, and powerful (compare to other existing methods) for solving higher-dimensional nonlinear problems arising in mathematics, and physics. The Mathematica software has been employed to perform numerical calculations and draw diagrams.


Keywords: Analytical methods; Calogero-Bogoyavlenskii-Schiff equation; The generalized exponential rational function method; Wave solutions, Higher-order PDEs


REFERENCES

  1. [1] A. D. Polyanin and V. G. Sorokin, (2021) “A method for constructing exact solutions of nonlinear delay PDEs" Journal of Mathematical Analysis and Applications 494(2): DOI: 10.1016/j.jmaa.2020.124619.
  2. [2] B. Ghanbari and A. Atangana, (2020) “Some new edge detecting techniques based on fractional derivatives with non-local and non-singular kernels" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-02890-9.
  3. [3] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da Sociedade Paranaense de Matematica 34(2): 213–229. DOI: 10.5269/bspm.v34i2.25501.
  4. [4] S. Djilali and B. Ghanbari, (2021) “The influence of an infectious disease on a prey-predator model equipped with a fractional-order derivative" Advances in Difference Equations 2021(1): DOI: 10.1186/s13662-020-03177-9.
  5. [5] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable time fractional derivative" Calcolo 53(3): 475–485. DOI: 10.1007/s10092-015-0158-8.
  6. [6] B. Ghanbari, (2020) “A fractional system of delay differential equation with nonsingular kernels in modeling hand-foot-mouth disease" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-02993-3.
  7. [7] B. Ghanbari, (2021) “Chaotic behaviors of the prevalence of an infectious disease in a prey and predator system using fractional derivatives" Mathematical Methods in the Applied Sciences 44(13): 9998–10013. DOI: 10.1002/mma.7386.
  8. [8] B. Ghanbari, (2020) “On approximate solutions for a fractional prey-predator model involving the Atangana–Baleanu derivative" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-03140-8.
  9. [9] A. Nabti and B. Ghanbari, (2021) “Global stability analysis of a fractional SVEIR epidemic model" Mathematical Methods in the Applied Sciences 44(11): 8577–8597. DOI: 10.1002/mma.7285.
  10. [10] M. S. M. Shehata, H. Rezazadeh, E. H. M. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New Optical Soliton Solutions of the Perturbed Fokas-Lenells Equation" Communications in Theoretical Physics 71(11): 1275–1280. DOI: 10.1088/0253-6102/71/11/1275.
  11. [11] H. Rezazadeh, (2018) “New solitons solutions of the complex Ginzburg-Landau equation with Kerr law nonlinearity" Optik 167: 218–227. DOI: 10.1016/j.ijleo.2018.04.026.
  12. [12] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17): DOI: 10.1142/S0217984919501963.
  13. [13] B. Ghanbari and S. Djilali, (2020) “Mathematical and numerical analysis of a three-species predator-prey model with herd behavior and time fractional-order derivative" Mathematical Methods in the Applied Sciences 43(4): 1736–1752. DOI: 10.1002/mma.5999.
  14. [14] A. Alharbi and M. Almatrafi, (2020) “Numerical investigation of the dispersive long wave equation using an adaptive moving mesh method and its stability" Results in Physics 16: DOI: 10.1016/j.rinp.2019.102870.
  15. [15] B. Ghanbari, (2020) “On the modeling of the interaction between tumor growth and the immune system using some new fractional and fractional-fractal operators" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-03040-x.
  16. [16] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear Engineering 4(1): 15–22. DOI: 10.1515/nleng-2014-0018.
  17. [17] M. Inc, A. Yusuf, A. I. Aliyu, and D. Baleanu, (2017) “Optical soliton solutions for the higher-order dispersive cubic-quintic nonlinear Schrödinger equation" Superlattices and Microstructures 112: 164–179. DOI: 10.1016/j.spmi.2017.08.059.
  18. [18] S. T. R. Rizvi, K. Ali, and H. Hanif, (2019) “Optical solitons in dual core fibers under various nonlinearities" Modern Physics Letters B 33(17): DOI: 10.1142/S0217984919501896.
  19. [19] J. Hu and H. Zhang, (2001) “A new method for finding exact traveling wave solutions to nonlinear partial differential equations" Physics Letters, Section A: General, Atomic and Solid State Physics 286(2-3): 175–179. DOI: 10.1016/S0375-9601(01)00291-2.
  20. [20] L. Wazzan, (2009) “A modified tanh–coth method for solving the KdV and the KdV–Burgers’ equations" Communications in Nonlinear Science and Numerical Simulation 14(2): 443–450.
  21. [21] B. Ghanbari and D. Baleanu, (2019) “A novel technique to construct exact solutions for nonlinear partial differential equations" European Physical Journal Plus 134(10): DOI: 10.1140/epjp/i2019-13037-9.
  22. [22] N. Sajid and G. Akram, (2020) “Novel solutions of Biswas-Arshed equation by newly ϕ6-model expansion method" Optik 211: DOI: 10.1016/j.ijleo.2020.164564.
  23. [23] B. Ghanbari, S. Kumar, M. Niwas, and D. Baleanu, (2021) “The Lie symmetry analysis and exact Jacobi elliptic solutions for the Kawahara–KdV type equations" Results in Physics 23: DOI: 10.1016/j.rinp.2021.104006.
  24. [24] H. Kim and R. Sakthivel, (2012) “New exact traveling wave solutions of some nonlinear higher-dimensional physical models" Reports on Mathematical Physics 70(1): 39–50.
  25. [25] Y. Li, D. Lu, M. Arshad, and X. Xu, (2021) “New exact traveling wave solutions of the unstable nonlinear Schrödinger equations and their applications" Optik 226: DOI: 10.1016/j.ijleo.2020.165386.
  26. [26] V. S. Erturk and P. Kumar, (2020) “Solution of a COVID-19 model via new generalized Caputo-type fractional derivatives" Chaos, Solitons and Fractals 139: DOI: 10.1016/j.chaos.2020.110280.
  27. [27] B. Ghanbari, M. Inc, and L. Rada, (2019) “Solitary wave solutions to the tzitzéica type equations obtained by a new efficient approach" Journal of Applied Analysis and Computation 9(2): 568–589. DOI: 10.11948/2156-907X.20180103.
  28. [28] R. H. Goodman, P. J. Holmes, and M. I. Weinstein, (2004) “Strong NLS soliton-defect interactions" Physica D: Nonlinear Phenomena 192(3-4): 215–248. DOI: 10.1016/j.physd.2004.01.021.
  29. [29] B. Ghanbari, (2021) “Abundant exact solutions to a generalized nonlinear Schrödinger equation with local fractional derivative" Mathematical Methods in the Applied Sciences 44(11): 8759–8774. DOI: 10.1002/mma.7302.
  30. [30] B. Ghanbari, (2022) “On the nondifferentiable exact solutions to Schamel’s equation with local fractional derivative on Cantor sets" Numerical Methods for Partial Differential Equations 38(5): 1255–1270. DOI: 10.1002/num.22740.
  31. [31] I. Herron, C. McCalla, and R. Mickens, (2020) “Traveling wave solutions of Burgers’ equation with time delay" Applied Mathematics Letters 107: DOI: 10.1016/j.aml.2020.106496.
  32. [32] B. Ghanbari, (2021) “On novel nondifferentiable exact solutions to local fractional Gardner’s equation using an effective technique" Mathematical Methods in the Applied Sciences 44(6): 4673–4685. DOI: 10.1002/mma.7060.
  33. [33] K. Munusamy, C. Ravichandran, K. S. Nisar, and B. Ghanbari, (2020) “Existence of solutions for some functional integrodifferential equations with nonlocal conditions" Mathematical Methods in the Applied Sciences 43(17): 10319–10331. DOI: 10.1002/mma.6698.
  34. [34] B. Ghanbari, K. S. Nisar, and M. Aldhaifallah, (2020) “Abundant solitary wave solutions to an extended nonlinear Schrödinger’s equation with conformable derivative using an efficient integration method" Advances in Difference Equations 2020(1): DOI: 10.1186/s13662-020-02787-7.
  35. [35] M. Goyal, H. Baskonus, and A. Prakash, (2020) “Regarding new positive, bounded and convergent numerical solution of nonlinear time fractional HIV/AIDS transmission model" Chaos, Solitons & Fractals 2020(139:110096):
  36. [36] B. Ghanbari, A. Yusuf, M. Inc, and D. Baleanu, (2019) “The new exact solitary wave solutions and stability analysis for the (2 + 1 ) -dimensional Zakharov–Kuznetsov equation" Advances in Difference Equations 2019(1): DOI: 10.1186/s13662-019-1964-0.
  37. [37] N. A. Kudryashov, (2020) “Traveling wave solutions of the generalized Gerdjikov–Ivanov equation" Optik 219: DOI: 10.1016/j.ijleo.2020.165193.
  38. [38] K. Ayub, M. Y. Khan, and Q. Mahmood-Ul-Hassan, (2017) “Solitary and periodic wave solutions of Calogero–Bogoyavlenskii–Schiff equation via exp-function methods" Computers and Mathematics with Applications 74(12): 3231–3241. DOI: 10.1016/j.camwa.2017.08.021.
  39. [39] M. Bruzón, M. Gandarias, C. Muriel, C. Ramírez, S. Saez, and F. Romero, (2003) “The Calogero-Bogoyavlenskii-Schiff equation in 2+1 dimensions" Theoretical and Mathematical Physics 137(1): 1367–1377. DOI: 10.1023/A:1026040319977.
  40. [40] Y.-Z. Peng, (2006) “New types of localized coherent structures in the Bogoyavlenskii-Schiff equation" International Journal of Theoretical Physics 45(9): 1779–1783. DOI: 10.1007/s10773-006-9139-7.
  41. [41] B. Li, Y. Chen, H. Xuan, and H. Zhang, (2003) “Symbolic computation and construction of soliton-like solutions for a breaking soliton equation" Chaos, Solitons and Fractals 17(5): 885–893. DOI: 10.1016/S0960-0779(02)00570-2.
  42. [42] S.-T. Chen and W.-X. Ma, (2018) “Lump solutions of a generalized Calogero–Bogoyavlenskii–Schiff equation" Computers & Mathematics with Applications 76(7): 1680–1685.
  43. [43] M. Bruzón, M. Gandarias, C. Muriel, C. Ramírez, S. Saez, and F. Romero, (2003) “The Calogero-Bogoyavlenskii-Schiff equation in 2+1 dimensions" Theoretical and Mathematical Physics 137(1): 1367–1377. DOI: 10.1023/A:1026040319977.
  44. [44] Z. Hammouch, T. Mekkaoui, and P. Agarwal, (2018) “Optical solitons for the Calogero-Bogoyavlenskii-Schiff equation in (2+1) dimensions with time-fractional conformable derivative" The European Physical Journal Plus 133(7): 1–6.
  45. [45] B. Ghanbari and M. Inc, (2018) “A new generalized exponential rational function method to find exact special solutions for the resonance nonlinear Schrödinger equation" European Physical Journal Plus 133(4): DOI: 10.1140/epjp/i2018-11984-1.
  46. [46] B. Ghanbari and C.-K. Kuo, (2021) “Abundant wave solutions to two novel KP-like equations using an effective integration method" Physica Scripta 96(4): DOI: 10.1088/1402-4896/abde5a.
  47. [47] W. Gao, B. Ghanbari, H. Günerhan, and H. M. Baskonus, (2020) “Some mixed trigonometric complex soliton solutions to the perturbed nonlinear Schrödinger equation" Modern Physics Letters B 34(3): DOI: 10.1142/S0217984920500347.
  48. [48] B. Ghanbari and J. Gómez-Aguilar, (2019) “Optical soliton solutions for the nonlinear Radhakrishnan-Kundu-Lakshmanan equation" Modern Physics Letters B 33(32): DOI: 10.1142/S0217984919504025.
  49. [49] B. Ghanbari and J. Gómez-Aguilar, (2019) “New exact optical soliton solutions for nonlinear Schrödinger equation with second-order spatio-temporal dispersion involving M-derivative" Modern Physics Letters B 33(20):1950235.
  50. [50] Z. Pinar and T. Özi¸s, (2015) “Observations on the class of "Balancing Principle" for nonlinear PDEs that can be treated by the auxiliary equation method" Nonlinear Analysis: Real World Applications 23: 9–16. DOI: 10.1016/j.nonrwa.2014.11.001.
  51. [51] M. M. Khater, M. S. Mohamed, and R. A. Attia, (2021) “On semi analytical and numerical simulations for a mathematical biological model; the time-fractional nonlinear Kolmogorov–Petrovskii–Piskunov (KPP) equation" Chaos, Solitons & Fractals 144: 110676.
  52. [52] W. Li, L. Akinyemi, D. Lu, and M. M. A. Khater, (2021) “Abundant traveling wave and numerical solutions of weakly dispersive long waves model" Symmetry 13(6): DOI: 10.3390/sym13061085.
  53. [53] S. Sahoo and S. Saha Ray, (2020) “Invariant analysis with conservation law of time fractional coupled Ablowitz–Kaup–Newell–Segur equations in water waves" Waves in Random and Complex Media 30(3): 530–543. DOI: 10.1080/17455030.2018.1540899.
  54. [54] A. Tripathy and S. Sahoo, (2021) “A novel analytical method for solving (2+ 1)-dimensional extended Calogero-Bogoyavlenskii-Schiff equation in plasma physics" Journal of Ocean Engineering and Science 6(4): 405–409.
  55. [55] Z. P. Izgi, F. N. Saglam, S. Sahoo, H. Rezazadeh, and L. Akinyemi, (2022) “A partial offloading algorithm based on intelligent sensing" International Journal of Modern Physics B 36(17): DOI: 10.1142/S0217979222500977.
  56. [56] S. Sahoo and A. Tripathy, (2022) “New exact solitary solutions of the (2+1)-dimensional Heisenberg ferromagnetic spin chain equation" European Physical Journal Plus 137(3): DOI: 10.1140/epjp/s13360-022-02609-7.
  57. [57] S. Sahoo and S. Saha Ray, (2019) “On the conservation laws and invariant analysis for time-fractional coupled Fitzhugh-Nagumo equations using the Lie symmetry analysis" European Physical Journal Plus 134(2): DOI: 10.1140/epjp/i2019-12440-6.
  58. [58] S. Sahoo and S. Saha Ray, (2019) “A novel approach for stochastic solutions of wick-type stochastic time-fractional Benjamin–Bona–Mahony equation for modeling long surface gravity waves of small amplitude" Stochastic Analysis and Applications 37(3): 377–387.
  59. [59] S. Sahoo, S. Saha Ray, and S. Das, (2017) “An efficient and novel technique for solving continuously variable fractional order mass-spring-damping system" Engineering Computations (Swansea, Wales) 34(8): 2815–2835. DOI: 10.1108/EC-04-2016-0145.
  60. [60] Y. Chu, M. M. A. Khater, and Y. Hamed, (2021) “Diverse novel analytical and semi-analytical wave solutions of the generalized (2+1)-dimensional shallow water waves model" AIP Advances 11(1): DOI: 10.1063/5.0036261.
  61. [61] M. M. Khater, A. E.-S. Ahmed, and M. El-Shorbagy, (2021) “Abundant stable computational solutions of Atangana–Baleanu fractional nonlinear HIV-1 infection of CD4+ T-cells of immunodeficiency syndrome" Results in Physics 22: DOI: 10.1016/j.rinp.2021.103890.
  62. [62] M. M. Khater, A. E.-S. Ahmed, S. Alfalqi, J. Alzaidi, S. Elbendary, and A. M. Alabdali, (2021) “Computational and approximate solutions of complex nonlinear Fokas–Lenells equation arising in optical fiber" Results in Physics 25: DOI: 10.1016/j.rinp.2021.104322.
  63. [63] M. M. A. Khater, K. S. Nisar, and M. S. Mohamed, (2021) “Numerical investigation for the fractional nonlinear space-time telegraph equation via the trigonometric Quintic B-spline scheme" Mathematical Methods in the Applied Sciences 44(6): 4598–4606. DOI: 10.1002/mma.7052.
  64. [64] M. M. A. Khater and B. Ghanbari, (2021) “On the solitary wave solutions and physical characterization of gas diffusion in a homogeneous medium via some efficient techniques" European Physical Journal Plus 136(4): DOI: 10.1140/epjp/s13360-021-01457-1.
  65. [65] M. M. A. Khater, M. S. Mohamed, and S. Elagan, (2021) “Diverse accurate computational solutions of the nonlinear Klein–Fock–Gordon equation" Results in Physics 23: DOI: 10.1016/j.rinp.2021.104003.
  66. [66] M. M. A. Khater, A. Bekir, D. Lu, and R. A. M. Attia, (2021) “Analytical and semi-analytical solutions for timefractional Cahn–Allen equation" Mathematical Methods in the Applied Sciences 44(3): 2682–2691. DOI:10.1002/mma.6951.
  67. [67] M. M. A. Khater and A. E.-S. Ahmed, (2021) “Strong langmuir turbulence dynamics through the trigonometric quintic and exponential b-spline schemes" AIMS Mathematics 6(6): 5896–5908. DOI: 10.3934/math.2021349.

Latest Articles

Effects of Mach Number and Magnetic Fields on Performance of MHDG in Nozzle
Effects of Mach Number and Magnetic Fields on Performance of MHDG in NozzleVolume 28, Issue 9 (In Press)

Read more: ...

Considering the Remaining Useful Life of Lithium Batteries for Modern Tram Power System Capacity Configuration
Considering the Remaining Useful Life of Lithium Batteries for Modern Tram Power System Capacity ConfigurationVolume 28, Issue 9 (In Press)

Read more: ...

Performance evaluation and sensitivity analysis of a low- capacity single-effect LiBr-H2O absorption cooling system using energy and exergy modeling
Performance evaluation and sensitivity analysis of a low- capacity single-effect LiBr-H2O absorption cooling system using energy and exergy modelingVolume 28, Issue 9 (In Press)

Read more: ...

Research on Power - load Cooperative Control of Wind Turbines Based on Variable Domain Theory
Research on Power - load Cooperative Control of Wind Turbines Based on Variable Domain TheoryVolume 28, Issue 9 (In Press)

Read more: ...

Behavior Anomaly Detection Based on Multi-modal Feature Fusion and Its Application in English Teaching
Behavior Anomaly Detection Based on Multi-modal Feature Fusion and Its Application in English TeachingVolume 28, Issue 9 (In Press)

Read more: ...

Enhanced Deep Learning Approaches for Diagnosing Drilling Machine Failures Using Gramian Angular Field and Markov Transition Field Encoding
Enhanced Deep Learning Approaches for Diagnosing Drilling Machine Failures Using Gramian Angular Field and Markov Transition Field EncodingVolume 28, Issue 9 (In Press)

Read more: ...

Sensor Image, Anomaly Detection Method for Hydroelectric Dam Structure Using Sensors Measurements and Deep Learning
Sensor Image, Anomaly Detection Method for Hydroelectric Dam Structure Using Sensors Measurements and Deep LearningVolume 28, Issue 9 (In Press)

Read more: ...

Carbon nanodots from used green tea biochar: antibacterial applications and antibiofilm carbon coatings
Carbon nanodots from used green tea biochar: antibacterial applications and antibiofilm carbon coatingsVolume 28, Issue 9 (In Press)

Read more: ...

A Prototype of a biomimetic scallop robot with bivalve propulsion and a hydrodynamic model for its velocity
A Prototype of a biomimetic scallop robot with bivalve propulsion and a hydrodynamic model for its velocityVolume 28, Issue 8

Read more: ...

    



 

2.1
2023CiteScore
 
 
69th percentile
Powered by  Scopus

SCImago Journal & Country Rank

You may also like these articles below...

Most Popular Articles

Research Categories

Enter your name and email below to receive latest published articles in Journal of Applied Science and Engineering.