Alexander Nikolaevich Belyaev1, Vladimir Pavlovich Shatsky2, Vyacheslav Gennadievich Kozlov 3, Tatiana Vladimirovna Trishina1, and Irina Alevtinovna Vysotskaya4
1Department of Applied Mechanics, Faculty of Agricultural Engineering, Voronezh State Agrarian University named after Emperor Peter the Great, 1 Michurina ave., Voronezh, 394087, Russian Federation
2Department of Mathematics and Physics, Faculty of Agricultural Engineering, Voronezh State Agrarian University named after Emperor Peter the Great, 1 Michurina ave., Voronezh, 394087, Russian Federation
3Department of Operation of Transport and Technological Machines, Faculty of Agricultural Engineering, Voronezh State Agrarian University named after Emperor Peter the Great, 1 Michurina ave., Voronezh, 394087, Russian Federation
4Department of Mathematics, Military Training and Research Center of the Air Force “Air Force Academy named after Professor N.E. Zhukovsky and Y.A. Gagarin”, 54A Bolsheviks str., Voronezh, 394064, Russian Federation
Received:
August 11, 2021
Accepted:
November 27, 2021
Publication Date:
December 17, 2021
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
Download Citation: ||https://doi.org/10.6180/jase.202210_25(5).0004
ABSTRACT
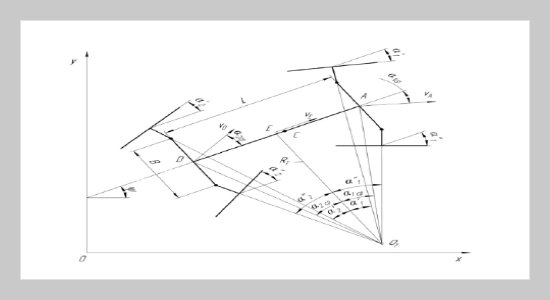
The most complete presentation of stability of curvilinear motion of wheeled vehicle is provided by variations of motion path of its kinematic center and turn coordinates: maximum abscissa and ordinate showing both transversal and longitudinal deviations of vehicle, including those determining its flexibility and capacity. The methods of motion of wheeled vehicle are selected on the basis of specific operation conditions. This article describes derivation of explicit equations for analytical prediction of theoretical coordinates of path points of wheeled vehicle kinematic center upon loop-free circulation cornering. The derivation is based on parametric equation for determination of current coordinates of theoretical curve of entering corner and circumference, as well as smoothness condition and path continuity at the sites of their junction. The proposed equations made it possible to determine theoretical path of total cycle of loop-free circulation cornering of wheeled vehicl as a function of the following parameters: design related – axle base, distance between kingpins, maximum turn angles of internal steered wheels, and operation related – vehicle forward speed, angular turning speed of steered wheels in transverse plane, as well as to analyze and to compare the most universal methods of cornering: by front steered wheel and by front and rear wheel by their turning in opposite directions with regard to frame.
Keywords:
curvilinear motion, rotation, trajectory, clothoid, vehicle
REFERENCES
- [1] A. Belyaev, V. Kozlov, I. Vysotskaya, and T. Trishina, (2019) “Computation of vehicle motion path upon entering turn" International Journal of Engineering and Advanced Technology 9(1): 4527–4531.
- [2] A. V. Skrypnikov, V. G. Kozlov, A. N. Belyaev, and E. V. Chernyshova. “Theoretical foundations of the method of designing a clothoid track with approximation of succession of points”. In: Perspectives on the use of New Information and Communication Technology (ICT) in the Modern Economy. Springer. 2017, 654–667.
- [3] I. Troyanovskaya and S. Voinash. “Model for stationary turn of an arbitrary vehicle”. In: IOP Conference Series: Materials Science and Engineering. 450.3.IOP Publishing. 2018, 032035.
- [4] R. Field and E. Hurtago, (2003) “Modeling of dynamic forces of a tractor in the MATLAB-simulink program environment" New York: Society of Automotive Engineers:
- [5] H. Itoh, A. Oida, and M. Yamazaki, (1999) “Numerical simulation of a 4WD-4WS tractor turning in a rice field" Journal of Terramechanics 36(2): 91–115. DOI: 10.1016/S0022-4898(98)00037-8.
- [6] L. Segel, (1956) “Theoretical prediction and experimental substantiation of the response of the automobile to steering control" Proceedings of the Institution of Mechanical Engineers: Automobile Division 10(1): 310–330.
- [7] C. B.Winkler, (1998) “Simplified analysis of the steadystate turning of complex vehicles" Vehicle System Dynamics 29(3): 141–180.
- [8] I. Troyanovskaya, A. Ulanov, A. Zhakov, and S. Voinash, (2019) “Friction forces at the wheel’s contact with the ground in a turning vehicle" Tribology in Industry 41(2): 166–171. DOI: 10.24874/ti.2019.41.02.03.
- [9] I. Troyanovskaya and B. Pozin, (2015) “Forces of friction at the wheel-to-ground contact in a turning vehicle" Procedia Engineering 129: 156–160.
- [10] R. M. Dreizler and C. S. Lüdde. Theoretical Mechanics: Theoretical Physics 1. Springer Science & Business Media, 2010.
- [11] T. Myint-U and L. Debnath, (2007) “Linear partial differential equations for scientists and engineers" Springer Science & Business Media:
- [12] R. N. Jazar. Vehicle dynamics: theory and application. Springer, 2017.
- [13] H. Pacejka. Tire and vehicle dynamics. Elsevier, 2005.
- [14] A. V. Aliev, O. V. Mishchenkova, and A. M. Lipanov. Mathematical modeling and numerical methods in chemical physics and mechanics. CRC Press, 2016.
- [15] J. Avellar, L. Duarte, and L. da Mota, (2012) “PSsolver: A Maple implementation to solve first order ordinary differential equations with Liouvillian solutions" Computer Physics Communications 183(10): 2313–2314. DOI:10.1016/j.cpc.2012.04.012.
- [16] M. Siddique and K. Mitchell. “Mathematical concepts and maple animations”. In: AIP Conference Proceedings. 1281. 1. American Institute of Physics. 2010,706–708.
- [17] S. Mammar and V. Baghdassarian. “Two-degree-offreedom formulation of vehicle handling improvement by active steering”. In: Proceedings of the 2000 American Control Conference. ACC (IEEE Cat. No.00CH36334). 1. 6. IEEE. 2000, 105–109.
- [18] O. Mokhiamar and M. Abe, (2002) “Active wheel steering and yaw moment control combination to maximize stability as well as vehicle responsiveness during quick lane change for active vehicle handling safety" Proceedings of the Institution of Mechanical Engineers, Part D: Journal of Automobile Engineering 216(2): 115–124.