Nurul Musfirah Murad1, Noraihan Afiqah Rawi This email address is being protected from spambots. You need JavaScript enabled to view it.1, Sharidan Shafie1, and Rahimah Mahat2
1Department of Mathematical Sciences, Faculty of Science, Universiti Teknologi Malaysia, 81310 UTM Johor Bahru, Johor, Malaysia 2Universiti Kuala Lumpur, Malaysian Institute of Industrial Technology, Persiaran Sinaran Ilmu, 81705 Masai, Johor
Received: December 7, 2020 Accepted: July 3, 2021 Publication Date: October 11, 2021
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
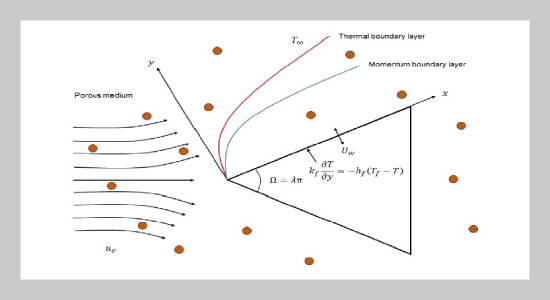
Hybrid nanofluid is known to improve heat transfer performance, and its advantages have led to relatively reasonable expectations for their applications. This research considered a moving wedge, namely the Falkner-Skan model, which is well-known in the aerodynamic field. Hybrid nanofluid has been chosen where the dispersion of alumina and copper nanoparticles with water as the base fluid is considered in the unsteady mixed convection flow over moving wedge. By using similarity transformations, the governing equations are converted into ordinary differential equations and then numerically solved using MATLAB bvp4c solver. The increasing values of porosity parameter caused the velocity of hybrid nanofluid to increase. The results also indicated that, the effect of porosity parameter improved the values of skin friction coefficient but decrease the value of Nusselt number.
Keywords: Falkner-Skan flow; Unsteady flow; Hybrid nanofluid; Porous medium
REFERENCES
[1] M. Salem Ahmed, M. R. A. Hady, and G. Abdallah, (2018) “Experimental investigation on the performance of chilled-water air conditioning unit using alumina nanofluids" Thermal Science and Engineering Progress 5: 589–596. DOI: https://doi.org/10.1016/j.tsep.2017.07.002.
[2] M. S. Ahmed and A. M. Elsaid, (2019) “Effect of hybrid and single nanofluids on the performance characteristics of chilled water air conditioning system" Applied Thermal Engineering 163: 114398. DOI: https://doi.org/10.1016/j.applthermaleng.2019.114398.
[3] J. Sarkar, P. Ghosh, and A. Adil, (2015) “A review on hybrid nanofluids: Recent research, development and applications" Renewable and Sustainable Energy Reviews 43: 164–177. DOI: https://doi.org/10.1016/j.rser.2014.11.023.
[4] N. A. L. Aladdin, N. Bachok, and I. Pop, (2020) “Cu-Al2O3/water hybrid nanofluid flow over a permeable moving surface in presence of hydromagnetic and suction effects" Alexandria Engineering Journal 59(2): 657–666. DOI: https://doi.org/10.1016/j.aej.2020.01.028.
[5] I.Waini, A. Ishak, and I. Pop, (2019) “Hybrid nanofluids flow and heat transfer over a nonlinear permeable stretching/shrinking surface" International Journal of Numerical Methods for Heat & Fluid Flow:
[6] T. Hayat and S. Nadeem, (2017) “Heat transfer enhancement with Ag–CuO/water hybrid nanofluid" Results in Physics 7: 2317–2324. DOI: https://doi.org/10.1016/j.rinp.2017.06.034.
[7] S. Ghadikolaei, M. Yassari, H. Sadeghi, K. Hosseinzadeh, and D. Ganji, (2017) “Investigation on thermophysical properties of Tio2–Cu/H2O hybrid nanofluids transport dependent on shape factor in MHD stagnation point flow" Powder Technology 322: 428–438. DOI:https://doi.org/10.1016/j.powtec.2017.09.006.
[8] B. M. Al-Srayyih, S. Gao, and S. H. Hussain, (2019) “Natural convection flow of a hybrid nanofluid in a square enclosure partially filled with a porous medium using a thermal non-equilibrium model" Physics of Fluids 31(4): 043609.
[9] M. Usman, M. Hamid, T. Zubair, R. Ul Haq, and W. Wang, (2018) “Cu-Al2O3/Water hybrid nanofluids through a permeable surface in the presence of nonlinear radiation and variable thermal conductivity via LSM" International Journal of Heat and Mass Transfer 126: 1347–1356. DOI: https://doi.org/10.1016/j.ijheatmasstransfer.2018.06.005.
[10] M. Izadi, R. Mohebbi, D. Karimi, and M. A. Sheremet, (2018) “Numerical simulation of natural convection heat transfer inside a ? shaped cavity filled by a MWCNTFe3O4/water hybrid nanofluids using LBM" Chemical Engineering and Processing - Process Intensification 125: 56–66. DOI: https://doi.org/10.1016/j.cep.2018.01.004.
[11] M. Ghalambaz, M. A. Sheremet, S. Mehryan, F. M. Kashkooli, and I. Pop, (2019) “Local thermal non-equilibrium analysis of conjugate free convection within a porous enclosure occupied with Ag–MgO hybrid nanofluid" Journal of Thermal Analysis and Calorimetry 135(2): 1381–1398.
[12] M. Adil Sadiq and T. Hayat, (2016) “Darcy–Forchheimer flow of magneto Maxwell liquid bounded by convectively heated sheet" Results in Physics 6: 884–890. DOI: https://doi.org/10.1016/j.rinp.2016.10.019.
[13] J. Umavathi, O. Ojjela, and K. Vajravelu, (2017) “Numerical analysis of natural convective flow and heat transfer of nanofluids in a vertical rectangular duct using Darcy-Forchheimer-Brinkman model" International Journal of Thermal Sciences 111: 511–524. DOI: https://doi.org/10.1016/j.ijthermalsci.2016.10.002.
[14] T. Hayat, A. Aziz, T. Muhammad, and A. Alsaedi, (2018) “An optimal analysis for Darcy–Forchheimer 3D flow of nanofluid with convective condition and homogeneous–heterogeneous reactions" Physics Letters A 382(39): 2846–2855. DOI: https://doi.org/10.1016/j.physleta.2018.06.015.
[15] A. K. Alzahrani, (2018) “Darcy–Forchheimer 3D flow of carbon nanotubes with homogeneous and heterogeneous reactions" Physics Letters A 382(38): 2787–2793. DOI:https://doi.org/10.1016/j.physleta.2018.06.011.
[16] Z. Shah, A. Dawar, S. Islam, I. Khan, and D. L. C. Ching, (2018) “Darcy-Forchheimer flow of radiative carbon nanotubes with microstructure and inertial characteristics in the rotating frame" Case Studies in Thermal Engineering 12: 823–832. DOI: https://doi.org/10.1016/j.csite.2018.09.007.
[17] B. Chandrasekhara and P. Namboodiri, (1985) “Influence of variable permeability on combined free and forced convection about inclined surfaces in porous media" International Journal of Heat and Mass Transfer 28(1): 199–206. DOI: https://doi.org/10.1016/0017-9310(85)90022-5.
[18] D. Rees and I. Pop, (2000) “Vertical free convection in a porous medium with variable permeability effects" International Journal of Heat and Mass Transfer 43(14): 2565–2571. DOI: https://doi.org/10.1016/S0017-9310(99)00316-6.
[19] R. S. Saif, T. Muhammad, and H. Sadia, (2020) “Significance of inclined magnetic field in Darcy–Forchheimer flow with variable porosity and thermal conductivity" Physica A: Statistical Mechanics and its Applications 551: 124067. DOI: https://doi.org/10.1016/j.physa.2019.124067.
[20] V. Falkner and S. Skan, (1931) “Some approximate solutions of the boundary-layer for flow past a stretching boundary" SIAM J Appl Math 49: 1350–1358.
[21] T. Watanabe, (1990) “Thermal boundary layers over a wedge with uniform suction or injection in forced flow" Acta Mechanica 83(3): 119–126.
[22] M. Kumari, H. S. Takhar, and G. Nath, (2001) “Mixed convection flow over a vertical wedge embedded in a highly porous medium" Heat and Mass Transfer 37(2): 139–146.
[23] A. Ishak, R. Nazar, and I. Pop, (2007) “Falkner-Skan equation for flow past a moving wedge with suction or injection" Journal of Applied Mathematics and Computing 25(1): 67–83.
[24] M. Ganapathirao, R. Ravindran, and E. Momoniat, (2015) “Effects of chemical reaction, heat and mass transfer on an unsteady mixed convection boundary layer flow over a wedge with heat generation/absorption in the presence of suction or injection" Heat and Mass Transfer 51(2): 289–300.
[25] I. Ullah, I. Khan, and S. Shafie, (2016) “Hydromagnetic Falkner-Skan flow of Casson fluid past a moving wedge with heat transfer" Alexandria Engineering Journal 55(3): 2139–2148. DOI: https://doi.org/10.1016/j.aej.2016.06.023.
[26] I. Ullah, S. Shafie, O. D. Makinde, and I. Khan, (2017) “Unsteady MHD Falkner-Skan flow of Casson nanofluids with generative/destructive chemical reaction" Chemical Engineering Science 172: 694–706. DOI: https://doi.org/10.1016/j.ces.2017.07.011.
[27] I. Ullah, S. Shafie, and I. Khan, (2017) “MHD Mixed convection flow of Casson fluid over a moving wedge saturated in a porous medium in the presence of chemical reaction and convective boundary conditions" Journal of Science and Technology 9(3):
We use cookies on this website to personalize content to improve your user experience and analyze our traffic. By using this site you agree to its use of cookies.
