Calculation of the energy levels and wave functions of electrons in nanowires by the shooting method

G. Gulyamov; A. B. Davlatov; S. T. Inoyatov; S. A. Makhmudov

doi:10.6180/jase.202202_25(1).0004

Calculation of the energy levels and wave functions of electrons in nanowires by the shooting method

Physics

G. Gulyamov¹, A.B. Davlatov This email address is being protected from spambots. You need JavaScript enabled to view it.², S.T. Inoyatov³, and S.A. Makhmudov³

¹Namangan Engineering Construction Institute, 12 Islam Karimov Street, Namangan 160103, Uzbekistan
²Physical-Technical Institute, Uzbek Academy of Sciences, Chingiz Aytmatov Street, 2 “B”, Tashkent 100084, Uzbekistan
³Namangan State University,316 Uychi Street, Namangan 160136, Uzbekistan

Received: April 1, 2021
Accepted: June 14, 2021
Publication Date: July 6, 2021

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202202_25(1).0004

ABSTRACT

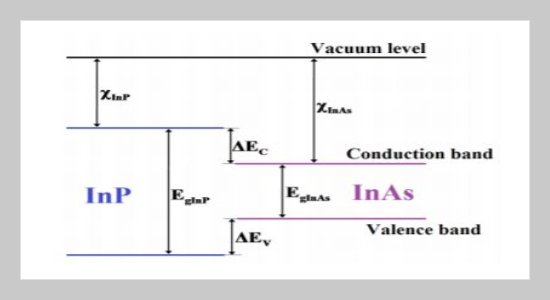
In this work, the energy levels and wave functions in rectangular and cylindrical nanowires with a finite potential well are calculated. The Schrödinger equation in Cartesian and cylindrical coordinate systems was solved by the shooting method. The calculations take into account the nonparabolicity of the energy spectrum of electrons. The graphs of the dependence of the energy levels on the sizes of nanowires are obtained. When calculating the energy levels and wave functions, changes in the effective mass of electrons were taken into account. The calculations were performed for the quantum well of the InP/InAs/InP heterostructure.

Keywords: Nanowires, Quantum wells, Heterostructure, Energy levels, Wave function, Nonparabolic zone

REFERENCES

[1] P. Mohan, J. Motohisa, and T. Fukui, (2005) “Controlled growth of highly uniform, axial/radial directiondefined, individually addressable InP nanowire arrays" Nanotechnology 16(12): 2903–2907. DOI: 10.1088/0957-4484/16/12/029.
[2] J. Motohisa, J. Noborisaka, J. Takeda, M. Inari, and T. Fukui, (2004) “Catalyst-free selective-area MOVPE of semiconductor nanowires on (111) B oriented substrates" Journal of crystal growth 272(1-4): 180–185. DOI: 10.1016/j.jcrysgro.2004.08.118.
[3] F. Dirnberger, M. Kammermeier, J. König, M. Forsch, P. E. Faria Junior, T. Campos, J. Fabian, J. Schliemann, C. Schüller, and T. Korn, (2019) “Ultralong spin lifetimes in one-dimensional semiconductor nanowires" Applied Physics Letters 114(20): 202101. DOI: 10.1063/1.5096970.
[4] G. Gulyamov, A. G. Gulyamov, A. B. Davlatov, and B. B. Shahobiddinov, (2020) “Electron energy in rectangular and cylindrical quantum wires" Journal of Nanoand Electronic Physics 12(4): DOI: 10.21272/jnep. 12(4).04023.
[5] A. Bouazra, S. A.-B. Nasrallah, A. Poncet, Y. Bouazra, and M. Said, (2009) “Numerical simulation of coupling effect on electronic states in quantum wires" The European Physical Journal B 67(2): 245–250.
[6] A. Esposito, M. Luisier, M. Frey, and A. Schenk, (2009) “A nonparabolicity model compared to tightbinding: The case of square silicon quantum wires" Solidstate electronics 53(3): 376–382. DOI: 10.1016/j.sse. 2009.01.012.
[7] F. M. S. Lima, O. A. C. Nunes, A. L. A. Fonseca, M. A. Amato, and E. F. Da Silva, (2008) “Effect of a terahertz laser field on the electron-DOS in a GaAs/AlGaAs cylindrical quantum wire: finite well model" Semiconductor Science and Technology 23(12): 125038. DOI:10.1088/0268-1242/23/12/125038.
[8] A. Radu, (2012) “Transverse laser dressing effects on the subband density of states in a 20-nm-wide GaAs/Al0. 3Ga0. 7As quantum well wire" Physica E: Low- Dimensional Systems and Nanostructures 44(7-8): 1446–1453. DOI: 10.1016/j.physe.2012.03.009.
[9] M. Tsetseri and G. P. Triberis, (2002) “A study of the ground state of quantum wires using the finite difference method" Superlattices and microstructures 32(1): 79–90. DOI: 10.1006/spmi.2002.1060.
[10] P. Harrison. QuantumWells,Wires and Dots: Theoretical and Computational Physics of Semiconductor Nanostructures. Ed. by John Wiley. 4th. 2005, 624. DOI: 10.1002/0470010827.
[11] G. Gulyamov, A. G. Gulyamov, A. B. Davlatov, and K. N. Juraev, (2020) “Energy Levels in Nanowires and Nanorods with a Finite Potential Well" Advances in Condensed Matter Physics 2020: DOI: 10 . 1155 /2020/4945080.
[12] J. H. Davies. The physics of low-dimensional semiconductors: an introduction. Cambridge university press, 1998.
[13] Q. Gao, V. G. Dubrovskii, P. Caroff, J. Wong- Leung, L. Li, Y. Guo, L. Fu, H. H. Tan, and C. Jagadish, (2016) “Simultaneous selective-area and vapor– liquid–solid growth of InP nanowire arrays" Nano letters 16(7): 4361–4367. DOI: 10.1021/acs.nanolett. 6b01461.
[14] E. O. Kane, (1957) “Band structure of indium antimonide" Journal of Physics and Chemistry of Solids 1(4): 249–261. DOI: 10.1016/0022-3697(57)90013-6.
[15] P. J. Baymatov and B. T. Abdulazizov, (2017) “Concentration dependences of the electron effective mass, fermi energy, and filling of subbands in doped InAs/AlSb quantum wells" Ukrainian Journal of Physics 62(1): 46.DOI: 10.15407/ujpe62.01.0046.
[16] G. Gulyamov, U. I. Erkaboev, and A. G. Gulyamov, (2017) “Influence of pressure on the temperature dependence of quantum oscillation phenomena in semiconductors" Advances in condensed matter physics 2017: DOI: 10.1155/2017/6747853.
[17] J. Robertson and B. Falabretti, (2006) “Band offsets of high K gate oxides on III-V semiconductors" Materials Science and Engineering B: Solid-State Materials for Advanced Technology 135(3): 267–271. DOI: 10.1016/j.mseb.2006.08.017.
[18] S. Saravanan, A. J. Peter, and C. W. Lee, (2016) “Phonon effects on interband optical transitions in InAs0. 8P0. 2/InP quantum wire" Journal of Luminescence 169: 86–92. DOI: 10.1016/j.jlumin.2015.08.043.
[19] R. Leonelli, C. A. Tran, J. L. Brebner, J. T. Graham, R. Tabti, R. A. Masut, and S. Charbonneau, (1993) “Optical and structural properties of metalorganic-vaporphase- epitaxy-grown InAs quantum wells and quantum dots in InP" Physical Review B 48(15): 11135–11143.DOI: 10.1103/PhysRevB.48.11135.
[20] P. R. C. Kent, G. L. W. Hart, and A. Zunger, (2002) “Biaxial strain-modified valence and conduction band offsets of zinc-blende GaN, GaP, GaAs, InN, InP, and InAs, and optical bowing of strained epitaxial InGaN alloys" Applied physics letters 81(23): 4377–4379. DOI: 10. 1063/1.1524299.