A novel approach for solving partial integro-differential equations using optimal perturbation iteration method

Noor S.  Rahmah; Osama H.  Mohammed

doi:10.6180/jase.202510_28(10).0002

A novel approach for solving partial integro-differential equations using optimal perturbation iteration method

Noor S. Rahmah¹This email address is being protected from spambots. You need JavaScript enabled to view it. and Osama H. Mohammed²

¹Department of Mathematics and Computer Applications, College of Science, Al-Nahrain University, Jadriya, Baghdad, Iraq

²Department of Mathematics and Computer Applications, College of Science, Al-Nahrain University, Jadriya, Baghdad, Iraq

Received: September 29, 2024
Accepted: November 25, 2024
Publication Date: January 13, 2025

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202510_28(10).0002

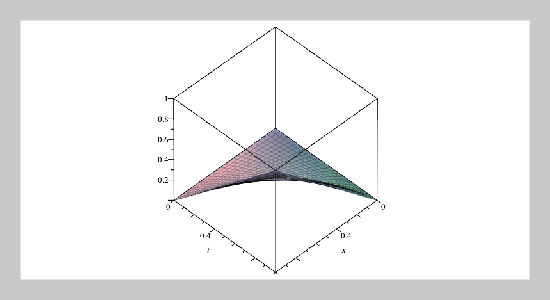
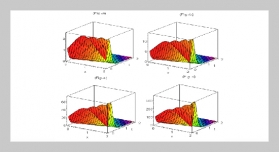
We present a novel and effective algorithm to solve non-linear fractional order partial integro-differential equations whichisbasedontheoptimalperturbationiteration transform method. Thefractionalorderderivative will be in the Caputo-Atangana-Baleanu sense. The optimal perturbation iteration transform method is a combination between the Laplace transform and the perturbation iteration algorithm. The solution is described by the suggested method as a quickly convergent series. To demonstrates the applicability of the proposed technique, two model examples are solved. The present paper also unveils that optimal perturbation iteration transform method quickly converges to the precise answers of the provided equations at a reduced iteration value.

Keywords: Perturbation iteration method, Partial integro-differential equations, Fractional calculus, Least squares method.

[1] E.Bazhlekova, (2001) “Fractional evolution equations in Banach spaces" Technische Universiteit Eindhoven: DOI: 10.6100/IR549476.
[2] K. Diethelm and N. J. Ford, (2002) “Analysis of frac tional differential equations" Journal of Mathemati cal Analysis and Applications 265(2): 229–248. DOI: 10.1006/jmaa.2000.7194.
[3] R.Hilfer. Applications of fractional calculus in physics. World scientific, 2000.
[4] A.A.Kilbas,H.M.Srivastava,andJ.J.Trujillo. Theory and applications of fractional differential equations.204.elsevier, 2006.
[5] M.Kosti´ c, (2020) “Abstract Degenerate Volterra Integro Differential Equations" Mathematical Institute SANU: Belgrade, Serbia:
[6] I. Podlubny, (1999) “Fractional-order systems and PI/sup/spl lambda//D/sup/spl mu//-controllers" IEEE Transactions on automatic control 44(1): 208–214.
[7] S. G. Samko, A. A. Kilbas, and O. I. Marichev. Inte grals and derivatives of fractional order and some of their applications. 1987.
[8] Y. Zhou. Basic theory of fractional differential equations. World scientific, 2023.
[9] B.Senol, A. Ates, B. B. Alagoz, and C. Yeroglu, (2014) “Anumericalinvestigation for robust stability of fractional order uncertain systems" ISA transactions 53(2): 189 198. DOI: 10.1016/j.isatra.2013.09.004.
[10] O. Heaviside. Electromagnetic theory. 237. American Mathematical Soc., 2003.
[11] Y. Zhaosheng and L. Jianzhong, (1998) “Numerical re search on the coherent structure in the viscoelastic second order mixing layers" Applied Mathematics and Me chanics 19(8): 717–723.
[12] K. B. Oldham, (2010) “Fractional differential equations in electrochemistry" Advances in Engineering soft ware 41(1): 9–12. DOI: 10.1016/j.advengsoft.2008.12.012.
[13] V. Lakshmikantham. Theory of integro-differential equa tions. 1. CRC press, 1995.
[14] E. G. Yanik and G. Fairweather, (1988) “Finite ele ment methods for parabolic and hyperbolic partial integro differential equations" Nonlinear Analysis: Theory, Methods & Applications 12(8): 785–809. DOI: 10. 1016/0362-546X(88)90039-9.
[15] F. Maimardi. Fractional calculus and waves in linear viscoelasticity. 2010.
[16] G. M.Zaslavsky. Hamiltonian chaos and fractional dy namics. Oxford University Press, USA, 2005.
[17] I. Podlubny. Fractional differential equations: an intro duction to fractional derivatives, fractional differential equations, to methods of their solution and some of their applications. elsevier, 1998.
[18] A.A.Kilbas,H.M.Srivastava,andJ.J.Trujillo.Theory and applications of fractional differential equations. 204. elsevier, 2006.
[19] H. Jaradat, F. Awawdeh, and E. Rawashdeh, (2011) “Analytic solution of fractional integro-differential equations" Annals of the University of Craiova Mathematics and Computer Science Series 38(1): 1–10. DOI: 10.52846/ami.v38i1.389.
[20] H. Dehestani, Y. Ordokhani, and M. Razzaghi, (2020) “Numerical solution of variable-order time fractional weakly singular partial integro-differential equations with error estimation" Mathematical Modelling and Anal ysis 25(4): 680–701. DOI: 10.3846/mma.2020.11692.
[21] A. H.Nayfeh. Introduction to perturbation techniques. John Wiley & Sons, 2011.
[22] J.-H. He, (2003) “Linearized perturbation technique and its applications to strongly nonlinear oscillators" Com puters & Mathematics with Applications 45(1-3): 1–8. DOI: 10.1016/S0898-1221(03)80002-0.
[23] J.-H. He, (2003) “Homotopy perturbation method: a new nonlinear analytical technique" Applied Mathematics and computation 135(1): 73–79. DOI: 10.1016/S0096-3003(01)00312-5.
[24] H.F. MohammedandO.H.Mohammed,(2024)“A hybrid technique for solving fractional delay variational problems by the shifted Legendre polynomials" Partial Differential Equations in Applied Mathematics 9: 100635. DOI: 10.1016/j.padiff.2024.100635.
[25] A. K. Farhood and O. H. Mohammed, (2023) “Shifted Chebyshev operational matrices to solve the fractional time-delay diffusion equation" Partial Differential Equations in Applied Mathematics 8: 100538. DOI: 10.1016/j.padiff.2023.100538.
[26] N. A. Abdulhameed, O. H. Mohammed, and A. A. Yousif, (2023) “A hybrid technique for approximating the solution of fractional order integro differential equations" Partial Differential Equations in Applied Mathe matics 8: 100552. DOI: 10.1016/j.padiff.2023.100552.
[27] H.Srivastava, S. Deniz, and K. M. Saad, (2021) “An efficient semi-analytical method for solving the generalized regularized long wave equations with a new fractional derivative operator" Journal of King Saud University Science 33(2): 101345. DOI: 10.1016/j.jksus.2021.101345.
[28] S. Deniz and N. Bildik. “Applications of optimal perturbation iteration method for solving nonlinear differential equations”. In: AIP Conference Proceed ings. 1798. 1. AIP Publishing. 2017. DOI: 10.1063/1.4972638.
[29] S. Deniz and N. Bildik, (2017) “A new analytical tech nique for solving Lane-Emden type equations arising in astrophysics" Bulletin of the Belgian Mathematical Society-Simon Stevin 24(2): 305–320. DOI: 10.36045/bbms/1503453712.
[30] S. Deniz and N. Bildik, (2018) “Optimal perturbation iteration method for Bratu-type problems" Journal of King Saud University-Science 30(1): 91–99. DOI: 10.1016/j.jksus.2016.09.001.