Analytical Approach For Time-Conformable Schrödinger Equations

Xinling  Dong; Yuanyuan  Chen

doi:10.6180/jase.202310_26(10).0010

Analytical Approach For Time-Conformable Schrödinger Equations

Physics

Xinling Dong^1,2 and Yuanyuan Chen³

¹Hainan Vocational University of Science and Technology, Haikou 571126, Hainan, China
²College of Humanities and Marxism, Hebei Oriental University, Langfang 065001, Hebei, China
³School of Foreign Languages Studies, University of Science and Technology Liaoning, Anshan 114051, Liaoning, China

Received: May 1, 2022
Accepted: July 23, 2022
Publication Date: February 9, 2023

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202310_26(10).0010

ABSTRACT

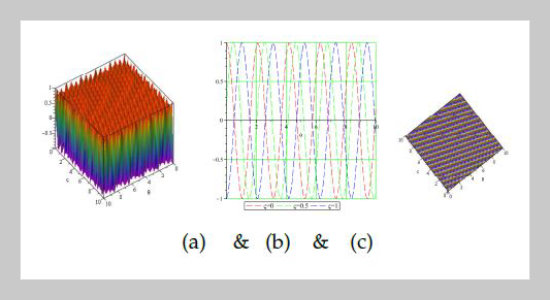
In this paper, we introduce a new technic for solving the conformable Schrödinger equations. The Schrödinger equation is an equation that describes how the quantum state of a physical system changes in time. The conformable derivatives are considered in Khalil sense. The proposed technic is based on conformable Laplace transform (CLT) and new homotopy perturbation technic (NHPT). Two examples are provided to illustrate the reliability and capability of the technique. We show some graphs to explain these solutions. The results obtained with the proposed method show that this approach is very simple, efficient and can be applied to other conformable differential equations

Keywords: Schrödinger Equation; NHPT; CLT.

REFERENCES

[1] R. Ellahi, S. T. Mohyud-Din, U. Khan, et al., (2018) “Exact traveling wave solutions of fractional order Boussinesq-like equations by applying Exp-function method" Results in physics 8: 114–120. DOI: 10.1016/j.rinp.2017.11.023.
[2] M. Mamat, S. Syouri, I. M. Alghrouz, I. M. Sulaiman, S. F. Sufahani, and P. Quds, (2020) “Conformable fractional differential transform method for solving fractional derivatives" Int. J. Adv. Sci. Technol 29: 1734–1743.
[3] L. Song and W. Wang, (2013) “A new improved Adomian decomposition method and its application to fractional differential equations" Applied Mathematical Modelling 37(3): 1590–1598. DOI: 10.1016/j.apm.2012.03.016.
[4] Q. Feng, (2018) “A new approach for seeking coefficient function solutions of conformable fractional partial differential equations based on the Jacobi elliptic equation" Chinese Journal of Physics 56(6): 2817–2828. DOI:10.1016/j.cjph.2018.08.006.
[5] A. Bhrawy, M. Tharwat, and A. Yildirim, (2013) “A new formula for fractional integrals of Chebyshev polynomials: Application for solving multi-term fractional differential equations" Applied Mathematical Modelling 37(6): 4245–4252. DOI: 10.1016/j.apm.2012.08.022.
[6] M. Senol, L. Akinyemi, A. Ata, and O. S. Iyiola, (2021) “Approximate and generalized solutions of conformable type Coudrey–Dodd–Gibbon–Sawada–Kotera equation" International Journal of Modern Physics B 35(02): 2150021. DOI: 10.1142/S0217979221500211.
[7] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear engineering 4(1): 15–22. DOI: 10.1515/nleng-2014-0018.
[8] O. A. Arqub and A. El-Ajou, (2013) “Solution of the fractional epidemic model by homotopy analysis method" Journal of King Saud University-Science 25(1): 73–81. DOI: 10.1016/j.jksus.2012.01.003.
[9] B. Ghanbari, K. S. Nisar, and M. Aldhaifallah, (2020) “Abundant solitary wave solutions to an extended nonlinear Schrodinger’s equation with conformable derivative using an efficient integration method" Advances in Difference Equations 2020(1): 1–25. DOI: 10.1186/s13662-020-02787-7.
[10] H. Aminikhah, A. H. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da sociedade paranaense de matemática 34(2): 213–229. DOI: 10.5269/bspm.v34i2.25501.
[11] M. S. Hashemi, M. Inc, and A. Yusuf, (2020) “On three-dimensional variable order time fractional chaotic system with nonsingular kernel" Chaos, Solitons & Fractals 133: 109628. DOI: 10.1016/j.chaos.2020.109628.
[12] Y. Salehi, M. T. Darvishi, andW. E. Schiesser, (2018) “Numerical solution of space fractional diffusion equation by the method of lines and splines" Applied Mathematics and Computation 336: 465–480. DOI: 10.1016/j.amc.2018.04.053.
[13] W. Satsanit, (2020) “On the solution of Wave-Schrodinger equation." Journal of Nonlinear Sciences & Applications (JNSA) 13(4):
[14] S. Al-Ahmada, I. M. Sulaimana, M. A. A. Nawib, M. Mamata, and M. Z. Ahmadc, “Analytical solution of systems of Volterra integro-differential equations using modified differential transform method": DOI: 10.22436/JMCS.026.01.01.
[15] X.-L. Yina, S.-X. Konga, Y.-Q. Liua, and X.-T. Zhengb, “New scheme for nonlinear Schrodinger equations with vari-able coefficients":
[16] M. M. Khater, (2021) “Diverse solitary and Jacobian solutions in a continually laminated fluid with respect to shear flows through the Ostrovsky equation" Modern Physics Letters B 35(13): 2150220. DOI: 10.1142/S0217984921502201.
[17] M. M. Khater, S. Elagan, M. El-Shorbagy, S. Alfalqi, J. Alzaidi, and N. A. Alshehri, (2021) “Folded novel accurate analytical and semi-analytical solutions of a generalized Calogero–Bogoyavlenskii–Schiff equation" Communications in Theoretical Physics 73(9): 095003. DOI: 10.1088/1572-9494/ac049f.
[18] M. M. Khater and D. Lu, (2021) “Analytical versus numerical solutions of the nonlinear fractional time–space telegraph equation" Modern Physics Letters B 35(19): 2150324. DOI: 10.1142/S0217984921503243.
[19] M. M. Khater, (2021) “Abundant breather and semianalytical investigation: On high-frequency waves’ dynamics in the relaxation medium" Modern Physics Letters B 35(22): 2150372. DOI: 10.1142/S0217984921503723.
[20] M. M. Khater, (2021) “Diverse bistable dark novel explicit wave solutions of cubic–quintic nonlinear Helmholtz model" Modern Physics Letters B 35(26): 2150441. DOI: 10.1142/S0217984921504418.
[21] M. M. Khater, (2021) “Diverse bistable dark novel explicit wave solutions of cubic–quintic nonlinear Helmholtz model" Modern Physics Letters B 35(26): 2150441. DOI: 10.1142/S0217984921504418.
[22] M. M. Khater, T. A. Nofal, H. Abu-Zinadah, M. S. Lotayif, and D. Lu, (2021) “Novel computational and accurate numerical solutions of the modified Benjamin–Bona–Mahony (BBM) equation arising in the optical illusions field" Alexandria Engineering Journal 60(1): 1797–1806. DOI: 10.1016/j.aej.2020.11.028.
[23] M. M. Khater, (2021) “Numerical simulations of Zakharov’s (ZK) non-dimensional equation arising in Langmuir and ion-acoustic waves" Modern Physics Letters B 35(31): 2150480. DOI: 10.1142/S0217984921504807.
[24] M. M. Khater, M. S. Mohamed, and R. A. Attia, (2021) “On semi analytical and numerical simulations for a mathematical biological model; the time-fractional nonlinear Kolmogorov–Petrovskii–Piskunov (KPP) equation" Chaos, Solitons & Fractals 144: 110676. DOI: 10.1016/j.chaos.2021.110676.
[25] J. Zhang, D. Lu, S. A. Salama, and M. M. Khater, (2022) “Accurate demonstrating of the interactions of two long waves with different dispersion relations: Generalized Hirota–Satsuma couple KdV equation" AIP Advances 12(2): 025015. DOI: 10.1063/5.0084588.
[26] M. M. Khater, A. E.-S. Ahmed, and M. El-Shorbagy, (2021) “Abundant stable computational solutions of Atangana–Baleanu fractional nonlinear HIV-1 infection of CD4+ T-cells of immunodeficiency syndrome" Results in Physics 22: 103890. DOI: 10.1016/j.rinp.2021.103890.
[27] M. M. Khater, A. E.-S. Ahmed, S. Alfalqi, J. Alzaidi, S. Elbendary, and A. M. Alabdali, (2021) “Computational and approximate solutions of complex nonlinear Fokas–Lenells equation arising in optical fiber" Results in Physics 25: 104322. DOI: 10 .1016/j.rinp.2021.104322.
[28] M. M. Khater, A. Mousa, M. El-Shorbagy, and R. A. Attia, (2021) “Analytical and semi-analytical solutions for Phi-four equation through three recent schemes" Results in Physics 22: 103954. DOI: 10.1016/j.rinp.2021.103954.
[29] M. M. Khater, K. S. Nisar, and M. S. Mohamed, (2021) “Numerical investigation for the fractional nonlinear spacetime telegraph equation via the trigonometric Quintic B-spline scheme" Mathematical Methods in the Applied Sciences 44(6): 4598–4606. DOI: 10.1002/mma.7052.
[30] U. Afzal, N. Raza, and I. G. Murtaza, (2019) “On soliton solutions of time fractional form of Sawada–Kotera equation" Nonlinear Dynamics 95(1): 391–405. DOI: 10.1007/s11071-018-4571-9.
[31] M. Khater, R. A. Attia, S. K. Elagan, and F. S. Bayones, (2021) “Analytical and semi analytical solutions of the internal waves of deep-stratified fluids" Thermal Science 25(Spec. issue 2): 227–232. DOI: 10.2298/TSCI21S2227K.
[32] M. M. Khater, S. Anwar, K. U. Tariq, and M. S. Mohamed, (2021) “Some optical soliton solutions to the perturbed nonlinear Schrodinger equation by modified Khater method" AIP Advances 11(2): 025130. DOI: 10.1063/5.0038671.
[33] M. Al-Smadi, O. A. Arqub, and S. Momani, (2020) “Numerical computations of coupled fractional resonant Schrodinger equations arising in quantum mechanics under conformable fractional derivative sense" Physica Scripta 95(7): 075218. DOI: 10.1088/1402-4896/ab96e0.
[34] A. El-Ajou, M. N. Oqielat, Z. Al-Zhour, S. Kumar, and S. Momani, (2019) “Solitary solutions for timefractional nonlinear dispersive PDEs in the sense of conformable fractional derivative" Chaos: An Interdisciplinary Journal of Nonlinear Science 29(9): 093102. DOI: 10.1063/1.5100234.
[35] M. S. Hashemi and D. Baleanu, (2016) “Numerical approximation of higher-order time-fractional telegraph equation by using a combination of a geometric approach and method of line" Journal of Computational Physics 316: 10–20. DOI: 10.1016/j.jcp.2016.04.009.
[36] M. S. Shehata, H. Rezazadeh, E. H. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New optical soliton solutions of the perturbed Fokas-Lenells equation" Communications in Theoretical Physics 71(11): 1275. DOI: 10.1088/0253-6102/71/11/1275.
[37] M. Khater and B. Ghanbari, (2021) “On the solitary wave solutions and physical characterization of gas diffusion in a homogeneous medium via some efficient techniques" The European Physical Journal Plus 136(4): 1–28. DOI: 10.1140/epjp/s13360-021-01457-1.
[38] R. Khalil, M. Al Horani, A. Yousef, and M. Sababheh, (2014) “A new definition of fractional derivative" Journal of computational and applied mathematics 264: 65–70. DOI: 10.1016/j.cam.2014.01.002.
[39] S. Qureshi, (2020) “Effects of vaccination on measles dynamics under fractional conformable derivative wit Liouville–Caputo operator" The European Physical Journal Plus 135(1): 63. DOI: 10.1140/epjp/s13360-020-00133-0.
[40] H. A. Ghany, A.-A. Hyder, and M. Zakarya, (2020) “Exact solutions of stochastic fractional Korteweg de–Vries equation with conformable derivatives" Chinese Physics B 29(3): 030203. DOI: 10.1088/1674-1056/ab75c9.
[41] F. Martinez, I. Martinez, M. K. Kaabar, R. Ortiz-Munuera, and S. Paredes, (2020) “Note on the conformable fractional derivatives and integrals of complex valued functions of a real variable" IAENG International Journal of Applied Mathematics 50(3): 609–615.
[42] M. Darvishi, M. Najafi, and A.-M. Wazwaz, (2021) “Conformable space-time fractional nonlinear (1+1)-dimensional Schrodinger-type models and their traveling wave solutions" Chaos, Solitons & Fractals 150: 111187. DOI: 10.1016/j.chaos.2021.111187.
[43] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable timefractional derivative" Calcolo 53(3): 475–485. DOI: 10.1007/s10092-015-0158-8.
[44] M. T. DARVISHI, M. NAJAFI, and B.-C. SHIN, (2021) “Conformable fractional sense of foam drainage equation and construction of its solutions" Journal of the Korean Society for Industrial and Applied Mathematics 25(3): 132–148.
[45] M. T. Darvishi and M. Najafi, (2020) “Propagation of sech-type solutions for conformable fractional nonlinear Schrodinger models" Com. Meth. Sci. Eng 2(2): 35.
[46] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17): 1950196. DOI: 10.1142/S0217984919501963.
[47] D. Kumar, G. C. Paul, A. R. Seadawy, and M. Darvishi, (2021) “A variety of novel closed-form soliton solutions to the family of Boussinesq-like equations with different types" Journal of Ocean Engineering and Science: DOI: 10.1016/j.joes.2021.10.007.
[48] M. T. Darvishi, M. Najafi, and A.-M. Wazwaz, (2021) “Some optical soliton solutions of space-time conformable fractional Schrodinger-type models" Physica Scripta 96(6): 065213. DOI: 10.1088/1402-4896/abf269.
[49] D. Zhao and M. Luo, (2017) “General conformable fractional derivative and its physical interpretation" Calcolo 54(3): 903–917. DOI: 10.1007/s10092-017-0213-8.
[50] J.-H. He, (1999) “Homotopy perturbation technique" Computer methods in applied mechanics and engineering 178(3-4): 257–262.
[51] H. Aminikhah, A. H. Refahi Sheikhani, and H. Rezazadeh, (2014) “Exact and numerical solutions of linear and non-linear systems of fractional partial differential equations" Journal of Mathematical Modeling 2(1): 22–40.