Shefaa M. N. Jasim and Ghada H. Ibraheem
Department of Mathematics, College of Education for Pure Sciences (Ibn AL-Haitham), University of Baghdad
Received:
July 5, 2022
Accepted:
October 11, 2022
Publication Date:
December 14, 2022
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
Download Citation: ||https://doi.org/10.6180/jase.202309_26(9).0013
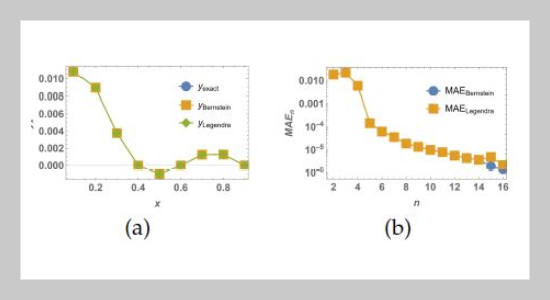
In conducting this research, the operational matrices methodology was applied. Bernstein and Legendre’s operational matrices have been used to solve the Bagley-Torvik equation. The Liouville-Caputo idea characterizes fractional derivative. The goal of these methods is to turn problems into a system of algebraic equations so that the unknowns may be easily found. Mathematica® 12 program was used to get the outcomes. Examples are provided to illustrate the efficacy of the two suggested techniques. Both approaches are successful, as shown by positive comparisons between the approximate and exact solutions to the problems.
Keywords:
Operational matrix, Bernstein polynomials, Legendre polynomials, Bagley-Torvik equations.
- [1] A. Rauf, Y. Mahsud, and I. Siddique, (2020) “Multilayer flows of immiscible fractional Maxwell fluids in a cylindrical domain" Chinese Journal of Physics 67: 265–282. DOI: 10.1016/j.cjph.2019.09.015.
- [2] A. Bonfanti, J. Fouchard, N. Khalilgharibi, G. Charras, and A. Kabla, (2020) “A unified rheological model for cells and cellularised materials" Royal Society open science 7(1): 190920. DOI: 10.1098/rsos.190920.
- [3] S. Q. Hasan and A. A. A. Sahib, (2014) “Convergence of the generalized homotopy perturbation method for solving fractional order integro-differential equations" Baghdad Science Journal 11(4):
- [4] I. Gorial, (2017) “Finite Difference Method for Two-Dimensional Fractional Partial Differential Equation with parameter" Ibn AL-Haitham Journal For Pure and Applied Science 24(2):
- [5] C. Li, A. Chen, and J. Ye, (2011) “Numerical approaches to fractional calculus and fractional ordinary differential equation" Journal of Computational Physics 230(9): 3352–3368. DOI: 10.1016/j.jcp.2011.01.030.
- [6] H. M. Srivastava, (2021) “An introductory overview of fractional-calculus operators based upon the Fox-Wright and related higher transcendental functions" Journal of Advanced Engineering and Computation 5(3): 135–166.
- [7] H. M. Srivastava, (2020) “Fractional-order derivatives and integrals: Introductory overview and recent developments" Kyungpook Mathematical Journal 60(1): 73–116. DOI: 10.5666/KMJ.2020.60.1.73.
- [8] A. A. Kilbas, H. M. Srivastava, and J. J. Trujillo. Theory and applications of fractional differential equations. 204. elsevier, 2006.
- [9] H. Srivastava, (2021) “Some parametric and argument variations of the operators of fractional calculus and related special functions and integral transformations" Journal of Nonlinear and Convex Analysis 22(8): 1501–1520.
- [10] S. A. Yousefi and M. Behroozifar, (2010) “Operational matrices of Bernstein polynomials and their applications" International Journal of Systems Science 41(6): 709–716. DOI: 10.1080/00207720903154783.
- [11] A. Khan, K. Shah, M. Arfan, T. Abdeljawad, and F. Jarad, (2020) “a New Numerical Treatment for Fractional Differential Equations Based on Non-Discretization of Data Using Laguerre Polynomials" Fractals 28(08): 2040046. DOI: 10.1142/S0218348X20400460.
- [12] A. H. Bhrawy, E. H. Doha, D. Baleanu, and S. S. Ezz-Eldien, (2015) “A spectral tau algorithm based on Jacobi operational matrix for numerical solution of time fractional diffusion-wave equations" Journal of Computational Physics 293: 142–156. DOI: 10.1016/j.jcp.2014.03.039.
- [13] A. H. Bhrawy, T. M. Taha, and J. A. T. Machado, (2015) “A review of operational matrices and spectral techniques for fractional calculus" Nonlinear Dynamics 81(3): 1023–1052. DOI: 10.1007/s11071-015-2087-0.
- [14] A. Isah and C. Phang, (2019) “New operational matrix of derivative for solving non-linear fractional differential equations via Genocchi polynomials" Journal of King Saud University-Science 31(1): 1–7. DOI: 10.1016/j.jksus.2017.02.001.
- [15] E. H. Doha, A. H. Bhrawy, and M. Saker, (2011) “Integrals of Bernstein polynomials: an application for the solution of high even-order differential equations" Applied Mathematics Letters 24(4): 559–565. DOI: 10.1016/j.aml.2010.11.013.
- [16] K. Maleknejad, E. Hashemizadeh, and B. Basirat, (2012) “Computational method based on Bernstein operational matrices for nonlinear Volterra–Fredholm–Hammerstein integral equations" Communications in Nonlinear Science and Numerical Simulation 17(1): 52–61. DOI: 10.1016/j.cnsns.2011.04.023.
- [17] M. Dehghan and A. Saadatmandi, (2006) “A tau method for the one-dimensional parabolic inverse problem subject to temperature overspecification" Computers & Mathematics with Applications 52(6-7): 933–940. DOI: 10.1016/j.camwa.2006.04.017.
- [18] A. Saadatmandi and M. Dehghan, (2008) “Numerical solution of a mathematical model for capillary formation in tumor angiogenesis via the tau method" Communications in numerical methods in engineering 24(11): 1467–1474. DOI: 10.1002/cnm.1045.
- [19] A. Saadatmandi and M. Dehghan, (2007) “Numerical solution of the one-dimensional wave equation with an integral condition" Numerical Methods for Partial Differential Equations: An International Journal 23(2): 282–292. DOI: 10.1002/num.20177.
- [20] R. K. Pandey and N. Kumar, (2012) “Solution of Lane–Emden type equations using Bernstein operational matrix of differentiation" New Astronomy 17(3): 303–308. DOI: 10.1016/j.newast.2011.09.005.
- [21] K. Maleknejad, E. Hashemizadeh, and B. Basirat, (2012) “Computational method based on Bernstein operational matrices for nonlinear Volterra–Fredholm–Hammerstein integral equations" Communications in Nonlinear Science and Numerical Simulation 17(1): 52–61. DOI: 10.1016/j.cnsns.2011.04.023.
- [22] W. Abd-Elhameed, Y. Youssri, and E. Doha, (2015) “A novel operational matrix method based on shifted Legendre polynomials for solving second-order boundary value problems involving singular, singularly perturbed and Bratu-type equations" Mathematical Sciences 9(2): 93–102. DOI: 10.1007/s40096-015-0155-8.
- [23] Y. H. Youssri and W. M. Abd-Elhameed, (2020) “Legendre-Spectral Algorithms for Solving Some Fractional Differential Equations" Fractional Order Analysis: Theory, Methods and Applications: 195–224.
- [24] F. Mohammadi and S. T. Mohyud-Din, (2016) “A fractional-order Legendre collocation method for solving the Bagley-Torvik equations" Advances in Difference Equations 2016(1): 1–14. DOI: 10.1186/s13662-016-0989-x.
- [25] H. Srivastava, F. Shah, and R. Abass, (2019) “An application of the Gegenbauer wavelet method for the numerical solution of the fractional Bagley-Torvik equation" Russian Journal of Mathematical Physics 26(1): 77–93. DOI: 10.1134/S1061920819010096.
- [26] A. F. Abduljaleel and A. R. Khudair, (2021) “Technique for Solving the Bagley-Torvik Equation via Integer-Order Differential Equations" Journal of Al-Qadisiyah for computer science and mathematics 13(3): Page–107.
- [27] M. Gülsu, Y. Öztürk, and A. Anapali, (2017) “Numerical solution the fractional Bagley–Torvik equation arising in fluid mechanics" International Journal of Computer Mathematics 94(1): 173–184. DOI: 10.1080/00207160.2015.1099633.
- [28] Y. H. Youssri, (2017) “A new operational matrix of Caputo fractional derivatives of Fermat polynomials: an application for solving the Bagley-Torvik equation" Advances in Difference Equations 2017(1): 1–17. DOI: 10.1186/s13662-017-1123-4.
- [29] A. G. Atta, G. M. Moatimid, and Y. H. Youssri, (2020) “Generalized Fibonacci operational tau algorithm for fractional Bagley-Torvik equation" Prog. Fract. Differ. Appl 6(3): 215–224. DOI: 10.18576/pfda/060305.
- [30] A. Saadatmandi, (2014) “Bernstein operational matrix of fractional derivatives and its applications" Applied Mathematical Modelling 38(4): 1365–1372. DOI: 10.1016/j.apm.2013.08.007.
- [31] H. Srivastava, R. M. Jena, S. Chakraverty, and S. K. Jena, (2020) “Dynamic response analysis of fractionally-damped generalized Bagley–Torvik equation subject to external loads" Russian Journal of Mathematical Physics 27(2): 254–268. DOI: 10 . 1134 /S1061920820020120.
- [32] S. S. Ezz-Eldien, (2016) “New quadrature approach based on operational matrix for solving a class of fractional variational problems" Journal of Computational physics 317: 362–381. DOI: 10.1016/j.jcp.2016.04.045.
- [33] M. E. Benattia and K. Belghaba, (2017) “Numerical solution for solving fractional differential equations using shifted Chebyshev wavelet" Gen. Lett. Math 3(2): 101–110.
- [34] A. Saadatmandi and M. Dehghan, (2010) “A new operational matrix for solving fractional-order differential equations" Computers & mathematics with applications 59(3): 1326–1336. DOI: 10.1016/j.camwa.2009.07.006.
- [35] S. A. Yousefi, M. Behroozifar, and M. Dehghan, (2011) “The operational matrices of Bernstein polynomials for solving the parabolic equation subject to specification of the mass" Journal of computational and applied mathematics 235(17): 5272–5283. DOI: 10.1016/j.cam.2011.05.038.
- [36] M. Akrami, M. Atabakzadeh, and G. Erjaee, (2013) “The operational matrix of fractional integration for shifted Legendre polynomials":