Daftardar-Jafari Method For Solving Conformable K (m, P,1) Equation

Guohua Fu; Xinxin Chang

doi:10.6180/jase.202308_26(8).0008

Daftardar-Jafari Method For Solving Conformable K (m, P,1) Equation

Guohua FuThis email address is being protected from spambots. You need JavaScript enabled to view it. and Xinxin Chang

School of Anyang Institute of Technology, Anyang, 455000, Henan, China

Received: April 19, 2022
Accepted: September 4, 2022
Publication Date: November 2, 2022

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202308_26(8).0008

ABSTRACT

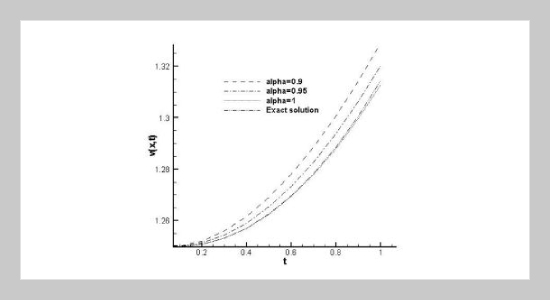
The Daftardar-Jafari technique is a powerful solution method for obtaining approximate and exact solutions of some nonlinear partial differential and integral equations. In this paper, the Daftardar-Jafari method is used to establish approximate solution of the conformable K (m, p,1) equation. This equation has been widely used in the applied sciences and engineering. The conformable derivative (CD) was introduced and explored with Khalil et al. We present the figures and tables to compare between the approximate solutions. The approximate results show that the Daftardar-Jafari method is a very efficient and most reliable approach to handle conformable partial differential and integral equations.

Keywords: The Daftardar-Jafari method, CFD, Conformable K (m, p,1) equation

REFERENCES

[1] M. Safari, D. D. Ganji, and M. Moslemi, (2009) “Application of He’s variational iteration method and Adomian’s decomposition method to the fractional KdV-Burgers-Kuramoto equation" Computers & Mathematics with Applications 58(11-12): 2091–2097. DOI: 10.1016/j.camwa.2009.03.043.
[2] S. Momani and K. AlKhaled, (2005) “Numerical solutions for systems of fractional differential equations by the decomposition method" Applied Mathematics and Computation 162(3): 1351–1365. DOI: 10.1016/j.amc.2004.03.014.
[3] S. Das, (2009) “Analytical solution of a fractional diffusion equation by variational iteration method" Computers & Mathematics with Applications 57(3): 483–487. DOI: 10.1016/j.camwa.2008.09.045.
[4] G.-C. Wu and D. Baleanu, (2013) “Variational iteration method for the Burgers’ flow with fractional derivatives—new Lagrange multipliers" Applied Mathematical Modelling 37(9): 6183–6190. DOI: 10.1016/j.apm.2012.12.018.
[5] M. Dehghan, J. Manafian, and A. Saadatmandi, (2010) “Solving nonlinear fractional partial differential equations using the homotopy analysis method" Numerical Methods for Partial Differential Equations: An International Journal 26(2): 448–479. DOI: 10.1002/num.20460.
[6] H. Jafari and S. Seifi, (2009) “Homotopy analysis method for solving linear and nonlinear fractional diffusion-wave equation" Communications in Nonlinear Science and Numerical Simulation 14(5): 2006–2012. DOI: 10.1016/j.cnsns.2008.05.008.
[7] Z. Ganji, D. D. Ganji, A. D. Ganji, and M. Rostamian, (2010) “Analytical solution of time-fractional Navier–Stokes equation in polar coordinate by homotopy perturbation method" Numerical Methods for Partial Differential Equations: An International Journal 26(1): 117–124. DOI: 10.1002/num.20420.
[8] E. Unal and A. Gokdougan, (2017) “Solution of conformable fractional ordinary differential equations via differential transform method" Optik 128: 264–273. DOI: 10.1016/j.ijleo.2016.10.031.
[9] A. Arikoglu and I. Ozkol, (2007) “Solution of fractional differential equations by using differential transform method" Chaos, Solitons & Fractals 34(5): 1473–1481. DOI: 10.1016/j.chaos.2006.09.004.
[10] W. Al-Hayani et al., (2017) “Daftardar-Jafari method for fractional heat-like and wave-like equations with variable coefficients" Applied Mathematics 8(02): 215.
[11] S. Bhalekar and V. Daftardar-Gejji, (2012) “Solving fractional-order logistic equation using a new iterative method" International Journal of Differential Equations 2012: DOI: 10.1155/2012/975829.
[12] L. Akinyemi, (2021) “Two improved techniques for the perturbed nonlinear Biswas–Milovic equation and its optical solitons" Optik 243: 167477. DOI: 10.1016/j.ijleo.2021.167477.
[13] H. Rezazadeh, D. Kumar, T. A. Sulaiman, and H. Bulut, (2019) “New complex hyperbolic and trigonometric solutions for the generalized conformable fractional Gardner equation" Modern Physics Letters B 33(17): 1950196. DOI: 10.1142/S0217984919501963.
[14] N. NGbo and Y. Xia, (2022) “Traveling Wave Solution of Bad and Good Modified Boussinesq Equations with Conformable Fractional-Order Derivative" Qualitative Theory of Dynamical Systems 21(1): 1–21. DOI: 10.1007/s12346-021-00541-2.
[15] B. Zhang, W. Zhu, Y. Xia, and Y. Bai, (2020) “A unified analysis of exact traveling wave solutions for the fractional-order and integer-order Biswas–Milovic equation: via bifurcation theory of dynamical system" Qualitative theory of dynamical systems 19(1): 1–28. DOI: 10.1007/s12346-020-00352-x.
[16] W. Zhu, Y. Xia, B. Zhang, and Y. Bai, (2019) “Exact traveling wave solutions and bifurcations of the timefractional differential equations with applications" International Journal of Bifurcation and Chaos 29(03): 1950041. DOI: 10.1142/S021812741950041X.
[17] H. Zheng, Y. Xia, Y. Bai, and L. Wu, (2021) “Travelling wave solutions of the general regularized long wave equation" Qualitative theory of dynamical systems 20(1): 1–21. DOI: 10.1007/s12346-020-00442-w.
[18] S. Abbagari, Y. Saliou, A. Houwe, L. Akinyemi, M. Inc, and T. B. Bouetou, (2022) “Modulated wave and modulation instability gain brought by the cross-phase modulation in birefringent fibers having anti-cubic nonlinearity" Physics Letters A 442: 128191. DOI: 10.1016/j.physleta.2022.128191.
[19] K. S. Nisar, M. Inc, A. Jhangeer, M. Muddassar, and B. Infal, (2022) “New soliton solutions of Heisenberg ferromagnetic spin chain model" Pramana 96(1): 1–8. DOI: 10.1007/s12043-021-02266-y.
[20] H. Halidou, S. Abbagari, A. Houwe, M. Inc, and B. B. Thomas, (2022) “Rational W-shape solitons on a nonlinear electrical transmission line with Josephson junction" Physics Letters A 430: 127951. DOI: 10.1016/j.physleta.2022.127951.
[21] S.-W. Yao, R. Manzoor, A. Zafar, M. Inc, S. Abbagari, and A. Houwe, (2022) “Exact soliton solutions to the Cahn–Allen equation and Predator–Prey model with truncated M-fractional derivative" Results in Physics 37: 105455. DOI: 10.1016/j.rinp.2022.105455.
[22] K. S. Nisar, I. E. Inan, H. Yepez-Martinez, and M. Inc, (2022) “Some new type optical and the other soliton solutions of coupled nonlinear Hirota equation" Results in Physics 35: 105388. DOI: 10.1016/j.rinp.2022.105388.
[23] M. M. Khater, (2021) “Diverse bistable dark novel explicit
wave solutions of cubic–quintic nonlinear Helmholtz model" Modern Physics Letters B 35(26): 2150441. DOI: 10.1142/S0217984921504418.
[24] H. Rezazadeh, (2018) “New solitons solutions of the complex Ginzburg-Landau equation with Kerr law nonlinearity" Optik 167: 218–227. DOI: 10.1016/j.ijleo.2018.04.026.
[25] M. M. Khater, (2021) “Abundant wave solutions of the perturbed Gerdjikov–Ivanov equation in telecommunication industry" Modern Physics Letters B 35(26): 2150456. DOI: 10.1142/S021798492150456X.
[26] M. M. Khater, S. Elagan, M. El-Shorbagy, S. Alfalqi, J. Alzaidi, and N. A. Alshehri, (2021) “Folded novel accurate analytical and semi-analytical solutions of a generalized Calogero–Bogoyavlenskii–Schiff equation" Communications in Theoretical Physics 73(9): 095003. DOI: 10.1088/1572-9494/ac049f.
[27] M. M. Khater and D. Lu, (2021) “Analytical versus numerical solutions of the nonlinear fractional time–space telegraph equation" Modern Physics Letters B 35(19): 2150324. DOI: 10.1142/S0217984921503243.
[28] M. M. Khater, (2021) “Abundant breather and semianalytical investigation: On high-frequency waves’ dynamics in the relaxation medium" Modern Physics Letters B 35(22): 2150372. DOI: 10.1142/S0217984921503723.
[29] M. S. Shehata, H. Rezazadeh, E. H. Zahran, E. Tala-Tebue, and A. Bekir, (2019) “New optical soliton solutions of the perturbed Fokas-Lenells equation" Communications in Theoretical Physics 71(11): 1275. DOI: 10.1088/0253-6102/71/11/1275.
[30] M. M. Khater, (2021) “Numerical simulations of Zakharov’s (ZK) non-dimensional equation arising in Langmuir and ion-acoustic waves" Modern Physics Letters B 35(31): 2150480.
[31] M. M. Khater, (2021) “Diverse solitary and Jacobian solutions in a continually laminated fluid with respect to shear flows through the Ostrovsky equation" Modern Physics Letters B 35(13): 2150220. DOI: 10.1142/S0217984921502201.
[32] V. Daftardar-Gejji and H. Jafari, (2006) “An iterative method for solving nonlinear functional equations" Journal of mathematical analysis and applications 316(2): 753–763. DOI: 10.1016/j.jmaa.2005.05.009.
[33] J. Patade and S. Bhalekar, (2015) “A new numerical method based on Daftardar-Gejji and Jafari technique for solving differential equations"World J. Model. Simul 11: 256–271.
[34] I. Ullah, H. Khan, and M. T. Rahim, (2014) “Numerical solutions of fifth and sixth order nonlinear boundary value problems by Daftardar Jafari method" Journal of Computational Engineering 2014:
[35] M. A. AL-Jawary, G. H. Radhi, and J. Ravnik, (2018) “Daftardar-Jafari method for solving nonlinear thin film flow problem" Arab Journal of Basic and Applied Sciences 25(1): 20–27. DOI: 10.1080/25765299.2018.1449345.
[36] H. Koçak, T. Özi¸s, and A. Yıldırım, (2010) “Homotopy perturbation method for the nonlinear dispersive K (m, n, 1) equations with fractional time derivatives" International Journal of Numerical Methods for Heat & Fluid Flow 20(2): 174–185. DOI: 10.1108/09615531011016948.
[37] L. Tian and J. Yin, (2007) “Shock-peakon and shockcompacton solutions for K (p, q) equation by variational iteration method" Journal of Computational and Applied Mathematics 207(1): 46–52. DOI: 10.1016/j.cam.2006.07.026.
[38] Y. Zhu, K. Tong, and T. Chaolu, (2007) “New exact solitary-wave solutions for the K (2, 2, 1) and K (3, 3, 1) equations" Chaos, Solitons & Fractals 33(4): 1411–1416. DOI: 10.1016/j.chaos.2006.01.090.
[39] Y. Cenesiz, Y. Keskin, and A. Kurnaz, (2011) “The solution of the nonlinear dispersive K (m, n, 1) equations by RDT method" Selcuk Journal of Applied Mathematics 12(2): 53–61.
[40] L. Tian and J. Yin, (2005) “Stability of multi-compacton solutions and Backlund transformation in K (m, n, 1)" Chaos, Solitons & Fractals 23(1): 159–169. DOI: 10.1016/j.chaos.2004.04.004.
[41] R. Khalil, M. Al Horani, A. Yousef, and M. Sababheh, (2014) “A new definition of fractional derivative" Journal of computational and applied mathematics 264: 65–70. DOI: 10.1016/j.cam.2014.01.002.
[42] T. Abdeljawad, (2015) “On conformable fractional calculus" Journal of computational and Applied Mathematics 279: 57–66. DOI: 10.1016/j.cam.2014.10.016.
[43] M. Eslami and H. Rezazadeh, (2016) “The first integral method for Wu–Zhang system with conformable timefractional derivative" Calcolo 53(3): 475–485. DOI: 10.1007/s10092-015-0158-8.
[44] H. Aminikhah, A. H. R. Sheikhani, and H. Rezazadeh, (2016) “Travelling wave solutions of nonlinear systems of PDEs by using the functional variable method" Boletim da sociedade paranaense de matemática 34(2): 213–229. DOI: 10.5269/bspm.v34i2.25501.
[45] H. Rezazadeh, A. Korkmaz, M. Eslami, J. Vahidi, and R. Asghari, (2018) “Traveling wave solution of conformable fractional generalized reaction Duffing model by generalized projective Riccati equation method" Optical and Quantum Electronics 50(3): 1–13. DOI: 10.1007/s11082-018-1416-1.
[46] A. Korkmaz, (2019) “Explicit exact solutions to some one-dimensional conformable time fractional equations" Waves in Random and Complex Media 29(1): 124–137. DOI: 10.1080/17455030.2017.1416702.
[47] H. Aminikhah, A. R. Sheikhani, and H. Rezazadeh, (2015) “Exact solutions for the fractional differential equations by using the first integral method" Nonlinear engineering 4(1): 15–22. DOI: 10.1515/nleng-2014-0018.