Analytical and Numerical Investigation of Buckling Behavior of Functionally Graded Sandwich Plate with Porous Core

Emad Kadum Njim; Sadeq H. Bakhy; Muhannad Al-Waily

doi:10.6180/jase.202204_25(2).0010

Analytical and Numerical Investigation of Buckling Behavior of Functionally Graded Sandwich Plate with Porous Core

Emad Kadum Njim¹, Sadeq H. Bakhy¹, and Muhannad Al-Waily This email address is being protected from spambots. You need JavaScript enabled to view it.²

¹University of Technology, Mechanical Engineering Department, Iraq
²Department of Mechanical Engineering, Faculty of Engineering, University of Kufa, Iraq

Received: April 21, 2021
Accepted: August 6, 2021
Publication Date: August 29, 2021

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202204_25(2).0010

ABSTRACT

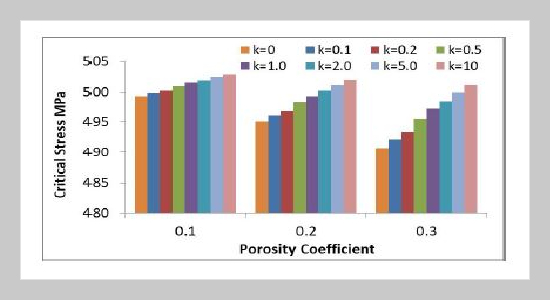
This research presents a new analytical model for the buckling analysis of a functionally graded rectangular sandwich plate with all edges simply supported and subjected to in-plane loading conditions. The sandwich plate comprises a porous metal core with an isotropic metal that serves as a skin. The functionally graded core metal has a varied distribution of porosities in accordance with the plate thickness, which may lead to variation in material properties. By considering classical plate theory principles, the kinematic relations are used to obtain the analytical solution. The effects of changing gradient index, elastic parameters, porosity distribution, slenderness ratio, boundary conditions, and core materials on buckling stresses and dynamic response of functionally graded sandwich plates are presented. A numerical study on elastic buckling using finite element analysis (FEA) was employed to examine the accuracy of the proposed analytical solution. From the obtained results, it is found that the porosity coefficients play significant influences on the buckling behavior and reliability of the FG sandwich structures.

Keywords: Functionally graded materials; Porous metal core; Theoretical formulation; Stability; Critical buckling stress; FEA

REFERENCES

[1] E. Njim, M. Al-Waily, and S. Bakhy, (2021) “A review of the recent research on the experimental tests of functionally graded sandwich panels" Journal of Mechanical Engineering Research and Developments 44(3): 420–441.
[2] B. Saleh, J. Jiang, R. Fathi, T. Al-hababi, Q. Xu, L. Wang, D. Song, and A. Ma, (2020) “30 Years of functionally graded materials: An overview of manufacturing methods, Applications and Future Challenges" Composites Part B: Engineering 201: DOI: 10 . 1016 / j.compositesb.2020.108376.
[3] A. Garg, M.-O. Belarbi, H. Chalak, and A. Chakrabarti, (2021) “A review of the analysis of sandwich FGM structures" Composite Structures 258: DOI:10.1016/j.compstruct.2020.113427.
[4] K. Magnucki and E. Magnucka-Blandzi, (2021) “Generalization of a sandwich structure model: Analytical studies of bending and buckling problems of rectangular plates" Composite Structures 255: DOI: 10.1016/j.compstruct.2020.112944.
[5] S. C. Chikr, A. Kaci, A. A. Bousahla, F. Bourada, A. Tounsi, E. Bedia, S. Mahmoud, K. H. Benrahou, and A. Tounsi, (2020) “A novel four-unknown integral model for buckling response of FG sandwich plates resting on elastic foundations under various boundary conditions using Galerkin’s approach" Geomechanics and Engineering21(5): 471–487.
[6] S. Refrafi, A. Bousahla, A. Bouhadra, A. Menasria, F. Bourada, A. Tounsi, E. Bedia, S. Mahmoud, K. Benrahou, and A. Tounsi, (2020) “Effects of hygro-thermomechanical conditions on the buckling of FG sandwich plates resting on elastic foundations" Computers and Concrete 25(4): 311–325. DOI: 10.12989/cac.2020.25.4.311.
[7] M. Uslu Uysal and U. Güven, (2015) “Buckling of functional graded polymeric sandwich panel under different load cases" Composite Structures 121: 182–196. DOI:10.1016/j.compstruct.2014.11.012.
[8] R. Abo-bakr, H. Abo-bakr, S. Mohamed, and M. Eltaher, (2021) “Optimal weight for buckling of FG beam under variable axial load using Pareto optimality" Composite Structures 258: DOI: 10.1016/j .compstruct.2020.113193.
[9] Y. Sitli, K. Mhada, O. Bourihane, and H. Rhanim, (2021) “Buckling and post-buckling analysis of a functionally graded material (FGM) plate by the Asymptotic Numerical Method" Structures 31: 1031–1040. DOI: 10.1016/j.istruc.2021.01.100.
[10] M. Arefi and F. Najafitabar, (2021) “Buckling and free vibration analyses of a sandwich beam made of a soft core with FG-GNPs reinforced composite face-sheets using Ritz Method" Thin-Walled Structures 158: DOI: 10.1016/j.tws.2020.107200.
[11] O. Civalek and M. Jalaei, (2020) “Shear buckling analysis of functionally graded (FG) carbon nanotube reinforced skew plates with different boundary conditions" Aerospace Science and Technology 99: DOI: 10.1016/j.ast.2020.105753.
[12] M. Kaddari, A. Kaci, A. Bousahla, A. Tounsi, F. Bourada, A. Tounsi, E. Bedia, and M. Al-Osta, (2020) “A study on the structural behaviour of functionally graded porous plates on elastic foundation using a new quasi-3D model: Bending and free vibration analysis" Computers and Concrete 25(1): 37–57. DOI: 10.12989/cac.2020.25.1.037.
[13] J. Wang, S. Cao, and W. Zhang, (2021) “Thermal vibration and buckling analysis of functionally graded carbon nanotube reinforced composite quadrilateral plate" European Journal of Mechanics, A/Solids 85: DOI:10.1016/j.euromechsol.2020.104105.
[14] H. Lu, W. Zhang, and J. Mao. “Buckling analyses of functionally graded graphene nanoplatelets reinforced nonlocal piezoelectric microplate”. In: 774. 1. cited By 0. 2020. DOI: 10.1088/1757-899X/774/1/012103.
[15] C. Li, H.-S. Shen, and H.Wang, (2020) “Postbuckling behavior of sandwich plates with functionally graded auxetic 3D lattice core" Composite Structures 237: DOI:10.1016/j.compstruct.2020.111894.
[16] N. Nguyen, H. Nguyen-Xuan, D. Lee, and J. Lee, (2020) “A novel computational approach to functionally graded porous plates with graphene platelets reinforcement" Thin-Walled Structures 150: DOI: 10.1016/j.tws.2020.106684.
[17] B. Adhikari, P. Dash, and B. Singh, (2020) “Buckling analysis of porous FGM sandwich plates under various types nonuniform edge compression based on higher order shear deformation theory" Composite Structures 251: DOI: 10.1016/j.compstruct.2020.112597.
[18] R. Moradi-Dastjerdi, K. Behdinan, B. Safaei, and Z. Qin, (2020) “Buckling behavior of porous CNT-reinforced plates integrated between active piezoelectric layers" Engineering Structures 222: DOI: 10.1016/j.engstruct. 2020.111141.
[19] C.-L. Thanh, K. Nguyen, N. Nguyen-Trong, S. Khatir, H. Nguyen-Xuan, and M. Abdel-Wahab, (2021) “A three-dimensional solution for free vibration and buckling of annular plate, conical, cylinder and cylindrical shell of FG porous-cellular materials using IGA" Composite Structures 259: DOI: 10 . 1016 / j . compstruct . 2020 . 113216.
[20] M. Bacciocchi, (2020) “Buckling analysis of three-phase CNT/polymer/fiber functionally graded orthotropic plates: Influence of the non-uniform distribution of the oriented fibers on the critical load" Engineering Structures 223:DOI: 10.1016/j.engstruct.2020.111176.
[21] M.-C. Trinh, T. Mukhopadhyay, and S.-E. Kim, (2020) “A semi-analytical stochastic buckling quantification of porous functionally graded plates" Aerospace Science and Technology 105: DOI: 10.1016/j.ast.2020.105928.
[22] V. V. Pham and Q. H. Le, (2021) “Finite element analysis of functionally graded sandwich plates with porosity via a new hyperbolic shear deformation theory" Defence Technology: DOI: 10.1016/j.dt.2021.03.006.
[23] A. Daikh and A. Zenkour, (2019) “Free vibration and buckling of porous power-law and sigmoid functionally graded sandwich plates using a simple higher-order shear deformation theory" Materials Research Express 6(11): DOI: 10.1088/2053-1591/ab48a9.
[24] M. Guellil, H. Saidi, F. Bourada, A. Bousahla, A. Tounsi, M. Al-Zahrani, M. Hussain, and S. Mahmoud, (2021) “Influences of porosity distributions and boundary conditions on mechanical bending response of functionally graded plates resting on Pasternak foundation" Steel and Composite Structures 38(1): 1–15. DOI: 10.12989/scs.2021.38.1.001.
[25] A. Zenkour, (2020) “Quasi-3D Refined Theory for Functionally Graded Porous Plates: Displacements and Stresses" Physical Mesomechanics 23(1): 39–53. DOI:10.1134/S1029959920010051.
[26] M. Al-Waily, M. Al-Shammari, and M. Jweeg, (2020) “An analytical investigation of thermal buckling behavior of composite plates reinforced by carbon nano particles" Engineering Journal 24(3): 11–21. DOI: 10.4186/ej.2020.24.3.11.
[27] Y. Liu, Y. Hu, T. Liu, J. Ding, and W. Zhong, (2015) “Mechanical behavior of high density polyethylene and its carbon nanocomposites under quasi-static and dynamic compressive and tensile loadings" Polymer Testing 41: 106–116. DOI: 10.1016/j.polymertesting.2014.11.003.
[28] N. Bonnheim, F. Ansari, M. Regis, P. Bracco, and L. Pruitt, (2019) “Effect of carbon fiber type on monotonic and fatigue properties of orthopedic grade PEEK" Journal of the Mechanical Behavior of Biomedical Materials 90: 484–492. DOI: 10.1016/j.jmbbm.2018.10.033.
[29] E. K. Njim, S. H. Bakhy, and M. Al-Waily, (2021) “Optimization design of vibration characterizations for functionally graded porous metal sandwich plate structure" Materials Today: Proceedings: