Observer Design for RIP Stability Using Takagi-Sugeno Fuzzy Model and Mean Value Theorem

Quy-Thinh  Dao; Bao-Trung  Dong; Thi-Van-Anh  Nguyen

doi:10.6180/jase.202509_28(9).0019

Observer Design for RIP Stability Using Takagi-Sugeno Fuzzy Model and Mean Value Theorem

Quy-Thinh Dao, Bao-Trung Dong, and Thi-Van-Anh NguyenThis email address is being protected from spambots. You need JavaScript enabled to view it.

School of Electrical and Electronic Engineering, Hanoi University of Science and Technology, Hanoi 100000, Vietnam

Received: July 4, 2024
Accepted: November 5, 2024
Publication Date: January 13, 2025

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202509_28(9).0019

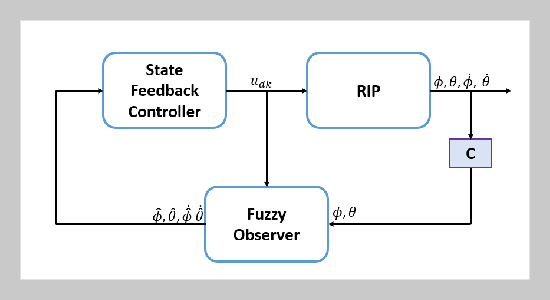
This paper presents an observer design for the Rotary Inverted Pendulum (RIP) system using the Takagi-Sugeno (T-S) fuzzy model and the mean value theorem. The proposed method addresses the nonlinear and inherently unstable dynamics of the RIP by transforming the error dynamics into a linear parametric varying system. A non-quadratic Lyapunov function candidate is employed to ensure the global exponential convergence of the estimation error to zero. By combining the differential mean value theorem with sector nonlinearity transformation, the observer guarantees robust performance in the presence of unmeasured premise variables. The stability conditions are derived based on the Lyapunov function, leading to the solvability of a set of linear matrix inequalities. Simulation results demonstrate the effectiveness of the proposed observer in significantly reducing dynamic errors and enhancing the overall stability and accuracy of state estimations. The proposed approach outperforms traditional methods, providing a reliable and precise solution for real-time control applications in nonlinear systems.

Keywords: Observer Design; Takagi-Sugeno Fuzzy Model; Stability control; Mean Value Theorem; Rotary Inverted Pendulum

[1] R. Hmidi, A. Ben Brahim, S. Dhahri, F. Ben Hmida, and A. Sellami, (2021) “Sliding mode fault-tolerant con trol for Takagi-Sugeno fuzzy systems with local nonlin ear models: Application to inverted pendulum and cart system" Transactions of the Institute of Measure ment and Control 43(4): 975–990. DOI: 10.1177/0142331220949.
[2] T.-V.-A. Nguyen, B.-T. Dong, and N.-T. Bui, (2023) “Enhancing stability control of inverted pendulum using Takagi–Sugeno fuzzy model with disturbance rejection and input–output constraints" Scientific Reports 13(1): 14412. DOI: 10.1038/s41598-023-41258-3.
[3] C.-H.Chiu,Y.-T. Hung,andY.-F.Peng,(2021)“Design of a decoupling fuzzy control scheme for omnidirectional inverted pendulum real-world control" IEEE Access 9: 26083–26092. DOI: 10.1109/ACCESS.2021.3057658.
[4] C.-C. Ku and S.-H. Jian, (2023) “Parameter-Dependent Polynomial Fuzzy Control of Nonlinear Inverted Pendu lum System" International Journal of Fuzzy Systems 25(5): 1770–1781. DOI: 10.1007/s40815-023-01473-6.
[5] M.Rahmawaty, (2021) “Modeling, Simulation, and Sta bilization of a Two-Wheeled Inverted Pendulum Robot Using Hybrid Fuzzy Control" Indonesian Journal of electronics, electromedical engineering, and medi cal informatics 3(3): DOI: 10.35882/ijeeemi.v3i3.2.
[6] M.-L. Nguyen, H.-P. Nguyen, and T.-V.-A. Nguyen, (2024) “H-Infinity Approach Control On Takagi-Sugeno Fuzzy Model For 2-D Overhead Crane System" Journal of Applied Science and Engineering 28: 995–1003. DOI: 10.6180/jase.202505_28(5).0008.
[7] A.-T. Nguyen, V. Campos, T.-M. Guerra, J. Pan, and W. Xie, (2021) “Takagi–Sugeno fuzzy observer design for nonlinear descriptor systems with unmeasured premise variables and unknown inputs" International Journal of Robust and Nonlinear Control 31(17): 8353–8372. DOI: 10.1002/rnc.5453.
[8] M. Ouzaz, A. El Assoudi, et al., (2023) “Fuzzy Ob server Design for State and Fault Estimation for Takagi Sugeno Implicit Models" International Journal of Fuzzy Logic and Intelligent Systems 23(1): 1–10. DOI: 10.5391/IJFIS.2023.23.1.1.
[9] W. Hamdi, M. Y. Hammoudi, and A. Boukhlouf, (2023) “Observer Design for Takagi–Sugeno Fuzzy Sys tems with Unmeasurable Premise Variables Based on Dif ferential Mean Value Theorem" Engineering Proceed ings 58(1): 28. DOI: 10.3390/ecsa-10-16008.
[10] K. Mimoune, M. Y. Hammoudi, R. Saadi, M. Ben bouzid, and A. Boukhlouf, (2023) “Real-time imple mentation of non linear observer based state feedback controller for induction motor using mean value theo rem" Journal of Electrical Engineering & Technol ogy 18(1): 615–628. DOI: 10.1007/s42835-022-01274-1.
[11] Y. Kim, Y. Lee, S. Lee, and O. Kwon. “T-S fuzzy con troller design for Rotary Inverted Pendulum with input delay using Wirtinger-based integral inequal ity”. In: 2022 22nd International Conference on Control, Automation and Systems (ICCAS). IEEE. 2022, 890–895. DOI: 10.23919/ICCAS55662.2022.10003811.
[12] D.-B. Pham, Q.-T. Dao, N.-T. Bui, and T.-V.-A. Nguyen, (2024) “Robust-optimal control of rotary in verted pendulum control through fuzzy descriptor-based techniques" Scientific Reports 14(1): 5593. DOI: 10.1038/s41598-024-56202-2.
[13] K. Mimoune, M. Hammoudi,W.Hamdi,andS.Mi moune, (2023) “Observer design for Takagi–Sugeno fuzzy systems with unmeasured premise variables: Conser vatism reduction using line integral Lyapunov function" ISA transactions 142: 626–634. DOI: 10.1016/j.isatra.2023.07.039.
[14] M. Y. Hammoudi, A. Allag, M. Becherif, M. Ben bouzid, and H. Alloui, (2014) “Observer design for induction motor: an approach based on the mean value the orem" Frontiers in Energy 8: 426–433. DOI: 10.1007/s11708-014-0314-x.
[15] D. Ichalal, B. Marx, S. Mammar, D. Maquin, and J. Ragot. “Observer for Lipschitz nonlinear systems: MeanValue Theorem and sector nonlinearity trans formation”. In: 2012 IEEE International Symposium on Intelligent Control. 2012, 264–269. DOI: 10.1109/ISIC. 2012.6398269.
[16] B.-J. Rhee and S. Won, (2006) “A new fuzzy Lyapunov function approach for a Takagi–Sugeno fuzzy control sys temdesign" Fuzzysetsandsystems157(9):1211–1228. DOI: 10.1016/j.fss.2005.12.020.