Emergent Pressure Dynamics In Coupled Nephron Compliance Tubular Model Using Cellular Automata Rules

Siva Manohar Reddy Kesu; Hariharan Ramasangu

doi:10.6180/jase.202310_26(10).0001

Emergent Pressure Dynamics In Coupled Nephron Compliance Tubular Model Using Cellular Automata Rules

Computer Science and Information Engineering

Siva Manohar Reddy kesu This email address is being protected from spambots. You need JavaScript enabled to view it.¹and Hariharan Ramasangu²

¹Dept. of Electronics and Communications Engineering, M.S. Ramaiah University of Applied Sciences, Bengaluru, India
²Research Division, Relecura. Inc, Bengaluru, India

Received: September 14, 2022
Accepted: November 28, 2022
Publication Date: December 22, 2022

Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.

Download Citation: ||https://doi.org/10.6180/jase.202310_26(10).0001

ABSTRACT

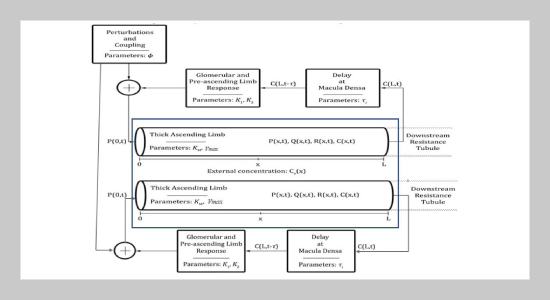
The dynamics of a coupled nephron model can be analyzed by studying the local and global properties. The governing equations of the model are solved using numerical methods to investigate the point-to-point spatiotemporal evolution. Bifurcation analysis has been used to study the global properties. The Cellular Automata (CA) framework was successfully used in the past to model emergent behavior of dynamical systems. The CA approach has not yet been investigated in the context of the coupled nephron model. In this paper, a CA approach has been proposed to study the global properties of the coupled nephron model for compliance tubule. Both tropical and Cole-Hopf transformation have been applied while arriving at the ultradiscretized equations. The CA rules have been derived from ultradiscretization for different transition cases. The global behavior of the derived CA rules has been compared with the class of dynamical systems, which has been observed in the experimental studies on nephrons. It is found that the CA approach captures the richness of the dynamical system behavior observed in the nephron experiments. This is promising and may lead to the development of CA models for analyzing the local behavior in future.

Keywords: nephron; Pressure dynamics; cellular automata; ultradiscretization; emergent properties

REFERENCES

[1] J. L. Laugesen, E. Mosekilde, and Z. T. Zhusubaliyev, (2012) “Bifurcation structure of the C-type perioddoubling transition" Physica D: Nonlinear Phenomena 241(5): 488–496.
[2] J. L. Laugesen, O. V. Sosnovtseva, E. Mosekilde, N.-H. Holstein-Rathlou, and D. J. Marsh, (2010) “Couplinginduced complexity in nephron models of renal blood flow regulation" American Journal of Physiology-Regulatory, Integrative and Comparative Physiology 298(4): R997–R1006.
[3] O. Sosnovtseva, D. Postnov, E. Mosekilde, and N.-H. Holstein-Rathlou, (2003) “Synchronization of tubular pressure oscillations in interacting nephrons" Chaos, Solitons & Fractals 15(2): 343–369.
[4] N.-H. Holstein-Rathlou, K.-P. Yip, O. V. Sosnovtseva, and E. Mosekilde, (2001) “Synchronization phenomena in nephron–nephron interaction" Chaos: An Interdisciplinary Journal of Nonlinear Science 11(2): 417–426.
[5] D. J. Marsh, D. D. Postnov, O. V. Sosnovtseva, and N.-H. Holstein-Rathlou, (2019) “The nephron-arterial network and its interactions" American Journal of Physiology-Renal Physiology 316(5): F769–F784.
[6] D. J. Marsh, D. D. Postnov, D. J. Rowland, A. S. Wexler, O. V. Sosnovtseva, and N.-H. Holstein-Rathlou, (2017) “Architecture of the rat nephron-arterial network: analysis with micro-computed tomography" American Journal of Physiology-Renal Physiology 313(2): F351–F360.
[7] D. J. Marsh, O. V. Sosnovtseva, K. H. Chon, and N.-H. Holstein-Rathlou, (2005) “Nonlinear interactions in renal blood flow regulation" American Journal of Physiology-Regulatory, Integrative and Comparative Physiology 288(5): R1143–R1159.
[8] D. J. Marsh, O. V. Sosnovtseva, A. N. Pavlov, K.-P. Yip, and N.-H. Holstein-Rathlou, (2005) “Frequency encoding in renal blood flow regulation" American Journal of Physiology-Regulatory, Integrative and Comparative Physiology 288(5): R1160–R1167.
[9] D. J. Marsh, O. V. Sosnovtseva, E. Mosekilde, and N.-H. Holstein-Rathlou, (2007) “Vascular coupling induces synchronization, quasiperiodicity, and chaos in a nephron tree" Chaos: An Interdisciplinary Journal of Nonlinear Science 17(1): 015114.
[10] D. J. Marsh, A. S. Wexler, A. Brazhe, D. E. Postnov, O. V. Sosnovtseva, and N.-H. Holstein-Rathlou, (2012) “Multinephron dynamics on the renal vascular network" American Journal of Physiology-Renal Physiology 304(1): F88–F102.
[11] N.-H. Holstein-Rathlou and P. Leyssac, (1986) “TGFmediated oscillations in the proximal intratubular pressure: differences between spontaneously hypertensive rats and Wistar-Kyoto rats" Acta Physiologica 126(3): 333–339.
[12] S. P. Kuznetsov, (2005) “Effect of noise on the dynamics at the Torus-doubling terminal point in a quadratic map under quasiperiodic driving" Physical Review E 72(2): 026205.
[13] J. L. Laugesen, (2011) “Modelling Nephron Autoregulation and Synchronization in Coupled Nephron Systems" Technical University of Denmark (DTU), PhD thesis:
[14] A. S. Pikovsky, M. G. Rosenblum, G. V. Osipov, and J. Kurths, (1997) “Phase synchronization of chaotic oscillators by external driving" Physica D: Nonlinear Phenomena 104(3-4): 219–238.
[15] A. Pavlov, O. Pavlova, A. Koronovskii, and A. Hramov, (2018) “Effect of measuring noise on scaling characteristics in the dynamics of coupled chaotic systems" Chaos, Solitons & Fractals 116: 106–113.
[16] O. V. Sosnovtseva, A. N. Pavlov, E. Mosekilde, K.-P. Yip, N.-H. Holstein-Rathlou, and D. J. Marsh, (2007) “Synchronization among mechanisms of renal autoregulation is reduced in hypertensive rats" American Journal of Physiology-Renal Physiology 293(5): F1545–F1555.
[17] A. Aljuboori, L. A. Tawfeeq, and K. A. Al-Karawi, (2021) “Pushing towards ehealth for iraqi hypertensive: an integrated class association rules into SECI model" Indonesian Journal of Electrical Engineering and Computer Science 22(1): 522–533.
[18] K. Nishinari and D. Takahashi, (1998) “Analytical properties of ultradiscrete Burgers equation and rule-184 cellular automaton" Journal of Physics A: Mathematical and General 31(24): 5439.
[19] M. Murata, (2013) “Tropical discretization: ultradiscrete Fisher–KPP equation and ultradiscrete Allen–Cahn equation" Journal of Difference Equations and Applications 19(6): 1008–1021.
[20] K. Matsuya and M. Murata, (2015) “Spatial pattern of discrete and ultradiscrete Gray-Scott model" Discrete & Continuous Dynamical Systems-B 20(1): 173.
[21] Wolfram, Stephen, (1984) “Computation theory of cellular automata" Communications in Mathematical Physics 96(1): 15–57.
[22] Wolfram S. A new kind of science. 5. Wolfram Media Champaign, IL, 2002.
[23] S. Wolfram. Cellular automata and complexity: collected papers. CRC Press, 2018.
[24] H. Ryu. “Feedback-Mediated Dynamics in the Kidney: Mathematical Modeling and Stochastic Analysis". (phdthesis). Duke University, 2014.
[25] S. M. R. Kesu and H. Ramasangu, (2022) “Cellular automata model for emergent properties of pressure flow in single nephron compliance tubule" Indonesian Journal of Electrical Engineering and Computer Science 25(3): 1227–1235.
[26] A. T. Layton and A. Edwards, (2014) “Mathematical Modeling in Renal Physiology" Springer ISBN 978-3-642-27367-4: VIII, 221 Pages.
[27] G.W. Flake. The computational beauty of nature: Computer explorations of fractals, chaos, complex systems, and adaptation. MIT press, 2000.
[28] J. M. Gutiérrez and A. Iglesias, (1998) “Mathematica package for analysis and control of chaos in nonlinear systems" Computers in Physics 12(6): 608–619.
[29] Wolfram, Stephen and Mallinckrodt, A John, (1995) “Cellular automata and complexity" Computers in Physics 9(1): 55–55.
[30] A. T. Layton, M. Bowen, A. Wen, and H. E. Layton, (2011) “Feedback-mediated dynamics in a model of coupled nephrons with compliant thick ascending limbs" Mathematical biosciences 230(2): 115–127.