Anjan Biswas 1,2,3,4, Yakup Yıldırım5, Mehmet Ekici6, Padmaja Guggilla1, Salam Khan1, O. González-Gaxiola7, Abdullah Khamis Alzahrani2, and Milivoj R. Belic8
1Department of Physics, Chemistry and Mathematics, Alabama A&M University, Normal, AL 35762-4900, USA
2Department of Mathematics, King Abdulaziz University, Jeddah-21589, Saudi Arabia
3Department of Applied Mathematics, National Research Nuclear University, 31 Kashirskoe Hwy, Moscow-115409, Russian Federation
4Department of Mathematics and Applied Mathematics„ Sefako Makgatho Health Sciences University, Medunsa–0204, South Africa
5Department of Mathematics, Faculty of Arts and Sciences„ Near East University, 99138 Nicosia, Cyprus
6Department of Mathematics, Faculty of Science and Arts, Yozgat Bozok University, 66100 Yozgat, Turkey
7Departamento de Matemáticas Aplicadas y Sistemas, Universidad Autónoma Metropolitana-Cuajimalpa. , Vasco de Quiroga 4871, 05348 Mexico City, Mexico
8Institute of Physics Belgrade, Pregrevica 118, 11080 Zemun, Serbia
Received:
December 9, 2020
Accepted:
December 20, 2020
Publication Date:
June 23, 2021
Copyright The Author(s). This is an open access article distributed under the terms of the Creative Commons Attribution License (CC BY 4.0), which permits unrestricted use, distribution, and reproduction in any medium, provided the original author and source are cited.
Download Citation: ||https://doi.org/10.6180/jase.202112_24(6).0014
ABSTRACT
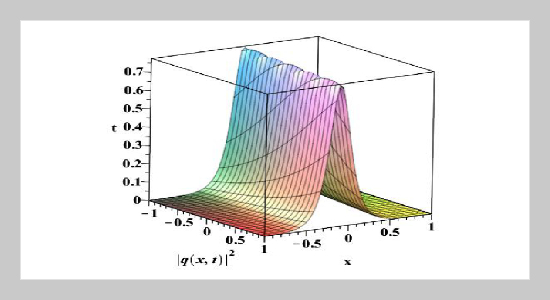
This paper secures a spectrum of cubic–quartic optical solitons for perturbed complex Ginzburg–Landau equation. There are eight powerful and prolific integration structures that made this retrieval possible. The perturbation terms are all of Hamiltonian type and are with maximum intensity. The existence criteria for such solitons naturally emerged from their respective parameter dynamics. As a byproduct, these schemes revealed periodic singular solutions.
OCIS Codes: 060.2310; 060.4510; 060.5530; 190.3270; 190.4370
Keywords:
solitons; perturbation; Ginzburg–Landau; non–Kerr law
REFERENCES
- [1] M. A. Abdou, A. A. Soliman, A. Biswas, M. Ekici, Q. Zhou & S.P. Moshokoa. “Dark–singular combo optical solitons with fractional complex Ginzburg–Landau equation". Optik. Volume 171, 463–467. (2018).
- [2] G. Akram, N. Mahak, Application of the first integral method for solving (1+1)–dimensional cubic–quintic complex Ginzburg–Landau equation". Optik. Volume 164, 210–217. (2018).
- [3] A. H. Arnous, A. R. Seadawy, R. T. Alqahtani & A. Biswas. “Optical solitons with complex Ginzburg–Landau equation by modified simple equation method" Optik. Volume 144, 475–480. (2017).
- [4] S. Arshed. “Soliton solutions of fractional complex Ginzburg–Landau equation with Kerr law and non–Kerr law media". Optik. Volume 160, 322–332. (2018).
- [5] S. Arshed, A. Biswas, F. Mallawi & M. R. Belic. “Optical solitons with complex Ginzburg–Landau equation having three nonlinear forms". Physics Letters A. Volume 383, Issue 36, 126026. (2019).
- [6] A. Biswas. “Chirp–free bright optical solitons and conservation laws for complex Ginzburg–Landau equation with three nonlinear forms". Optik. Volume 174, 207–215. (2018).
- [7] A. Biswas. “Temporal 1–soliton solution of the complex Ginzburg–Landau equation with power law nonlinearity". Progress in Electromagnetics Research. Volume 96, 1–7. (2009).
- [8] A. Biswas & R. T. Alqahtani. “Optical soliton perturbation with complex Ginzburg–Landau equation by semi–inverse variational principle". Optik. Volume 147, 77–81. (2017).
- [9] A. Biswas, Y. Yildirim, E. Yasar, H. Triki, A. S. Alshomrani, M. Z. Ullah, Q. Zhou, S. P. Moshokoa & M. Belic. “Optical soliton perturbation with complex Ginzburg–Landau equation using trial solution approach". Optik. Volume 160, 44–60. (2018).
- [10] A. Biswas, Y. Yildirim, E. Yasar, H. Triki, A. S. Alshomrani, M. Z. Ullah, Q. Zhou, S. P. Moshokoa & M. Belic. “Optical soliton perturbation for complex Ginzburg–Landau equation with modified simple equation method". Optik. Volume 158, 399–415. (2018).
- [11] M. Mirzazadeh, M. Ekici, A. Sonmezoglu, M. Eslami, Q. Zhou, A. H. Kara, D. Milovic, F. B. Majid, A. Biswas & M. Belic. “Optical solitons with complex Ginzburg–Landau equation". Nonlinear Dynamics. Volume 85, Issue 3, 1979–2016. (2016).
- [12] S. Naghshband & M. A. F. Araghi. “Solving generalized quintic complex Ginzburg–Landau equation by homotopy analysis method". Ain Shams Engineering Journal. Volume 9, Issue 4, 607–613. (2018).
- [13] M. S. Osman. “On complex wave solutions governed by the 2D Ginzburg–Landau equation with variable coefficients". Optik. Volume 156, 169–174. (2018).
- [14] S. Shwetanshumala. “Temporal solitons of modified complex Ginzberg–Landau equation". Progress In Electromagnetics Research Letters. Volume 3, 17–24. (2008).
- [15] H. Triki, S. Crutcher, A. Yildirim, T. Hayat, O.M. Aldossary & A. Biswas. “ Bright and dark solitons of the modified complex Ginzburg–Landau equation with parabolic and dual–power law nonlinearity". Romanian Reports in Physics. Volume 64, Issue 2, 367–380. (2012).
- [16] Y. Yan & W. Liu. “Stable transmission of solitons in the complex cubic–quintic Ginzburg–Landau equation with nonlinear gain and higher–order effects". Applied Mathematics Letters. Volume 98, 171–176. (2019).
- [17] E. M. E. Zayed, M. E. M. Alngar, M. El–Horbaty, A. Biswas, A. S. Alshomrani, M. Ekici, Y. Yildirm & M. R. Belic. “Optical solitons with complex Ginzburg–Landau equation having a plethora of nonlinear forms with a couple of improved integration norms". Optik. Volume 207, 163804. (2020).
- [18] Y. Zhao, C–Y Xia & H–B Zeng. “Cascade replication of soliton solutions in the one-dimensional complex cubic–quintic Ginzburg–Landau equation". Physics Letters A. Volume 384, Issue 18, 126395. (2020).
- [19] E. M. E. Zayed, M. E. M. Alngar, A. Biswas, S. Khan, M. Ekici, L. Moraru & A. S. Alshomrani. “Pure–cubic optical soliton perturbation with complex Ginzburg–Landau equation having a dozen of nonlinear refractive index structures". Journal of Communications Technology and Engineering. Volume 66, Issue 5, 481–544. (2021).
- [20] M. Zhang, A. Zheng, Q. Chen & J. Liu. “Nitrogenvacancy defects induced bright, dark, and Ginzburg–Landau phonon solitons in cavity arrays". Optik. Volume 218, 165255. (2020).