Mohamed Gibigaye1, Crespin Prudence Yabi2, Ezéchiel I. Alloba2, Ehsan Noroozinejad Farsangi 3, Gerard Degan2
1University of Abomey-Calavi 01BP 2009, Cotonou, Benin
2National University of Sciences, Technologies, Engineering and Mathematics of Abomey, Benin
3Faculty of Civil and Surveying Engineering, Graduate University of Advanced Technology, Iran
Received:
March 24, 2020
Accepted:
April 24, 2020
Publication Date:
September 1, 2020
Download Citation:
||https://doi.org/10.6180/jase.202009_23(3).0008
ABSTRACT
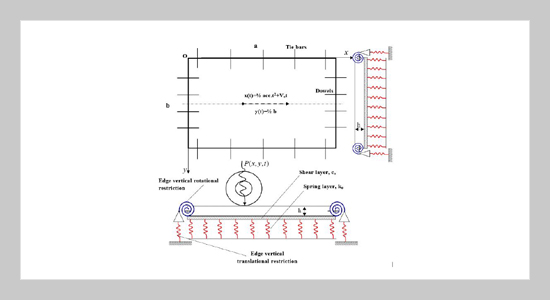
The static/dynamic analysis of the thin plate is of great importance in engineering problems. Based on the complexity of the problem, only for some important cases of boundary conditions, analytical solutions were derived. While in general case, numerical approaches should be implemented to solve the problem. The discrete singular convolution (DSC) technique is one of the most accurate methods. Until now, this method is difficult to be used for the determination of the static and dynamic responses for plates with semi-rigid boundary conditions. Based on the fact that DSC method using the Taylor approach (DSC-T) is recently used for the analysis of the free vibration of free and semi-rigid edges rectangular plate, this study aims to extend the application of the DSC-T methods to the static and dynamic analysis of the thin rectangular plate restrained with the dowels and resting on elastic foundation. The DSC-T approach is extended to the resolution of the static case of a dowelled plate, and with the combination of the Newmark scheme, to the case of the dynamic load. The results showed the applicability of the studied method for the determination of static and dynamic responses of the plates. Besides, it is shown that there is a linear relationship between the value of the depth and the decrement of the modified Vlasov soil. The value of the logarithmic decrement of the soil is highly influenced by the thickness of the plate namely for membranes and very thin plates. The DSC-T method could be recommended for the engineering design of a variety of civil structures.
Keywords:
DSC; Newmark scheme; Rigid pavement; Boundary conditions; Elastic foundation; Thin isotropic plate; Taylor series expansion.
REFERENCES
- [1] O. Civalek, Nonlinear analysis of thin rectangular plates on Winkler – Pasternak elastic foundations by DSC – HDQ methods, Appl. Math. Model. 31 (2007) 606–624. doi:10.1016/j.apm.2005.11.023.
- [2] M. Gibigaye, C.P. Yabi, G. Degan, Free vibration analysis of dowelled rectangular isotropic thin plate on a Modified Vlasov soil type by using discrete singular convolution method, Appl. Math. Model. 61 (2018) 618–633. doi:10.1016/j.apm.2018.05.019.
- [3] K. Ozgan, A.T. Daloglu, Dynamic analysis of thick plates on elastic foundations using Winkler foundation model, Sci. Iran. A. 19 (2013) 29–41. doi:10.3233/SAV-2012-0723.
- [4] M. Gibigaye, C.P. Yabi, I.E. Alloba, Dynamic Response of a Rigid Pavement Plate Based on an Inertial Soil, Int. Sch. Res. Not. 2016 (2016) 1–9. doi:10.1155/2016/4975345.
- [5] S.W. Alisjahbana, W. Wangsadinata, Dynamic analysis of rigid roadway pavement under moving traffic loads with variable velocity, Interact. Multiscale Mech. 5 (2012) 105–114. doi:10.12989/imm.2012.5.2.105.
- [6] X. Wang, Z. Yuan, Discrete singular convolution and Taylor series expansion method for free vibration analysis of beams and rectangular plates with free boundaries, Int. J. Mech. Sci. 122 (2017) 184–191. doi:10.1016/j.ijmecsci.2017.01.023.
- [7] Ö.C.K.M.B.A. Ciğdem Demir, Frequencies Values of Orthotropic Composite Circular and Annular Plates, 9 (2017) 55–65. doi:10.24107/ijeas.309060.
- [8] O. Civalek, Fundamental frequency of isotropic and orthotropic rectangular plates with linearly varying thickness by discrete singular convolution method, Appl. Math. Model. 33 (2009) 3825–3835. doi:10.1016/j.apm.2008.12.019.
- [9] S.W. Alisjahbana, I. Alisjahabana, S. Kiryu, B.S. Gan, Semi analytical solution of a rigid pavement under a moving load on a Kerr foundation model Semi analytical solution of a rigid pavement under a moving load on a Kerr foundation model, (2018). doi:10.21595/jve.2018.20082.
- [10] H. Khov, W.L. Li, R.F. Gibson, An accurate solution method for the static and dynamic deflections of orthotropic plates with general boundary conditions, Compos. Struct. 90 (2009) 474–481. doi:10.1016/j.compstruct.2009.04.020.
- [11] Q. Zhu, X. Wang, Free vibration analysis of thin isotropic and anisotropic rectangular plates by the discrete singular convolution algorithm, Int. J. Numer. Methods Eng. 86 (2011) 782–800. doi:10.1002/nme.
- [12] Y.B. Zhao, G.W. Wei, DSC Analysis of Rectangular Plates With Non-Uniform Boundary Conditions, J. Sound Vib. 255 (2002) 203–228. doi:10.1006/jsvi.2001.4150.
- [13] X. Wang, S. Xu, Free vibration analysis of beams and rectangular plates with free edges by the discrete singular convolution, J. Sound Vib. 329 (2010) 1780–1792. doi:10.1016/j.jsv.2009.12.006.
- [14] C. Shu, Q. Yao, K.S. Yeo, Block-marching in time with DQ discretization: An efficient method for time-dependent problems, Comput. Methods Appl. Mech. Eng. 191 (2002) 4587–4597. doi:10.1016/S0045-7825(02)00387-0.
- [15] O. Civalek, A. Yavas, Large Deflection Static Analysis of Rectangular Plates On Two Parameter Elastic Foundations, Int. J. Sci. Technol. 1 (2006) 43–50. doi:10.1.1.530.5332.
- [16] S. Zhao, G.W. Wei, Y. Xiang, DSC analysis of free-edged beams by an iteratively matched boundary method, J. Sound Vib. 284 (2005) 487–493. doi:10.1016/j.jsv.2004.08.037.
- [17] S.A. Eftekhari, A.A. Jafari, Vibration of an initially stressed rectangular plate due to an accelerated traveling mass, Sci. Iran. 19 (2012) 1195–1213. doi:10.1016/j.scient.2012.07.008.
- [18] S.A. Eftekhari, A modified differential quadrature procedure for numerical solution of moving load problem, 0 (2015) 1–17. doi:10.1177/0954406215584630.
- [19] S.A. Eftekhari, A note on mathematical treatment of the Dirac-delta function in the differential quadrature bending and forced vibration analysis of beams and rectangular plates, Appl. Math. Model. (2015). doi:10.1016/j.apm.2015.01.063.
- [20] S.A. Eftekhari, A.A. Jafari, Numerical simulation of chaotic dynamical systems by the method of differential quadrature, Sci. Iran. 19 (2012) 1299–1315. doi:10.1016/j.scient.2012.08.003.
- [21] S.A. Eftekhari, Young, A Differential Quadrature Procedure with Regularization of the Dirac-delta Function for Numerical Solution of Moving Load Problem, Lat. Am. J. Solids Struct. (2014) 1241–1265.
- [22] S.Y. Yang, Y.C. Zhou, G.W. Wei, Comparison of the discrete singular convolution algorithm and the Fourier pseudospectral method for solving partial differential equations, Comput. Phys. Commun. 143 (2002) 113–135. doi:10.1016/S0010-4655(01)00427-1.
- [23] Y.M. Xie, An assessment of time integration schemes for non-linear dynamic equations, J. Sound Vib. 192 (1996) 321–331. doi:10.1006/jsvi.1996.0190.
- [24] C. Runge, Ueber die numerische Auflösung von Differentialgleichungen, Math. Ann. 46 (1895) 167–178. doi:10.1007/BF01446807.
- [25] V. Nguyen, Comportement dynamique de structures non-linéaires soumises à des charges mobiles, Ecole Nationale des Ponts et Chaussées, 2002.
- [26] N.M. Newmark, A Method of Computation for Structural Dynamics, J. Eng. Mech. 85 (1959) 67–94. doi:0.1016/j.compgeo.2015.08.008.
- [27] A. Ben-Israel, A Newton-Raphson method for the solution of systems of equations, J. Math. Anal. Appl. 15 (1966) 243–252. doi:10.1016/0022-247X(66)90115-6.
- [28] W.T. Straughan, Analysis of plates on elastic foundations, Texas Technologie University, 1990.
- [29] X. Wang, Y. Wang, S. Xu, DSC analysis of a simply supported anisotropic rectangular plate, Compos. Struct. 94 (2012) 2576–2584. doi:10.1016/j.compstruct.2012.03.005.
- [30] G.W. Wei, Discrete singular convolution for the solution of the Fokker–Planck equation, J. Chem. Phys. 110 (1999) 8930–8942. doi:10.1063/1.478812.
- [31] G.W. Wei, Y.B. Zhao, Y. Xiang, Discrete singular convolution and its application to the analysis of plates with internal supports.Part 1: Theory and algorithm, Int. J. Numer. Methods Eng. 55 (2002) 913–946. doi:10.1002/nme.527.
- [32] G. Duan, X. Wang, Vibration analysis of stepped rectangular plates by the discrete singular convolution algorithm, Int. J. Mech. Sci. 82 (2014) 100–109. doi:10.1016/j.ijmecsci.2014.03.004.
- [33] C.W. Bert, X. Wang, A.G. Striz, Differential quadrature for static and free vibration analyses of anisotropic plates, Int. J. Solids Struct. 30 (1993) 1737–1744. doi:10.1016/0020-7683(93)90230-5.
- [34] M. Géradin, D.J. Rixen, Mechanical Vibrations: Theory and Application to Structural Dynamics, 2015.
- [35] K.L. Nguyen, Q.T. Tran, L. Manin, S. Baguet, M. Andrianoely, Un schéma d’intégration temporelle pour la réponse transitoire de systèmes mécaniques avec butées de contact, (2017).
- [36] SETRA, LCPC, Conception et dimensionnement des structures de chaussée: guide technique, 1994.
- [37] A. Turhan, A Consistent Vlasov Model for Analysis of Plates on Elastic Foundations Using the Finite Element Method, Graduate Faculty of Texas Tech University, 1992. https://ttu-ir.tdl.org/ttu-ir/bitstream/.../31295007142515.pdf?...1.
- [38] NCHRP, Guide for Mechanistic-Empirical Design OF NEW AND REHABILITATED PAVEMENT STRUCTURES, ARA, Inc., ERES Division 505 West University Avenue Champaign, Illinois 61820, 2003.
- [39] C. Kneifati, Analysis of Plates on a Kerr Foundation, I (1985) 1325–1342.
- [40] Liu D, Chen Y. Eigenvalue analysis of thin plate with complicated shapes by a novel infinite element method. Computer Modeling in Engineering & Sciences. 2019 Jan 1;120(2):273-92.
- [41] Qiu Z, Lu J, Elgamal A, Su L, Wang N, Almutairi A. OpenSees three-dimensional computational modeling of ground-structure systems and liquefaction scenarios. Computer Modeling in Engineering & Sciences. 2019 Jan 1;120(3):629-56.
- [42] Ma Z, Kong L, Jin X. An Explicit-Implicit Mixed Staggered Asynchronous Step Integration Algorithm in Structural Dynamics. Computer Modeling in Engineering & Sciences. 2018 Jul 1;116(1):51-67.
- [43] Lu, H., Gao, Z., Xu, L., & Wu, B. (2018). Effects of the Convex Topography on Railway Environmental Vibrations. Computer Modeling in Engineering & Sciences, 118(1), 177-205.
- [44] Chang DW, Lee MR, Hong MY, Wang YC. A simplified modeling for seismic responses of rectangular foundation on piles subjected to horizontal earthquakes. Journal of GeoEngineering. 2016 Dec 1;11(3):109-22.
- [45] Chang DW, Lien HW, Wang T. Finite difference analysis of vertically loaded raft foundation based on the plate theory with boundary concern. Journal of GeoEngineering. 2018 Sep 1;13(3):135-47.