Rahul P. Mehta 1 and Hari R. Kataria2
1Applied Science Humanities Department, Sardar Vallabhbhai Patel Institute of Technology, Vasad, India
2Department of Mathematics, Faculty of Science, The M. S. University of Baroda, Vadodara, India
Received:
March 14, 2019
Accepted:
June 17, 2019
Publication Date:
June 1, 2020
Download Citation:
||https://doi.org/10.6180/jase.202006_23(2).0015
ABSTRACT
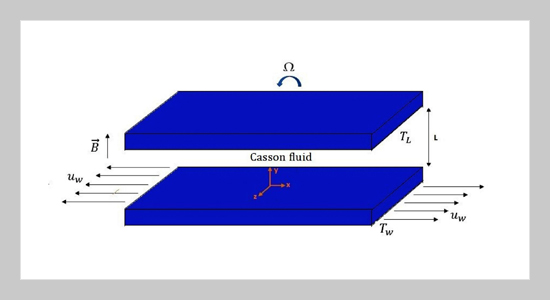
This article deals with three dimensional MHD flow of Casson fluid past between horizontal plates. Here the considered fluid is conducting which passes through medium which has porosity. Problem is modeled as a system of PDE with some initial conditions and boundary conditions. System under consideration is rotating. Some variables are transformed and converted to dimensionless. The system is then transformed to ordinary differential equations with corresponding initial conditions and boundary conditions. This system of equations with initial conditions and boundary conditions are solved using method of Homotopy Analysis. Expressions for fluid velocity (in all three directions), temperature and concentration profiles are obtained. The features of the velocity, temperature and concentration are analyzed by plotting graphs and the physical aspects are studied for different parameters like the magnetic field parameter, Casson fluid parameter, radiation parameter, rotation parameter and time.
Keywords:
HAM; MHD; Casson fluid; free Covection; Porous Medium.
REFERENCES
- [1]Casson, (1959): A flow equation for the pigment oil suspensions of the printing ink type, in: Rheology of Disperse Systems, Pergamon, NewYork, 84-102.
- [2]Mahanta, S. Shaw, (2015): 3D Casson fluid flow past a porous linearly stretching sheet with convective boundary condition Alexandria Engineering Journal, 54 (3) 653–659.
- [3]Hartmann, (1937): Hg-dynamics I theory of the laminar flow of an electrically conductive liquid in a homogenous magnetic field, Det Kal. Danske Videnskabernes selskab, Mathematisk-fysiske Meddeleser, 15 1-27.
- [4]Nadeem, R. U. Haq, N. S. Akbar, Z.H. Khan, (2013): MHD three-dimensional Casson fluid flow past a porous linearly stretching sheet, Alexandria Engineering Journal,52 577–582.
- [5]R. Kataria, H. R. Patel, (2015): Effect of magnetic field on unsteady natural convective flow of a micropolar fluid between two vertical walls , Ain Shams Engineering Journal, doi. 10.1016/j.asej.2015.08.013.
- [6]M. Rashidi, E. Erfani, (2012): Analytical Method for Solving Steady MHD Convective and Slip Flow due to a Rotating Disk with Viscous Dissipation and Ohmic Heating, Engineering Computations 29 (6) 562–579.
- [7]Hatami and H. Safari, (2016): "Effect of inside heated cylinder on the natural convection heat transfer of nanofluids in a wavy-wall enclosure." International Journal of Heat and Mass Transfer 103 1053-1057.
- [8]Hatami, D. Song, and D. Jing, (2016): "Optimization of a circular-wavy cavity filled by nanofluid under the natural convection heat transfer condition." International Journal of Heat and Mass Transfer 98 758-767.
- [9]Hatami, M. Sheikholeslami, M. Hosseini, D. D. Ganji, (2014): Analytical investigation of MHD nanofluid flow in non-parallel walls, Journal of Molecular Liquids 194 251–259.
- [10]Sheikholeslami, H. F. Oztop, MHD free convection of nanofluid in a cavity with sinusoidal walls by using CVFEM, Chinese Journal of Physics, 55 (2017) 2291-2304.
- [11]Selimefendigil, H. F. Öztop, conjugate natural convection in a nanofluid filled partitioned horizontal annulus formed by two isothermal cylinder surfaces under magnetic field, International Journal of Heat and Mass Transfer, 108 (2017) 156–171
- [12]Selimefendigil, Hakan F. Öztopb, A. J. Chamkhac, Fluid–structure-magnetic field interaction in a nanofluid filled lid-driven cavity with flexible side wall, European Journal of Mechanics B/Fluids, 61 (2017) 77–85.
- [13]H M Sedighi, K H Shirazi, J Zare, An analytic solution of transversal oscillation of quintic non-linear beam with homotopy analysis method, International Journal of Non-Linear Mechanics 47 (2012), 777-784
- [14]H M Sedighi, K H Shirazi, Using homotopy analysis method to determine profile for disk cam by means of optimization of dissipated energy, International Review of Mechanical Engineering 5 (2018), 941-946
- [15]H M Sedighi, F Daneshmand, Nonlinear transversely vibrating beams by the homotopy perturbation method with an auxiliary term, Journal of Applied and Computational Mechanics 1 (2014), 1-9
- [16]A Reza, H M Sedighi, Nonlinear vertical vibration of tension leg platforms with homotopy analysis method, Advances in Applied Mathematics and Mechanics 7 (2015), 357-368
- [17]U Filobello-Nino, H Vazquez-Leal, MM Rashidi, Hamid M Sedighi, A Perez-Sesma, M Sandoval-Hernandez, A Sarmiento-Reyes, AD Contreras-Hernandez, D Pereyra-Diaz, C Hoyos-Reyes, VM Jimenez-Fernandez, J Huerta-Chua, F Castro-Gonzalez, JR Laguna-Camacho, Laplace transform homotopy perturbation method for the approximation of variational problems, SpringerPlus 5 (2016), 276.
- [18]R. Kataria, A. S. Mittal, (2015): Mathematical model for velocity and temperature of gravity-driven convective optically thick nanofluid flow past an oscillating vertical plate in presence of magnetic field and radiation. Journal of Nigerian Mathematical Society, 34 303–317.
- [19]R. Kataria, A. S. Mittal, (2017): Velocity, mass and temperature analysis of gravity-driven convection nanofluid flow past an oscillating vertical plate in presence of magnetic field in a porous medium, Applied Thermal Engineering, 110 864-874.
- [20]Freidoonimehr, M. M. Rashidi, S. Mahmud, (2015): Unsteady MHD free convective flow past a permeable stretching vertical surface in a nano-fluid, International Journal of Thermal Sciences, 87 136-145.
- [21]Sheikholeslami, M. M. Bhatti, (2017): Active method for nanofluid heat transfer enhancement by means of EHD, International Journal of Heat and Mass Transfer 109 115–122.
- [22]Sheikholeslami, S.A. Shehzad, (2017): Thermal radiation of ferrofluid in existence of Lorentz forces considering variable viscosity, International Journal of Heat and Mass Transfer 109 82–92.
- [23]M. Rashidi, M. Ali, N. Freidoonimehr, B. Rostami, M. Anwar Hossain, (2014): Mixed convective heat transfer for MHD viscoelastic fluid flow over a porous wedge with thermal radiation, Advances in Mechanical Engineering, Volume 2014 Article number 735939.
- [24]I. Khan, S. Qayyum, T. Hayat, M. Waqas, M. I. Khan, A. Alsaedi, Entropy generation minimization and binary chemical reaction with Arrhenius activation energy in MHD radiative flow of nanomaterial, doi: 10.1016/j.molliq.2018.03.049
- [25]I. Khan, M. I. Khan, M. Waqas, T. Hayat, A. Alsaedi, Chemically reactive flow of Maxwell liquid due to variable thicked surface, International Communications in Heat and Mass Transfer, 86 (2017) 231–238.
- [26]A. Shehzad, T. Hayat, A. Alsaedi, (2016): Three-Dimensional MHD Flow of Casson Fluid in Porous Medium with Heat Generation, Journal of Applied Fluid Mechanics, 9 215-223.
- [27]M. Hussain, J. Jain, G.S. Seth, M.M. Rashidi, (2017): Free convective heat transfers with hall effects, heat absorption and chemical reaction over an accelerated moving plate in a rotating system, Journal of Magnetism and Magnetic Materials, 422 112–123
- [28]Nandkeolyar, M. Das, P. Sibanda, (2013): Exact solutions of unsteady MHD free convection in a heat absorbing fluid flow past a flat plate with ramped wall temperature, Boundary Value Problems, 2013:247
- [29]R. Kataria, H. R. Patel, (2016): Heat and Mass Transfer in MHD Second Grade Fluid Flow with Ramped Wall Temperature through Porous Medium, Mathematics Today, 32 67-83
- [30]R. Kataria, H. R. Patel, (2016): Effect of thermo-diffusion and parabolic motion on MHD Second grade fluid flow with ramped wall temperature and ramped surface concentration, Alexandria Engineering Journal, 10.1016/j.aej.2016.11.014
- [31]S. Seth, S. M. Hussain, S. Sarkar, (2015): Hydromagnetic natural convection flow with heat and mass transfer of a chemically reacting and heat Absorbing fluid past an accelerated moving vertical plate with ramped temperature and ramped surface Concentration through a porous medium, Journal of the Egyptian Mathematical Society 23 197–207
- [32]Kumaran, N. Sandeep, (2017): Thermophoresis and Brownian moment effects on parabolic flow of MHD Casson and Williamson fluids with cross diffusion, Journal of Molecular Liquids, 233 262–269.
- [33]Sandeep, O. K. Koriko, I. L. Animasaun, (2016): Modified kinematic viscosity model for 3D-Casson fluid flow within boundary layer formed on a surface at absolute zero, Journal of Molecular Liquids, 221 1197–1206
- [34]R. Kataria, H. R. Patel, (2016): Radiation and chemical reaction effects on MHD Casson fluid flow past an oscillating vertical plate embedded in porous medium, Alexandria Engineering Journal, 55 583–595
- [35]R. Kataria, H. R. Patel, (2016): soret and heat generation effects on MHD Casson fluid flow past an oscillating vertical plate embedded through porous medium, Alexandria Engineering Journal 55 2125–2137
- [36]K. Anantha, R. J. V. Ramana, N. Sandeep, V. Sugunamma, (2016): Dual Solutions for Thermo Diffusion and Diffusion Thermo Effects on 3D MHD Casson Fluid Flow over a Stretching Surface, R.J.Pharmacy and tech. 9(8), 1187-1194
- [37]Sulochana, S. S. Payad, N. Sandeep, (2016): Non uniform heat source or sink effect on the flow of 3D Casson fluid in presence of Soret and thermal radiation, Int.J.Eng. Resaech in Afrika, 20 112-129.
- [38]I. Khan, M. Waqas, T. Hayat, A. Alsaedi, (2017): A comparative study of Casson fluid with homogeneous-heterogeneous reactions, Journal of Colloid and Interface Science 85-90
- [39]Sheikholeslami, T. Hayat, A. Alsaedi, (2017): Numerical study for external magnetic source influence on water based nanofluid convective heat transfer, International Journal of Heat and Mass Transfer, 745-755.
- [40]K. Nayak, G. C. Dash, L. P. Singh, (2014): Steady MHD flow and heat transfer of a third grade fluid in wire coating analysis with temperature dependent viscosity, Int. J. Heat Mass Transfer, 79 (2014) 1087–1095.
- [41]Hayat, T. Muhammad, A. Qayyum, A. Alsaedi, M. Mustafa, (2016): On squeezing flow of nanofluid in the presence of magnetic field effects. J. Mol. Liquids, 179-185.
- [42]Hayat, M. Shafique, A. Tanveer, A. Alsaedi, (2016): Magnetohydrodynamic effects on peristaltic flow of hyperbolic tangent nanofluid with slip conditions and Joule heating in an inclined channel, Int. J. Heat Mass Transfer, 102 54–63.
- [43]R. Kataria, A. S. Mittal, (2017): Analysis of Casson Nanofluid Flow in Presence of Magnetic Field and Radiation, Mathematics Today, 33(1), 99 - 120.
- [44]Rosseland, (1931): Astrophysik und atom-theoretische Grundlagen. Berlin: Springer-Verlag.
- [45]J. Liao, (2003): Beyond Perturbation: Introduction to Homotopy Analysis Method, Chapman and Hall/CRC Press, Boca Raton.