1Department of Mathematics, I.K. Gujral Punjab Technical University, Jalandhar, Punjab, India
Received:
October 14, 2016
Accepted:
April 13, 2017
Publication Date:
September 1, 2017
Download Citation:
||https://doi.org/10.6180/jase.2017.20.3.09
ABSTRACT
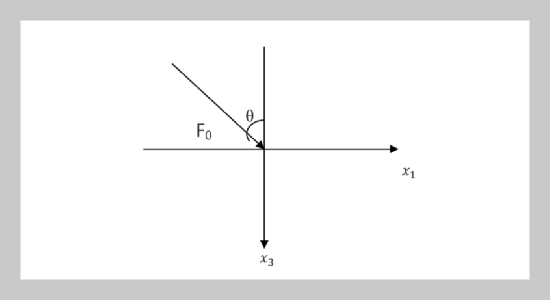
The study of present paper deals with two dimensional problem in magneto-microstretch thermoelastic medium in the presence of combined effects of Hall current and rotation. The microstretch theory of thermoelasticity with two relaxation times derived by Eringen has been used to investigate the problem. The problem is solved to obtain displacement, stress components, current density components and temperature distribution. Numerical computed results of all the considered variables have been shown graphically to depict the combined effect of Hall current and rotation. Some particular cases of interest are also deduced from the present study.
Keywords:
Microstretch Thermoelastic, Hall Current, Rotation, Current Density Vector
REFERENCES
- [1] Eringen, A. C., “Theory of Thermomicrostretch Elastic Solids,” International Journal of Engineering Science, Vol. 28, No. 12, pp. 1291–1301 (1990).
- [2] Cicco, S. D., “Stress Concentration Effects in Microstretch Elastic Bodies,” International Journal of Engineering Sciences, Vol. 41, pp. 187199 (2003).
- [3] Ciarletta, M. and Scalia, A., “Some Results in Linear Theory of Thermomicrostretch Elastic Solids,” Meccanica, Vol. 39, pp. 191–206 (2004).
- [4] Iesan, D. and Quintanilla, R., “Thermal Stresses in Microstretch Elastic Plates,” Int. J. Eng. Sci., Vol. 43, pp. 885–907 (2005). doi: 10.1016/j.ijengsci.2005.03.005
- [5] Aouadi, M., “Thermomechanical Interactions in a Generalized Thermomicrostretch Elastic Half Space,” Journal of Thermal Stresses, Vol. 29, pp. 511528 (2006). doi: 10.1080/01495730500373495
- [6] Marin, M., “A Domain of Influence Theorem for Microstretch Elastic Materials,” Nonlinear Analysis, Real World Applications, Vol. 11, pp. 34463452 (2010). doi: 10.1016/j.nonrwa.2009.12.005
- [7] Marin, M., “Apartition of Energy in Thermoelasticity of Microstretch Bodies,” Nonlinear Anal., Real World Appl., Vol. 11, pp. 2436–2447 (2010a). doi: 10.1016/ j.nonrwa.2009.07.014
- [8] Marin, M., “Lagrange Identity Method for Microstretch Thermoelastic Materials,” J. Math. Anal. Appl., Vol. 363, pp. 275–286 (2010b). doi: 10.1016/j.jmaa. 2009.08.045
- [9] Passarella, F. and Tibullo, V., “Some Results in Linear Theory of Thermoelasticity Backward in Time for Microstretch Materials,” J. Therm. Stress, Vol. 33, pp. 559–576 (2010). doi: 10.1080/01495731003772811
- [10] Kumar, R. and Kumar, A., “Elastodynamic Response Due to Mechanical Forces in a Microstretch Thermoelastic Medium with Mass Diffusion,” Material Physics and Mechanics, Vol. 22, pp. 4452 (2015).
- [11] Kumar, R., Kumar, A. and Singh, D., “Thermomechanical Interactions of Laser Pulse with Microstretch Thermoelastic Medium,” Archives of Mechanics, Vol. 67, No. 6, pp. 439456 (2015).
- [12] Gad, N. S., “Effects of Hall Currents on Peristaltic Transport with Compliant Walls,” Applied Mathematics and Computation, Vol. 235, pp. 546554 (2014). doi: 10.1016/j.amc.2014.02.081
- [13] Ezzat, M. A. and Awad, E. S., “Micropolar Generalized Magneto-thermoelasticity with Modified Ohm’s and Fourier’s Laws,” J. Math. Anal. Appl., Vol. 353, pp. 99113 (2009). doi: 10.1016/j.jmaa.2008.11.058
- [14] Zakaria, M., “Effects of Hall Current and Rotationon Magneto-micropolar Generalized Thermoelasticity Due to Ramp-type Heating,” International Journal of Electromagnetics and Applications, Vol. 2, No. 3, pp. 24 32 (2012). doi: 10.5923/j.ijea.20120203.02
- [15] Eringen, A. C., Microcontinuum Field Theories I: Foundations and Solids, Springer-Verleg, New York (1999).
- [16] Eringen, A. C., “Plane Waves in Non-local Micropolar Elasticity,” Int. J. Eng. Sci., Vol. 22, pp. 1113–1121 (1984). doi: 10.1016/0020-7225(84)90112-5
- [17] Dhaliwal, R. S. and Singh, A., Dynamic Coupled Thermoelasticity, Hindustan Publication Corporation, New Delhi (1980).