Deqian Zheng This email address is being protected from spambots. You need JavaScript enabled to view it.1,2, Ming Gu1 , Aishe Zhang3 , Yanjie Xie2 , Beibei Huang2 and Haochen Hu2
1State Key Laboratory for Disaster Reduction in Civil Engineering, Tongji University, Shanghai 200092, P.R. China 2School of Civil Engineering and Architecture, Henan University of Technology, Zhengzhou 450001, P.R. China 3School of Civil Engineering, Shandong Jianzhu University, Ji’nan 250101, P.R. China
Received: October 28, 2016 Accepted: February 15, 2017 Publication Date: September 1, 2017
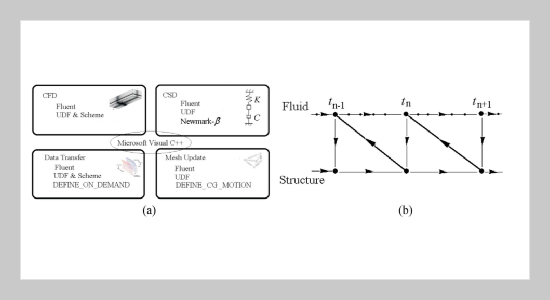
Wind-induced transverse vibration of a two-dimensional square cylinder was numerically simulated, based on commercial code Fluent. The Reynolds number, defined by inflow velocity and the depth of the cylinder, was set to be 22000. The fluid-structure coupled system was solved by employing partitioned coupling scheme. The fluid field was simulated using SST k- turbulence model, and the structural motion was calculated by Newmark method. The solution procedure was programmed by user define function (UDF). Flow around the cylinder at stationary state was firstly simulated to obtain initial flow field condition for the coupled system. Wind-induced transverse vibration of the cylinder was then simulated at different reduced wind velocities. Wind-induced galloping, the beat-phenomena and vortex-excited resonance of the cylinder in the transverse direction, were all captured, with the increase of the reduced wind velocities. The simulated data were also compared with those of previous studies. The comparison results showed that the present method is applicable in solving wind-induced vibration problems. Finally, parametric analysis of Scruton number’s effect on the across-wind vibrations of the cylinder was investigated. The results indicated that wind-induced vibration of the cylinder was remarkably affected by the Scruton number. The transverse vibrations were obviously divided into galloping and vortex-induced vibration at large Scruton number, while the switch between the two vibration types was not so remarkable when the Scruton number was low.
[1] Braun, A. L. and Awruch, A. M., “Aerodynamic and Aeroelastic Analyses on the CAARC Standard Tall Building Model Using Numerical Simulation,” Computers & Structures, Vol. 87, No. 910, pp. 564581 (2009). doi: 10.1016/j.compstruc.2009.02.002
[2] Farhat, C., Rallu, A., Wang, K., et al., “Robust and Provably Second-order Explicit-explicit and Implicitexplicit Staggered Time-integrators for Highly Nonlinear Compressible Fluid-structure Interaction Problems,” International Journal for Numerical Methods in Engineering, Vol. 84, No. 1, pp. 73107 (2010). doi: 10.1002/nme.2883
[3] Tamura, T. and Ono, Y., “LES Analysis on Aeroelastic Instability of Prisms in Turbulent Flow,” Journal of Wind Engineering and Industrial Aerodynamics, Vol. 91, No. 1215, pp. 18271846 (2003). doi: 10.1016/ j.jweia.2003.09.032
[4] Deng, J., Ren, A. L. and Zou, J. F., “Numerical Study of Transverse Galloping and Vortex-induced Vibrations of Square Cylinder,” Journal of Zhejiang University (Engineering Science), Vol. 39, No. 4, pp. 595 599 (2005). (in Chinese)
[5] Zhao, M., Cheng, L. and Zhou, T. M., “Numerical Simulation of Vortex-induced Vibration of a Square Cylinder at a Low Reynolds Number,” Physics of Fluids, Vol. 25, No. 2 (2013). doi: 10.1063/1.4792351
[6] Fang, P. Z. and Gu, M., “Numerical Simulation of Vortex-induced Vibration for a Square Cylinder at High Reynolds Number,” Journal of Tongji University (Natural Science), Vol. 36, No. 2, pp. 161165 (2008). (in Chinese)
[7] Hirt, C. W., Amsden, A. A. and Cook, J. L., “An Arbitrary Lagrangian-Eulerian Computing Method for All Flow Speeds,” Journal of Computational Physics, Vol. 135, pp. 203–216 (1997). doi: 10.1006/jcph.1997.5727
[8] Glück, M., “Computation of Wind-induced Vibrations of Flexible Shells and Membranous Structures,” Journal of Fluids and Structures, Vol. 17, No. 5, pp. 739 765 (2003). doi: 10.1016/S0889-9746(03)00006-9
[9] Scruton, C., “On the Wind Excited Oscillations of Stacks, Towers and Masts,” Proceedings of the International Conference on Wind Effects on Buildings and Structures, Her Majesty’s Stationary Office, Teddington, Vol. 298 (1963).
[10] Lyn, D. A., “Phase Averaged Turbulence Measurements in the Separated Shear Layer Region of Flow Around a Square Cylinder,” Proc 23rd Cong Int Ass Hydraulic Research, Ottawa Canada, pp. 8592 (1989).
[11] Bouris, D. and Bergeles, G., “2D LES of Vortex Shedding from a Square Cylinder,” Journal of Wind Engineering and Industrial Aerodynamics, Vol. 80, pp. 3146 (1999).
We use cookies on this website to personalize content to improve your user experience and analyze our traffic. By using this site you agree to its use of cookies.
