P. R. Shrama 1 and Gurminder Singh2
1Department of Mathematics, University of Rajasthan, Jaipur - 302055, India
2Birla Institute of Technology (Mesra, Ranchi) Ext. Centre Jaipur, 27, Malviya Industrial Area, Jaipur - 302017, India
Received:
December 16, 2008
Accepted:
September 12, 2009
Publication Date:
September 1, 2010
Download Citation:
||https://doi.org/10.6180/jase.2010.13.3.02
ABSTRACT
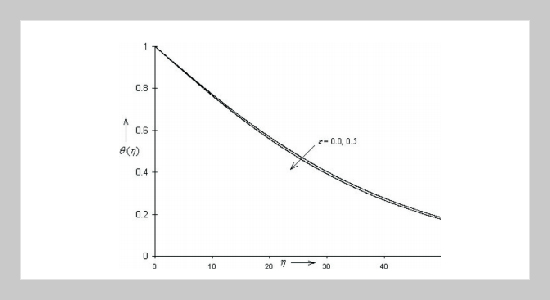
Aim of the paper is to investigate the effect of temperature dependent electrical conductivity on steady natural convection flow of a viscous incompressible low Prandtl (Pr <<1) electrically conducting fluid along an isothermal vertical non-conducting plate in the presence of transverse magnetic field and exponentially decaying heat generation. The governing equations of continuity, momentum and energy are transformed into ordinary differential equations using similarity transformation. The resulting coupled non-linear ordinary differential equations are solved using Runge-Kutta fourth order method alongwith shooting technique. The velocity and temperature distributions are discussed numerically and presented through graphs. The numerical values of skin-friction coefficient and Nusselt number at the plate are derived, discussed numerically for various values of physical parameters and presented through Tables.
Keywords:
Steady, MHD, Free Convection, Boundary Layer Flow, Variable Electrical Conductivity, Internal Heat Generation, Skin-Friction Coefficient, Nusselt Number
REFERENCES
- [1]Jaluria, Y., Natural Convection, Heat and Mass Transfer, Pergamon Press, New York (1980).
- [2]Michiyoshi, I.; Takahashi, I. and Serizawa, A., “Natural convection heat transfer from a horizontal cylinder to mercury under magnetic field”, International Journal of Heat and Mass Transfer, Vol. 19, pp. 1021-1029(1976).
- [3]Fumizawa, M., “Natural convection experiment with NaK under transverse magnetic field”, Journal of Nuclear Science and Technology, Vol. 17(2), pp. 98-105(1980).
- [4]Sahin, A.Z., “Transient heat conduction in semi-infinite solid with spatially decaying exponential heat generation”, International Communication in Heat and Mass Transfer, 19, pp.349-358 (1992).
- [5]Crepeau, J.C. and Clarksean, R., “Similarity solution of natural convection with internal heat generation”, ASME Journal of Heat Transfer, Vol. 119(1), pp. 183-185 (1997).
- [6]Ostrach, S., An analysis of laminar free convective flow and heat transfer about a flat plate parallel to direction of the generating body force, NACA Technical Report 1111, 1952.
- [7]Gebhart, B., “Effects of viscous dissipation in natural convection”, Journal of Fluid Mechanics, Vol. 14(2), pp.225-232 (1962).
- [8]Soundalgekar, V.M., “Effects of mass transfer on free convective flow of a dissipative, incompressible fluid past an infinite vertical porous plate with suction”, Indian Academy of Sciences, India, Vol. 84A, pp.194-203 (1976).
- [9]Joshi,Y. and Gebhart, B., “Effect of pressure stress work and viscous dissipation in some natural convection flow”, International Journal of Heat and Mass Transfer, Vol. 24, pp. 1577-1588 (1981).
- [10]Wantanabe, T. and Pop, I., “Thermal boundary layers in magnetohydrodynamic flow over a flat plate in the presence of a transverse magnetic field”, Acta Mechanica, 105(1-4), pp. 233-238(1994).
- [11]Chamkha, A.J. and Khaled, A.R.A., “Similarity solutions for hydromagnetic simultaneous heat and mass transfer by natural convection from an inclined plate with internal heat generation or absorption”, Heat and Mass Transfer, Vol. 37(2-3), pp.117-123 (2001).
- [12]Chen, C.H., “Heat and mass transfer in MHD flow by natural convection from a permeable, inclined surface with variable wall temperature and concentration”, Acta Mechanica, Vol. 172(3-4), pp.219-235(2004).
- [13]Molla, M.M.; Hossain, M.A. and Yao, L.S., “Natural convection flow along a vertical wavy surface with heat generation / absorption”, International Journal of Thermal Science, Vol. 43(2), pp. 157-163(2004).
- [14]Molla, M.M., Hossain, M.A. and Paul, M.C., “Natural convection flow from an isothermal cylinder in the presence of heat generation”, International Journal of Engineering Science, Vol. 44(13-14), pp. 949-958 (2006).
- [15]Jeffery, A., Magnetohydrodynamics, Oliver and Boyed, New York, USA (1966).
- [16]Bansal, J.L., Viscous Fluid Dynamics, Oxford & IBH Pub. Co., New Delhi, India (1977).
- [17]Bansal, J.L., Magnetofluiddynamics of Viscous Fluids, Jaipur Pub. House, Jaipur, India (1994).
- [18]Schlichting, H. and Gersten, K., Boundary Layer Theory, McGraw-Hill Book Co., New York, USA(1999).
- [19]Jain, M.K.; Iyengar, S.R. and Jain, R.K., Numerical Methods for Scientific and Engineering Computation, Wiley Eastern Ltd., New Delhi, India (1985).
- [20]Krishnamurthy, E.V. and Sen, S.K., Numerical Algorithms, Affiliated East-West Press Pvt. Ltd., New Delhi, India (1986).
- [21]Jain, M.K., Numerical Solution of Differential Equations, New Age Int. Pub. Co., New Delhi, India (2000).
- [22]Conte, S.D. and Boor, C., Elementary Numerical Analysis, McGraw-Hill Book Co., New York (1981).