N. Nithyadevi1, V. Divya 1 and M. Rajarathinam1
1Department of Mathematics, Bharathiar University, Coimbatore - 641 046, India
Received:
May 24, 2016
Accepted:
January 31, 2017
Publication Date:
June 1, 2017
Download Citation:
||https://doi.org/10.6180/jase.2017.20.2.05
ABSTRACT
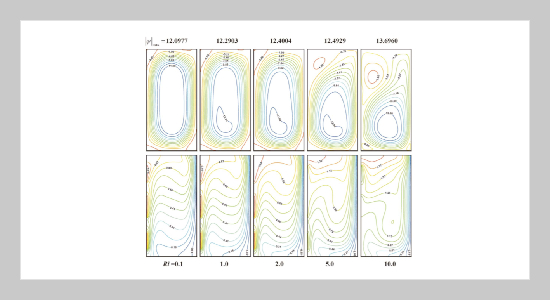
The present numerical study investigates the laminar natural convection heat transfer and the effect of Prandtl number in a two dimensional rectangular enclosure with discrete heaters. Four different cases are considered based on the number of discrete heaters which is maintained at isothermal condition Th (Th > Tc). The right vertical wall is maintained at cold temperature Tc and the remaining all other walls are thermally insulated. The above schematic setup can be modeled into mathematical form and the governing non-dimensional equations are solved using Finite Volume Method with power-law scheme. SIMPLE algorithm is employed for the pressure-velocity coupled momentum equations. Numerical simulations are carried out to find the effect of different Prandtl numbers (0.054, 0.71, 1.4 and 7.0), internal heat generation parameter Ri ranging from 0.1 to 10.0 and the distribution of discrete heaters. Results are given in the form of streamlines, isotherms, the velocity profiles and average Nusselt number. It is found that the maximum heat transfer rate is achieved for the distribution of discrete heater and also for increasing values of Prandtl number.
Keywords:
Discrete Heater, Natural Convection, Prandtl Number, SIMPLE Algorithm
REFERENCES
- [1] Ho, C. J. and Chang, J. Y., “A Study of Natural Convection Heat Transfer in a Vertical Rectangular Enclosure with Two-dimensional Discrete Heating: Effect of Aspect Ratio,” Int. J. Heat Mass Transfer, Vol. 37, No. 6, pp. 917925 (1994). doi: 10.1016/0017-9310(94) 90217-8
- [2] Aydin, O. and Pop, I., “Natural Convection from a Discrete Heater in Enclosures Filled with a Micropolar Fluid,” Int. J. of Engg. Science, Vol. 43, No. 1920, pp. 1409–1418 (2005). doi: 10.1016/j.ijengsci.2005.06. 005
- [3] Sivasankaran, S., “Buoyant Convection in a Cavity with Discrete Heat Sources and Internal Heat Generation,” Int. J. of Appl. Math. and Mech., Vol. 2, No. 2, pp. 6374 (2006).
- [4] Saha, G., Saha, S., Islam, M. Q. and Akhanda, M. A. R., “Natural Convection in Enclosure with Discrete Isothermal Heating from Below,” J. Naval Architecture and Marine Engg., Vol. 4, No. 1, pp. 113 (2007). doi: 10.3329/jname.v4i1.912
- [5] Nithyadevi, N., Kandaswamy, P. and Lee, J., “Natural Convection in a Rectangular Cavity with Partially Active Side Walls,” Int. J. of Heat and Mass Transfer, Vol. 50, No. 2324, pp. 46884697 (2007). doi: 10. 1016/j.ijheatmasstransfer.2007.03.050
- [6] Kandaswamy, P., Nithyadevi, N. and Ng, C. O., “Natural Convection in Enclosures with Partially Thermally Active Side Walls Containing Internal Heat Sources,” Phys. of Fluids, Vol. 20, pp. 097104097123 (2008). doi: 10.1063/1.2981834
- [7] Alam, P., Kumar, A., Kapoor, S. and Ansari, S. R., “Numerical Investigation of Natural Convection in a Rectangular Enclosure Due to Partial Heating and Cooling at Vertical Walls,” Commun. in Nonlinear Sci. Numer. Simulat., Vol. 17, No. 6, pp. 24032414 (2012). doi: 10.1016/j.cnsns.2011.09.004
- [8] Falahat, A., “Effect of Aspect Ratio on Laminar Natural Convection in Partially Heated Enclosure,” Universal J. Mech. Engg., Vol. 2, No. 1, pp. 2833 (2014). doi: 10.13189/ujme.2014.020104
- [9] Turan, O., Poole, R. J. and Chakraborty, N., “Influence of Boundary Conditions on Laminar Natural Convection in Rectangular Enclosures with Differentially Heated Side Walls,” Int. J. Heat and Fluid Flow, Vol. 33, No. 1, pp. 131146 (2012). doi: 10.1016/j. ijheatfluidflow.2011.10.009
- [10] Zaman, F. S., Turja, T. S. and Molla, Md. M., “Buoyancy Driven Natural Convection in an Enclosure with Two Discrete Heating from Below,” Procedia Engg., Vol. 56, pp. 104111 (2013). doi: 10.1016/j.proeng. 2013.03.095
- [11] Qarnia, H. E., Draoui, A. and Lakhal, E. K., “Computation of Melting with Natural Convection Inside a Rectangular Enclosure Heated by Discrete Protruding Heat Sources,” Appl. Math. Modelling, Vol. 37, No. 6, pp. 3968–3981 (2013). doi: 10.1016/j.apm.2012.08. 021
- [12] An, C., Vieira, C. B. and Su, J., “Integral Transform Solution of Natural Convection in a Square Cavity with Volumetric Heat Generation,” Braz. J. Chem. Eng., Vol. 30, No. 4, pp. 883896 (2013). doi: 10. 1590/S0104-66322013000400020
- [13] Ahmed, S. E., Mansour, M. A., Hussein, A. K. and Sivasankaran, S., “Mixed Convection from a Discrete Heat Source in Enclosures with Two Adjacent Moving Walls and Filled with Micropolar Nanofluids,” Int. J. Engg. Science and Technology, Vol. 19, No. 1, pp. 364–376 (2016). doi: 10.1016/j.jestch.2015.08.005
- [14] Purusothaman, A., Oztop, H. F., Nithyadevi, N. and Abu-Hamdeh, N. H., “3D Natural Convection in a Cubical Cavity with a Thermally Active Heater under the Presence of an External Magnetic Field,” Comp. & Fluids, Vol. 128, pp. 3040 (2016). doi: 10.1016/j. compfluid.2016.01.011
- [15] Graebel, W. P., “The Influence of Prandtl Number on Free Convection in a Rectangular Cavity,” Int. J. Heat and Mass Transfer, Vol. 24, No. 1, pp. 125131 (1981). doi: 10.1016/0017-9310(81)90100-9
- [16] Basak, T., Roy, S. and Balakrishnan, A. R., “Effects of Thermal Boundary Conditions on Natural Convection Flows within a Square Cavity,” Int. J. Heat and Mass Transfer, Vol. 49, No. 2324, pp. 45254535 (2006). doi: 10.1016/j.ijheatmasstransfer.2006.05.015
- [17] Rahman, M. M., Parvin, S., Rahim, N. A., Islam, M. R., Saidur, R. and Hasanuzzaman, M., “Effects of Reynolds and Prandtl Number on Mixed Convection in a Ventilated Cavity with a Heat-generating Solid Circular Block,” Appl. Math. Modelling, Vol. 36, No. 5, pp. 20562066 (2012). doi: 10.1016/j.apm.2011. 08.014
- [18] Urquiza, G., Castro, L., Garcia, J., Basurtio, M. and Bogarin, E., “Numerical Simulation on Mixed Convection in a Rotating Cylindrical Cavity: Influence of Prandtl Number,” Advances in Mech. Engg., Vol. 2013, pp. 95076659507673 (2013). doi: 10.1155/ 2013/950765
- [19] Jani, S., Mahmoodi, M. and Amini, M., “Natural Convection at Different Prandtl Numbers in Rectangular Cavities with a Fin on the Cold Wall,” J. Energy: Engg. and Management, Vol. 2, No. 4, pp. 5869 (2012).
- [20] Lee, J. R. and Park, I. S., “Numerical Analysis for Prandtl Number Depending on Natural Convection in an Enclosure Having an Vertical Thermal Gradient with a Square Insulator Inside,” Nuclear Engg. Tech., Vol. 44, No. 3, pp. 283296 (2012). doi: 10.5516/ NET.02.2011.027
- [21] Bouabid, M., Hidouri, N., Magherbi, M., Eljery, A. and Brahim, A. B., “Irreversibility Investigation on MHD Natural Convection in a Square Cavity for Different Prandtl Numbers,” World Science Research Journals, Vol. 2, No. 4, pp. 6075 (2014).
- [22] Patankar, S. V., Numerical Heat Transfer and Fluid Flow, Hemisphere/McGraw-Hill, Washington (1980).
- [23] Davis, D. V., “Natural Convection of Air in a Square Cavity; a Bench Mark Numerical Solution,” Int. J. Numer. Methods in Fluids, Vol. 3, No. 4, pp. 249264 (1983). doi: 10.1002/fld.1650030305
- [24] Mobedi, M., Ozkol, U. and Sunden, B., “Visualization of Diffusion and Convection Heat Transport in a Square Cavity with Natural Convection,” Int. J. Heat Mass Transfer, Vol. 53, No. 13, pp. 99109 (2010). doi: 10.1016/j.ijheatmasstransfer.2009.09.048